Video hướng dẫn giải
LG a
a) Khảo sát sự biến thiên và vẽ đồ thị (C) của hàm số y=x+3x+1.
Phương pháp giải:
Khảo sát và vẽ đồ thi qua các bước đã được học.
Lời giải chi tiết:
Xét hàm số: y=x+3x+1
Tập xác định : D=R∖{−1}
* Sự biến thiên:
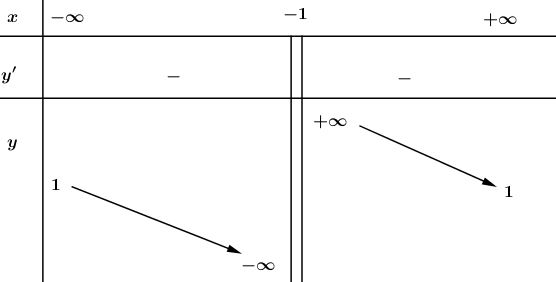
y′=−2(x+1)2<0,∀x∈D
- Hàm số nghịch biến trên khoảng: (−∞;−1) và (−1;+∞)
- Cực trị: Hàm số không có cực trị.
- Tiệm cận:
limx→−1−y=−∞limx→−1+y=+∞limx→±∞y=1
Tiệm cận đứng: x=−1.
Tiệm cận ngang: y=1.
Bảng biến thiên:
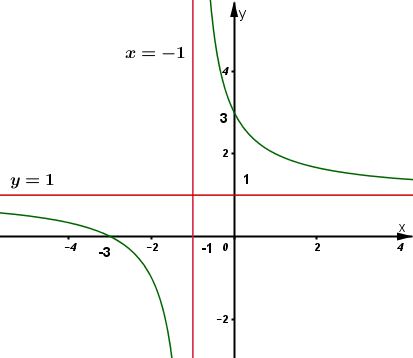
* Đồ thị:
Đồ thị hàm số giao Ox tại (−3;0), giao Oy tại (0;3)
Đồ thị hàm số nhận điểm I(−1;1) làm tâm đối xứng.
LG b
b) Chứng minh rằng với mọi giá trị của m, đường thẳng y=2x+m luôn cắt (C) tại hai điểm phân biệt M và N.
Phương pháp giải:
Chứng minh phương trình hoành độ giao điểm của hai đồ thị hàm số có hai nghiệm phân biệt khác −1 với mọi m.
Lời giải chi tiết:
Xét phương trình có nghiệm là hoành độ giao điểm của (C) và đường thẳng (d): y=2x+m (1)
x+3x+1=2x+m⇔x+3=(2x+m)(x+1)⇔2x2+(m+1)x+m−3=0,x≠−1
\displaystyle Δ = (m+1)^2– 4.2(m-3) \\ = m^2+2m+1-8m+24 \\= m^2– 6m + 25\\ = (m-3)^2+ 16> 0
\displaystyle \Rightarrow (1) luôn có hai nghiệm phân biệt.
Lại có: \displaystyle f\left( { - 1} \right) = 2.{\left( { - 1} \right)^2} - \left( {m + 1} \right) + m - 3 = - 2 \ne 0 hay phương trình (1) có nghiệm khác \displaystyle -1.
Vậy phương trình (1) luôn có 2 nghiệm phân biệt khác \displaystyle -1 với mọi \displaystyle m.
Vậy (d) luôn cắt (C) tại hai điểm phân biệt \displaystyle M, N (hoành độ của \displaystyle M, N chính là nghiệm của (1)).
LG c
c) Xác định m sao cho độ dài \displaystyle MN là nhỏ nhất.
Phương pháp giải:
Với hai điểm \displaystyle M và \displaystyle N tìm được ở câu trên, tính độ dài đoạn thẳng \displaystyle MN theo công thức: \displaystyle MN = \sqrt {{{\left( {{x_N} - {x_M}} \right)}^2} + {{\left( {{y_N} - {y_M}} \right)}^2}} = \sqrt {f\left( x \right)} .
+) Khảo sát và tìm giá trị nhỏ nhất của hàm số \displaystyle y=f(x) từ đó suy ra độ dài nhỏ nhất của \displaystyle MN.
Lời giải chi tiết:
Gọi \displaystyle M\left( {{x_M};\;{y_M}} \right) và \displaystyle N\left( {{x_N};\;{y_N}} \right) là hai giao điểm của \displaystyle (C) và đường tahnwgr \displaystyle y=2x+m.
Theo định lí Vi-et ta có:\displaystyle \left\{ \matrix{{x_M} + {x_N} = - {{m + 1} \over 2} \hfill \cr {x_M}.{x_N} = {{m - 3} \over 2} \hfill \cr} \right.
\displaystyle \begin{array}{l} M{N^2} = {\left( {{x_M} - {x_N}} \right)^2} + {\left( {{y_M} - {y_N}} \right)^2}\\ = {\left( {{x_M} - {x_N}} \right)^2} + {\left[ {2{x_M} + m - \left( {2{x_N} + m} \right)} \right]^2}\\ = {\left( {{x_M} - {x_N}} \right)^2} + 4{\left( {{x_M} - {x_N}} \right)^2}\\ = 5{\left( {{x_M} - {x_N}} \right)^2}\\ = 5\left[ {{{\left( {{x_M} + {x_N}} \right)}^2} - 4{x_M}{x_N}} \right]\\ = 5\left[ {{{\left( { - \frac{{m + 1}}{2}} \right)}^2} - 4.\frac{{m - 3}}{2}} \right]\\ = 5\left( {\frac{{{m^2} + 2m + 1}}{4} - 2m + 6} \right)\\ = 5.\frac{{{m^2} - 6m + 25}}{4} \\ = \frac{5}{4}\left[ {\left( {{m^2} - 6m + 9} \right) + 16} \right]\\ = \frac{5}{4}\left[ {{{\left( {m - 3} \right)}^2} + 16} \right]. \end{array}
Ta có: \displaystyle {\left( {m - 3} \right)^2} \ge 0\;\forall \;m \Rightarrow {\left( {m - 3} \right)^2} + 16 \ge 16
\displaystyle \begin{array}{l} \Rightarrow M{N^2} \ge \frac{5}{4}.16 = 20.\\ \Rightarrow MN \ge 2\sqrt 5 . \end{array}
Dấu "=" xảy ra \displaystyle \Leftrightarrow m - 3 = 0 \Leftrightarrow m = 3.
Vậy độ dài \displaystyle MN nhỏ nhất bằng \displaystyle 2\sqrt5 khi \displaystyle m=3.
LG d
d) Tiếp tuyến tại một điểm \displaystyle S bất kì của \displaystyle (C) luôn cắt hai tiệm cận của \displaystyle (C) tại \displaystyle P và \displaystyle Q. Chứng minh rằng \displaystyle S là trung điểm của \displaystyle PQ.
Phương pháp giải:
Gọi \displaystyle S(x_0;\, y_0) là 1 điểm bất kì thuộc đồ thị hàm số \displaystyle (C). Khi đó phương trình tiếp tuyến của đồ thị hàm số tại \displaystyle S là: \displaystyle \Delta: \, y = y'\left( {{x_0}} \right)\left( {x - {x_0}} \right) + {y_0}.
+) Tìm các giao điểm \displaystyle P, \, \, Q của tiếp tuyến \displaystyle \Delta với các đường tiệm cận.
+) Khi đó \displaystyle S là trung điểm của \displaystyle PQ\Leftrightarrow \left\{ \begin{array}{l}{x_0} = \frac{{{x_P} + {x_Q}}}{2}\\{y_0} = \frac{{{y_P} + {y_Q}}}{2}\end{array} \right..
Lời giải chi tiết:
Giả sử \displaystyle S(x_0;y_0) là điểm bất kì thuộc (C)
Phương trình tiếp tuyến \displaystyle Δ của (C) tại \displaystyle S là:
\displaystyle \eqalign{ & y - y_0 = y'({x_0})(x - {x_0}) \cr & \Leftrightarrow y = {{ - 2} \over {{{({x_0} + 1)}^2}}}(x - {x_0}) + {{{x_0} + 3} \over {{x_0} + 1}} \cr}
Tiệm cận đứng: \displaystyle x=-1 và tiệm cận ngang: \displaystyle y=1.
Giả sử \displaystyle Δ cắt tiệm cận ngang tại \displaystyle P(x_P; 1). Khi đó:
\displaystyle \begin{array}{l} \frac{{ - 2}}{{{{\left( {{x_0} + 1} \right)}^2}}}\left( {{x_P} - {x_0}} \right) + \frac{{{x_0} + 3}}{{{x_0} + 1}} = 1\\ \Leftrightarrow - 2{x_P} + 2{x_0} + x_0^2 + 4{x_0} + 3 = x_0^2 + 2{x_0} + 1\\ \Leftrightarrow - 2{x_P} = - 4{x_0} - 2\\ \Leftrightarrow {x_P} = 2{x_0} + 1\\ \Rightarrow P\left( {2{x_0} + 1;\;1} \right). \end{array}
\displaystyle Δ cắt tiệm cận đứng tại \displaystyle Q( - 1; y_Q). Khi đó:
\displaystyle \begin{array}{l} \frac{{ - 2}}{{{{\left( {{x_0} + 1} \right)}^2}}}\left( { - 1 - {x_0}} \right) + \frac{{{x_0} + 3}}{{{x_0} + 1}} = {y_Q}\\ \Leftrightarrow 2 + 2{x_0} + x_0^2 + 4{x_0} + 3 = {y_Q}{\left( {{x_0} + 1} \right)^2}\\ \Leftrightarrow x_0^2 + 6{x_0} + 5 = {y_Q}{\left( {{x_0} + 1} \right)^2}\\ \Leftrightarrow \left( {{x_0} + 1} \right)\left( {{x_0} + 5} \right) = {y_Q}{\left( {{x_0} + 1} \right)^2}\\ \Leftrightarrow {y_Q} = \frac{{{x_0} + 5}}{{{x_0} + 1}}.\\ \Rightarrow Q\left( { - 1;\;\frac{{{x_0} + 5}}{{{x_0} + 1}}} \right) \end{array}
\displaystyle \Rightarrow \left\{ \begin{array}{l} {x_P} + {x_Q} = 2{x_0} + 1 - 1 = 2{x_0} = 2{x_S}\\ {y_P} + {y_Q} = 1 + \frac{{{x_0} + 5}}{{{x_0} + 1}} = \frac{{2{x_0} + 6}}{{{x_0} + 1}} = \frac{{2\left( {{x_0} + 3} \right)}}{{{x_0} + 1}} = 2{y_0} = 2{y_S}. \end{array} \right.
Vậy \displaystyle S là trung điểm của \displaystyle PQ.