Đề bài
Cho tứ giác ABCD. Gọi M,N,P,Q lần lượt là trung điểm của các đoạn AC,BD,AD và có MN=PQ. Chứng minh rằng AB⊥CD.
Phương pháp giải - Xem chi tiết
Ta cần chứng minh →AB.→CD=0
Lời giải chi tiết
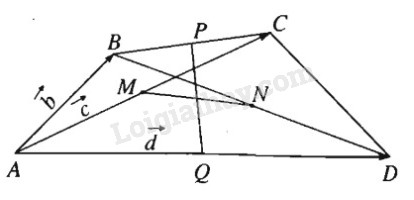
Ta cần chứng minh →AB.→CD=0
Đặt →AB=→b,→AC=→c,→AD=→d. Ta có:
→MN=→MA+→AN =−12→AC+12(→AB+→AD)
Suy ra →MN=12(→b+→d−→c)
→QP=→QA+→AP=−12→AD+12(→AB+→AC)=12(→b+→c−→d)
Theo giả thiết ta có:
MN=PQ⇔→MN2=→QP2
(→b+→d−→c)2=(→b+→c−→d)2⇔→b.→d−→b.→c=→b.→c−→b.→d⇔2→b.→d−2→b.→c=0⇔→b.(→d−→c)=0⇔→AB.(→AD−→AC)=0⇔→AB.→CD=0⇔→AB⊥→CD

