Trong các đẳng thức sau đây, đẳng thức nào đúng ?
Vì \(\alpha = {150^0}\) là góc tù nên \(\sin \alpha > 0,\cos \alpha < 0,\tan \alpha = \dfrac{{\sin \alpha }}{{c{\rm{os}}\alpha }} < 0,\cot \alpha < 0.\)
Do đó các đáp án A, B, D đều sai. Ta chỉ xét đáp án C. Ta có \(\tan {150^ \circ } = - \tan {30^ \circ } = - \dfrac{1}{{\sqrt 3 }}.\)
Cho hình bình hành ABCD. M là điểm bất kì, khi đó:
Đáp án A ta có:
\(\left\{ \begin{array}{l}\overrightarrow {MC} - \overrightarrow {MA} = \overrightarrow {AC} \\\overrightarrow {MB} - \overrightarrow {MD} = \overrightarrow {DB} \\\overrightarrow {AC} \ne \overrightarrow {DB} \end{array} \right. \) \(\Rightarrow \overrightarrow {MC} - \overrightarrow {MA} \ne \overrightarrow {MB} - \overrightarrow {MD} \)
\(\Rightarrow \) đáp án A sai.
Đáp án B ta có:
\(\left\{ \begin{array}{l}\overrightarrow {MC} - \overrightarrow {MA} = \overrightarrow {AC} \\\overrightarrow {DA} - \overrightarrow {DC} = \overrightarrow {CA} \\\overrightarrow {AC} \ne \overrightarrow {CA} \end{array} \right. \) \(\Rightarrow \overrightarrow {MC} - \overrightarrow {MA} \ne \overrightarrow {DA} - \overrightarrow {DC} \)
\(\Rightarrow \) B sai.
Đáp án C ta có:
\(\left\{ \begin{array}{l}\overrightarrow {MC} - \overrightarrow {MA} = \overrightarrow {AC} \\\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC} \end{array} \right.\) \(\Rightarrow \overrightarrow {MC} - \overrightarrow {MA} = \overrightarrow {AB} + \overrightarrow {AD} \)
\(\Rightarrow \) C đúng.
Cho tứ giác ABCD có \(\overrightarrow {AD} = \overrightarrow {BC} \). Mệnh đề nào trong các mệnh đề sau là sai?
Dựa vào hình vẽ ta thấy ABCD là hình bình hành nên A đúng.
\( \Rightarrow AD = BC \Rightarrow \) B đúng.
Hai vectơ \(\overrightarrow {AB} \) và \(\overrightarrow {DC} \) cùng hướng và AB = DC nên \(\overrightarrow {AB} = \overrightarrow {DC} \Rightarrow D\) đúng.
Vậy C sai. (2 đường chéo của hình bình hành không bằng nhau)
Cho ba điểm A, B, C phân biệt. Điều kiện cần và đủ để ba điểm A, B, C thẳng hàng là:
Điều kiện cần và đủ để ba điểm A, B, C thẳng hàng là 2 vectơ \(\overrightarrow {AB} \) và \(\overrightarrow {AC} \) cùng phương
\( \Leftrightarrow \exists k \ne 0:\overrightarrow {AB} = k\overrightarrow {AC} \).
Cho tam giác ABC, có BC = a, CA = b, AB = c. Mệnh đề nào sau đây đúng?
Nếu \(\cos A > 0\) thì góc A nhọn hay \({b^2} + {c^2} - {a^2} > 0\) thì góc A nhọn.
Cho hai vectơ \(\overrightarrow a = \left( {4;3} \right),\overrightarrow b = \left( {1;7} \right)\). Góc giữa hai vectơ \(\overrightarrow a \) và \(\overrightarrow b \) là?
\(\cos \left( {\overrightarrow a ;\overrightarrow b } \right) = \dfrac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}}\) \( = \dfrac{{4.1 + 3.7}}{{\sqrt {{4^2} + {3^2}} .\sqrt {{1^2} + {7^2}} }} = \dfrac{{25}}{{\sqrt {25} .\sqrt {50} }} \) \(= \dfrac{{\sqrt 2 }}{2}\)
Do đó góc giữa hai véc tơ bằng \(45^0\).
Cho hai tam giác ABC và A’B’C’ lần lượt có trọng tâm G và G’. Đẳng thức nào dưới đây là sai?
Vì G và G’ lần lượt là trọng tâm của tam giác ABC và A’B’C’ nên ta có:
\(\begin{array}{l}\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} = \overrightarrow 0 \\\overrightarrow {G'A'} + \overrightarrow {G'B'} + \overrightarrow {G'C'} = \overrightarrow 0 .\end{array}\)
Với điểm M bất kì khác điểm G ta chứng minh: \(3\overrightarrow {MG} = \overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} \)
Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} \) \(= \overrightarrow {MG} + \overrightarrow {GA} + \overrightarrow {MG} + \overrightarrow {GB} + \overrightarrow {MG} + \overrightarrow {GC} = 3\overrightarrow {MG} \)
Tương tự ta có: \(3\overrightarrow {MG'} = \overrightarrow {MA'} + \overrightarrow {MB'} + \overrightarrow {MC'} \)
Từ đó suy ra
\(\begin{array}{l}3\overrightarrow {GG'} = 3\left( {\overrightarrow {MG'} - \overrightarrow {MG} } \right) = 3\overrightarrow {MG'} - 3\overrightarrow {MG} \\ = \overrightarrow {MA'} + \overrightarrow {MB'} + \overrightarrow {MC'} - \overrightarrow {MA} - \overrightarrow {MB} - \overrightarrow {MC} \\ = \left( {\overrightarrow {MA'} - \overrightarrow {MA} } \right) + \left( {\overrightarrow {MB'} - \overrightarrow {MB} } \right) + \left( {\overrightarrow {MC'} - \overrightarrow {MC} } \right)\\ = \overrightarrow {AA'} + \overrightarrow {BB'} + \overrightarrow {CC'} .\end{array}\)
Nên A đúng.
Đáp án B:
\(\begin{array}{l}3\overrightarrow {GG'} = \overrightarrow {GG'} + \overrightarrow {GG'} + \overrightarrow {GG'} \\ = \overrightarrow {GA} + \overrightarrow {AC'} + \overrightarrow {C'G'} + \overrightarrow {GB} + \overrightarrow {BA'} + \overrightarrow {A'G'} \\ + \overrightarrow {GC} + \overrightarrow {CB'} + \overrightarrow {B'G'} \\ = \left( {\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} } \right) + \left( {\overrightarrow {AC'} + \overrightarrow {BA'} + \overrightarrow {CB'} } \right)\\ + \left( {\overrightarrow {C'G'} + \overrightarrow {A'G'} + \overrightarrow {B'G'} } \right)\\ = \overrightarrow 0 + \left( {\overrightarrow {AC'} + \overrightarrow {BA'} + \overrightarrow {CB'} } \right) + \overrightarrow 0 \\ = \overrightarrow {AC'} + \overrightarrow {BA'} + \overrightarrow {CB'} \end{array}\)
Nên B đúng.
Đáp án C:
\(\begin{array}{l}3\overrightarrow {GG'} = \overrightarrow {GG'} + \overrightarrow {GG'} + \overrightarrow {GG'} \\ = \overrightarrow {GA} + \overrightarrow {AB'} + \overrightarrow {B'G'} + \overrightarrow {GB} + \overrightarrow {BC'} + \overrightarrow {C'G'} \\ + \overrightarrow {GC} + \overrightarrow {CA'} + \overrightarrow {A'G'} \\ = \left( {\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} } \right) + \left( {\overrightarrow {AB'} + \overrightarrow {BC'} + \overrightarrow {CA'} } \right)\\ + \left( {\overrightarrow {B'G'} + \overrightarrow {C'G'} + \overrightarrow {A'G'} } \right)\\ = \overrightarrow 0 + \left( {\overrightarrow {AB'} + \overrightarrow {BC'} + \overrightarrow {CA'} } \right) + \overrightarrow 0 \\ = \overrightarrow {AB'} + \overrightarrow {BC'} + \overrightarrow {CA'} \end{array}\)
Nên C đúng.
D sai do A đúng.
Tam giác ABC vuông cân tại A có AB = AC = a. Đường trung tuyến BM có độ dài là?
\(B{M^2} = \dfrac{{B{A^2} + B{C^2}}}{2} - \dfrac{{A{C^2}}}{4} = \dfrac{{{a^2} + 2{a^2}}}{2} - \dfrac{{{a^2}}}{4} = \dfrac{{5{a^2}}}{4} \Rightarrow BM = \dfrac{{a\sqrt 5 }}{2}.\)
Trong mặt phẳng $Oxy$ cho ba điểm \(A\left( { - 1;1} \right),{\rm{ }}B\left( {2;4} \right),{\rm{ }}C\left( {6;0} \right)\). Khi đó tam giác $ABC$ là tam giác
Ta có:
\(\overrightarrow {AB} = \left( {3;3} \right),\overrightarrow {AC} = \left( {7; - 1} \right),\overrightarrow {BC} = \left( {4; - 4} \right)\)
Do \(\overrightarrow {AB} .\overrightarrow {BC} = 3.4 + 3.\left( { - 4} \right) = 0\) nên \(AB \bot BC\) hay tam giác \(ABC\) vuông tại \(B\)
Cho tam giác ABC và I thỏa mãn \(\overrightarrow {IA} = 3\overrightarrow {IB} \). Đẳng thức nào sau đây là đẳng thức đúng.
Ta có:
\(\begin{array}{l}\overrightarrow {CI} = \overrightarrow {CA} - \overrightarrow {IA} = \overrightarrow {CA} - 3\overrightarrow {IB} = \overrightarrow {CA} - 3\left( {\overrightarrow {CB} - \overrightarrow {CI} } \right)\\ \Leftrightarrow \overrightarrow {CI} = \overrightarrow {CA} - 3\overrightarrow {CB} + 3\overrightarrow {CI} \\ \Leftrightarrow 2\overrightarrow {CI} = 3\overrightarrow {CB} - \overrightarrow {CA} \\ \Leftrightarrow \overrightarrow {CI} = \dfrac{1}{2}\left( {3\overrightarrow {CB} - \overrightarrow {CA} } \right)\end{array}\)
Cho tam giác $ABC$ đều cạnh bằng $1$. Tính \(\overrightarrow {AB} .\overrightarrow {BC} \) ?
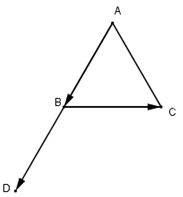
Lấy điểm $D$ sao cho \(\overrightarrow {AB} = \overrightarrow {BD} \Rightarrow \left( {\overrightarrow {AB} ,\overrightarrow {BC} } \right) = \left( {\overrightarrow {BD} ;\overrightarrow {BC} } \right) = {120^0}\)
\( \Rightarrow \overrightarrow {AB} .\overrightarrow {BC} = \left| {\overrightarrow {AB} } \right|.\left| {\overrightarrow {BC} } \right|.\cos \left( {\overrightarrow {AB} ,\overrightarrow {BC} } \right) = 1.1.\cos {120^0} = - \dfrac{1}{2}\)
(vì tam giác $ABC$ đều cạnh $1$ nên \(AB = BC = 1 \Rightarrow \left| {\overrightarrow {AB} } \right| = \left| {\overrightarrow {BC} } \right| = 1\))
Cho hình thang vuông \(ABCD\) có đáy lớn \(AB = 4a\), đáy nhỏ \(CD = 2a\), đường cao \(AD = 3a\); \(I\) là trung điểm của \(AD\) . Khi đó \(\left( {\overrightarrow {IA} + \overrightarrow {IB} } \right).\overrightarrow {ID} \) bằng:
Ta có \(\left( {\overrightarrow {IA} + \overrightarrow {IB} } \right).\overrightarrow {ID} = \left( {\overrightarrow {IA} + \overrightarrow {IA} + \overrightarrow {AB} } \right).\overrightarrow {ID} = 2\overrightarrow {IA} .\overrightarrow {ID} = - \dfrac{{9{a^2}}}{2}\) nên chọn B.
Trong mặt phẳng\(Oxy\) cho \(A\left( { - 1;1} \right)\), \(B\left( {1;3} \right)\), \(C\left( {1; - 1} \right)\). Khẳng định nào sau đây đúng.
Phương án A: do \(\overrightarrow {AB} = \left( {2;2} \right)\) nên loại A.
Phương án B: \(\overrightarrow {AB} = \left( {2;2} \right)\), \(\overrightarrow {BC} = \left( {0; - 4} \right)\), \(\overrightarrow {AB} .\overrightarrow {BC} = - 8\) suy ra \(\overrightarrow {AB} \) không vuông góc \(\overrightarrow {BC} \) nên loại B.
Phương án C: Ta có \(\overrightarrow {AB} = \left( {2;2} \right)\), \(\overrightarrow {AC} = \left( {2; - 2} \right)\), \(\overrightarrow {BC} = \left( {0; - 4} \right)\), suy ra \(AB = AC = \sqrt 8 \), \(\overrightarrow {AB} .\overrightarrow {AC} = 0\).
Nên tam giác \(ABC\) vuông cân tại \(A\). Do đó chọn C.
Cho $2$ vectơ \(\overrightarrow a \) và \(\overrightarrow b \)có độ dài bằng \(1\) thỏa\(\left| {\overrightarrow a + \overrightarrow b } \right| = 2\). Hãy xác định \(\left( {3\overrightarrow a - 4\overrightarrow b } \right)\left( {2\overrightarrow a + 5\overrightarrow b } \right)\)
\(\left| {\overrightarrow a } \right| = \left| {\overrightarrow b } \right| = 1\),\(\left| {\overrightarrow a + \overrightarrow b } \right| = 2 \Leftrightarrow {\left( {\overrightarrow a + \overrightarrow b } \right)^2} = 4 \Leftrightarrow \overrightarrow a .\overrightarrow b = 1\), \(\left( {3\overrightarrow a - 4\overrightarrow b } \right)\left( {2\overrightarrow a + 5\overrightarrow b } \right) = 6{\overrightarrow a ^2} - 20{\overrightarrow b ^2} + 7\overrightarrow a .\overrightarrow b = - 7\)
Cho 2 vectơ \(\overrightarrow a \) và \(\overrightarrow b \) có \(\left| {\overrightarrow a } \right| = 4\), \(\left| {\overrightarrow b } \right| = 5\) và \(\left( {\overrightarrow a ,\overrightarrow b } \right) = {120^{\rm{o}}}\). Tính \(\left| {\overrightarrow a + \overrightarrow b } \right|\)
Ta có \(\left| {\overrightarrow a + \overrightarrow b } \right| = \sqrt {{{\left( {\overrightarrow a + \overrightarrow b } \right)}^2}} = \sqrt {{{\overrightarrow a }^2} + {{\overrightarrow b }^2} + 2\overrightarrow a .\overrightarrow b } \)\( = \sqrt {{{\left| {\overrightarrow a } \right|}^2} + {{\left| {\overrightarrow b } \right|}^2} + 2\left| {\overrightarrow a } \right|\left| {\overrightarrow b } \right|\;\cos \left( {\overrightarrow a ,\overrightarrow b } \right)} = \sqrt {21} \)
Hai chiếc tàu thuỷ cùng xuất phát từ vị trí \(A\), đi thẳng theo hai hướng tạo với nhau một góc \({60^0}\). Tàu thứ nhất chạy với tốc độ \(30\,km/h\), tàu thứ hai chạy với tốc độ \(40\,km/h\). Hỏi sau \(2\) giờ hai tàu cách nhau bao nhiêu \(km\)?
Ta có: Sau \(2h\) quãng đường tàu thứ nhất chạy được là: \({S_1} = 30.2 = 60\,km.\)
Sau \(2h\) quãng đường tàu thứ hai chạy được là: \({S_2} = 40.2 = 80\,km.\)
Vậy: sau \(2h\) hai tàu cách nhau là: \(S = \sqrt {{S_1}^2 + {S_2}^2 - 2{S_1}.{S_2}.\cos {{60}^0}} = 20\sqrt {13} .\)
Cho tam giác ABC biết $A\left( { - 1;\,\,2} \right),\,\,B\left( {2;\,\,0} \right),\,\,C\left( { - 3;\,\,1} \right)$. Tìm tọa độ điểm M thuộc BC sao cho ${S_{ABM}} = \dfrac{1}{3}{S_{ABC}}$.
Giả sử M(x; y) là điểm thỏa mãn điều kiện đề bài.
Kẻ AH vuông góc với BC. Suy ra
$\begin{array}{l}\dfrac{1}{2}BM.AH = \dfrac{1}{3}.\dfrac{1}{2}AH.BC \Rightarrow \overrightarrow {BM} = \dfrac{1}{3}\overrightarrow {BC} \\\left( {x - 2;y} \right) = \dfrac{1}{3}\left( { - 5;1} \right) \Leftrightarrow \left\{ \begin{array}{l}x - 2 = - \dfrac{5}{3}\\y = \dfrac{1}{3}\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x = \dfrac{1}{3}\\y = \dfrac{1}{3}\end{array} \right. \Rightarrow M\left( {\dfrac{1}{3};\dfrac{1}{3}} \right).\end{array}$
Cho hình vuông ABCD cạnh a, tâm O. Gọi M là điểm tùy ý trên đường tròn nội tiếp hình vuông. Tính $\overrightarrow {MA} .\overrightarrow {MB} + \overrightarrow {MC} .\overrightarrow {MD} $.
Ta có
$\begin{array}{l}\overrightarrow {MA} .\overrightarrow {MB} + \overrightarrow {MC} .\overrightarrow {MD} = \left( {\overrightarrow {MO} + \overrightarrow {OA} } \right)\left( {\overrightarrow {MO} + \overrightarrow {OB} } \right) + \left( {\overrightarrow {MO} + \overrightarrow {OC} } \right)\left( {\overrightarrow {MO} + \overrightarrow {OD} } \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2M{O^2} + \overrightarrow {OA} .\overrightarrow {OB} + \overrightarrow {OC.} \overrightarrow {OD} + \overrightarrow {MO} \left( {\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} } \right).\end{array}$
Có \(\overrightarrow {OA} + \overrightarrow {OC} = \overrightarrow 0 ;\overrightarrow {OB} + \overrightarrow {OD} = \overrightarrow 0 \Rightarrow \overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow 0 \)
\(\overrightarrow {OA} \bot \overrightarrow {OB} \Rightarrow \overrightarrow {OA} .\overrightarrow {OB} = 0,\overrightarrow {OC} \bot \overrightarrow {OD} \Rightarrow \overrightarrow {OC} .\overrightarrow {OD} = 0\)
Đường tròn nội tiếp hình vuông cạnh a có bán kính \(\dfrac{a}{2} \Rightarrow MO = \dfrac{a}{2} \Rightarrow M{O^2} = \dfrac{{{a^2}}}{4}.\)
Vậy $\overrightarrow {MA} .\overrightarrow {MB} + \overrightarrow {MC} .\overrightarrow {MD} = 2.\dfrac{{{a^2}}}{4} = \dfrac{{{a^2}}}{2}$
Cho ba vector $\overrightarrow a ,\,\,\overrightarrow b ,\,\,\overrightarrow c $ thỏa mãn $\left| {\overrightarrow a } \right| = a,\,\,\left| {\overrightarrow b } \right| = b,\,\,\left| {\overrightarrow c } \right| = c$ và $\overrightarrow a + \overrightarrow b + 3\overrightarrow c = \overrightarrow 0 $. Tính $A = \overrightarrow a .\overrightarrow b + \overrightarrow b .\overrightarrow c + \overrightarrow c .\overrightarrow a $
Ta có $\overrightarrow a + \overrightarrow b + 3\overrightarrow c = \overrightarrow 0 $ $ \Leftrightarrow \overrightarrow a + \overrightarrow b + \overrightarrow c = - 2\overrightarrow c $ $ \Leftrightarrow {\left( {\overrightarrow a + \overrightarrow b + \overrightarrow c } \right)^2} = 4{\overrightarrow c ^2} $ $\Leftrightarrow {a^2} + {b^2} + {c^2} + 2A = 4{c^2}$
Do đó $A = \dfrac{1}{2}\left( {3{c^2} - {a^2} - {b^2}} \right)$.
Cho tam giác $ABC$ với tọa độ các đỉnh $A\left( {1;\,\, - 3} \right),\,\,B\left( {3;\,\, - 5} \right),\,\,C\left( {2;\,\, - 2} \right)$. Tìm tọa độ giao điểm $E$ của BC với phân giác trong của góc A.
Ta có $A{B^2} = 8,\,\,A{C^2} = 2 \Rightarrow \dfrac{{AC}}{{AB}} = \dfrac{1}{2}$
Giả sử E (x; y) thuộc đoạn BC. Theo tính chất đường phân giác ta có:
\(\begin{array}{l}\dfrac{{EB}}{{AB}} = \dfrac{{EC}}{{AC}} \Rightarrow \dfrac{{EC}}{{EB}} = \dfrac{{AC}}{{AB}} = \dfrac{1}{2}.\\ \Rightarrow \dfrac{{\overrightarrow {EC} }}{{\overrightarrow {EB} }} = - \dfrac{1}{2}\\ \Leftrightarrow \left( {2 - x;\,\, - 2 - y} \right) = - \dfrac{1}{2}\left( {3 - x;\,\, - 5 - y} \right)\\ \Leftrightarrow \left\{ \begin{array}{l}2 - x = - \dfrac{3}{2} + \dfrac{1}{2}x\\ - 2 - y = \dfrac{5}{2} + \dfrac{1}{2}y\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x = \dfrac{7}{3}\\y = - 3\end{array} \right.\\ \Rightarrow E\left( {\dfrac{7}{3}; - 3} \right)\end{array}\)
