Hiệu của hai véc tơ

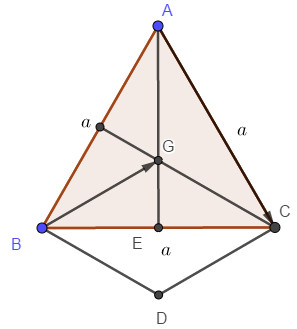
Ta có G là trọng tâm tam giác ABC nên \(GB \bot AC\) và \(GB = \dfrac{{a\sqrt 3 }}{3}\).

Vẽ hình bình hành \(BGCD\). Khi đó \(\overrightarrow {BG}  = \overrightarrow {DC} \)\( \Rightarrow CD = \dfrac{{a\sqrt 3 }}{3}\)

\(\overrightarrow {AC}  - \overrightarrow {BG}  = \overrightarrow {AC}  + \overrightarrow {CD}  = \overrightarrow {AD} \)\( \Rightarrow \left| {\overrightarrow {AC}  - \overrightarrow {BG} } \right| = AD\)

Vì \(GB \bot AC\) nên \(AC \bot CD\). Suy ra \(AD = \sqrt {{a^2} + \dfrac{{{a^2}}}{3}}  = \dfrac{{2a\sqrt 3 }}{3}\).

Vậy \(\left| {\overrightarrow {AC}  - \overrightarrow {BG} } \right| = \dfrac{{2a\sqrt 3 }}{3}\).

N là trung điểm của AC nên \(\overrightarrow {MA}  + \overrightarrow {MC}  = 2\overrightarrow {MN} \).

Ta có:

\(\begin{array}{l}\overrightarrow {MA}  - \dfrac{1}{2}\overrightarrow {AB}  + \overrightarrow {MC}  = \overrightarrow 0 \\ \Leftrightarrow \overrightarrow {MA}  + \overrightarrow {MC}  = \dfrac{1}{2}\overrightarrow {AB} \\ \Leftrightarrow \overrightarrow {MN}  = \dfrac{1}{4}\overrightarrow {AB}  = \overrightarrow {AE} \end{array}\)

Khi đó M là điểm thỏa mãn AENM là hình bình hành.

Xét các đáp án:

Đáp án A. Ta có \(\overrightarrow {CA}  - \overrightarrow {BA}  = \overrightarrow {CA}  + \overrightarrow {AB}  = \overrightarrow {CB}  =  - \overrightarrow {BC} \).Vậy A sai.

Đáp án B. Ta có \(\overrightarrow {AB}  + \overrightarrow {AC}  = \overrightarrow {AD}  \ne \overrightarrow {BC} \) (với \(D\) là điểm thỏa mãn \(ABDC\) là hình bình hành). Vậy B sai.

Đáp án C. Ta có \(\overrightarrow {AB}  + \overrightarrow {CA}  = \overrightarrow {CA}  + \overrightarrow {AB}  = \overrightarrow {CB} \). Vậy C đúng.

Xét các đáp án:

Đáp án A. Ta có \(\overrightarrow {OA}  - \overrightarrow {OB}  = \overrightarrow {BA}  = \overrightarrow {CD} \). Vậy A đúng.

Đáp án B. Ta có \(\left\{ \begin{array}{l}\overrightarrow {OB}  - \overrightarrow {OC}  = \overrightarrow {CB}  =  - \overrightarrow {AD} \\\overrightarrow {OD}  - \overrightarrow {OA}  = \overrightarrow {AD} \end{array} \right.\). Vậy B sai.

Đáp án C. Ta có \(\overrightarrow {AB}  - \overrightarrow {AD}  = \overrightarrow {DB} .\) Vậy C đúng.

Đáp án D. Ta có \(\left\{ \begin{array}{l}\overrightarrow {BC}  - \overrightarrow {BA}  = \overrightarrow {AC} \\\overrightarrow {DC}  - \overrightarrow {DA}  = \overrightarrow {AC} \end{array} \right.\). Vậy D đúng.       

Điều kiện cần và đủ để \(I\) là trung điểm của đoạn thẳng \(AB\) là \(\overrightarrow {IA}  =  - \overrightarrow {IB}  \Leftrightarrow \overrightarrow {IA}  + \overrightarrow {IB}  = \overrightarrow 0 \)

Ta có \(\left| {\overrightarrow {AB}  - \overrightarrow {DA} } \right| = \left| {\overrightarrow {AB}  + \overrightarrow {AD} } \right| = \left| {\overrightarrow {AC} } \right| = AC = a\sqrt 2 .\)

Ta có \(\overrightarrow {AB}  =  - \overrightarrow {CD}  = \overrightarrow {DC} \). Do đó:

\(\overrightarrow {AB} \) và \(\overrightarrow {CD} \) ngược hướng.

\(\overrightarrow {AB} \) và \(\overrightarrow {CD} \) cùng độ dài.

\(ABCD\) là hình bình hành nếu \(\overrightarrow {AB} \) và \(\overrightarrow {CD} \) không cùng giá.

\(\overrightarrow {AB}  + \overrightarrow {CD}  = \overrightarrow 0 .\)

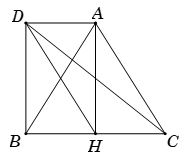
Gọi \(D\) là điểm thỏa mãn tứ giác \(ACHD\) là hình bình hành \(\Rightarrow CH = AD \Rightarrow AD = HB\).

\( \Rightarrow AHBD\) là hình chữ nhật \( \Rightarrow BD = AH\).

\(\left| {\overrightarrow {CA}  - \overrightarrow {HC} } \right| = \left| {\overrightarrow {CA}  + \overrightarrow {CH} } \right| = \left| {\overrightarrow {CD} } \right| = CD.\)

Ta có \(CD = \sqrt {B{D^2} + B{C^2}}  = \sqrt {A{H^2} + B{C^2}} \)\( = \sqrt {\dfrac{{3{a^2}}}{4} + {a^2}}  = \dfrac{{a\sqrt 7 }}{2}\)

Do \(\Delta ABC\) cân tại \(A\), \(AH\) là đường cao nên \(H\) là trung điểm \(BC\).

Xét các đáp án:

Đáp án A. Ta có \(\left\{ \begin{array}{l}\left| {\overrightarrow {AH}  + \overrightarrow {HB} } \right| = \left| {\overrightarrow {AB} } \right| = a\\\left| {\overrightarrow {AH}  + \overrightarrow {HC} } \right| = \left| {\overrightarrow {AC} } \right| = a\end{array} \right.\)

\( \Rightarrow \left| {\overrightarrow {AH}  + \overrightarrow {HB} } \right| = \left| {\overrightarrow {AH}  + \overrightarrow {HC} } \right|.\)

Đáp án B. Ta có \(\left\{ \begin{array}{l}\overrightarrow {AH}  - \overrightarrow {AB}  = \overrightarrow {BH} \\\overrightarrow {AH}  - \overrightarrow {AC}  = \overrightarrow {CH}  =  - \overrightarrow {BH} \end{array} \right..\) Do đó B sai.

Đáp án C. Ta có \(\left\{ \begin{array}{l}\overrightarrow {BC}  - \overrightarrow {BA}  = \overrightarrow {AC} \\\overrightarrow {HC}  - \overrightarrow {HA}  = \overrightarrow {AC} \end{array} \right.\)\( \Rightarrow \overrightarrow {BC}  - \overrightarrow {BA}  = \overrightarrow {HC}  - \overrightarrow {HA} \)

Đáp án D. Ta có \(\left| {\overrightarrow {AB}  - \overrightarrow {AH} } \right| = \left| {\overrightarrow {HB} } \right| = \left| {\overrightarrow {AH} } \right|\) (do \(\Delta ABC\) vuông cân tại \(A\)).

Do \(ABCD\) là hình bình hành nên \(\overrightarrow {BC}  = \overrightarrow {AD} .\)

Suy ra \(\overrightarrow {AB}  - \overrightarrow {BC}  = \overrightarrow {AB}  - \overrightarrow {AD}  = \overrightarrow {DB} .\)

Ta có \(\overrightarrow {AO}  - \overrightarrow {DO}  =  - \overrightarrow {OA}  + \overrightarrow {OD}  = \overrightarrow {OD}  - \overrightarrow {OA}  = \overrightarrow {AD}  = \overrightarrow {BC} \)

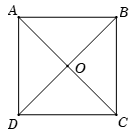
Xét các đáp án:

Đáp án A. Ta có \(\overrightarrow {BC}  + \overrightarrow {AB}  = \overrightarrow {AB}  + \overrightarrow {BC}  = \overrightarrow {AC}  =  - \overrightarrow {CA} .\)

Đáp án B. Ta có \( - \overrightarrow {OA}  + \overrightarrow {OC}  = \overrightarrow {OC}  - \overrightarrow {OA}  = \overrightarrow {AC}  =  - \overrightarrow {CA} .\)

Đáp án C. Ta có \(\overrightarrow {BA}  + \overrightarrow {DA}  =  - \left( {\overrightarrow {AD}  + \overrightarrow {AB} } \right) =  - \overrightarrow {AC}  = \overrightarrow {CA} .\)

Đáp án D. Ta có \(\overrightarrow {DC}  - \overrightarrow {CB}  = \overrightarrow {DC}  + \overrightarrow {BC}  =  - \left( {\overrightarrow {CD}  + \overrightarrow {CB} } \right) =  - \overrightarrow {CA} .\)

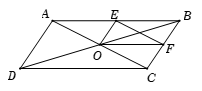
Ta có \(OF,\;OE\) lần lượt là đường trung bình của tam giác \(\Delta BCD\) và \(\Delta ABC\).

\( \Rightarrow BEOF\) là hình bình hành.

\(\overrightarrow {BE}  + \overrightarrow {BF}  = \overrightarrow {BO} \)\( \Rightarrow \overrightarrow {BE}  + \overrightarrow {BF}  - \overrightarrow {DO}  = \overrightarrow {BO}  - \overrightarrow {DO} \) \( = \overrightarrow {OD}  - \overrightarrow {OB}  = \overrightarrow {BD} \)

Ta có \(\left| {\overrightarrow {MB}  - \overrightarrow {MC} } \right| = \left| {\overrightarrow {BM}  - \overrightarrow {BA} } \right| \Leftrightarrow \left| {\overrightarrow {CB} } \right| = \left| {\overrightarrow {AM} } \right| \Rightarrow AM = BC\)

Mà \(A,\;B,\;C\) cố định \( \Rightarrow \) Tập hợp điểm \(M\) là đường tròn tâm \(A\), bán kính \(BC\).

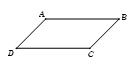
\(\overrightarrow {MA}  + \overrightarrow {MB}  - \overrightarrow {MC}  = \overrightarrow {MD}  \) \(\Leftrightarrow \overrightarrow {MB}  - \overrightarrow {MC}  = \overrightarrow {MD}  - \overrightarrow {MA} \)

\( \Leftrightarrow \overrightarrow {CB}  = \overrightarrow {AD} \): vô lí

\( \Rightarrow \) Không có điểm \(M\) thỏa mãn.

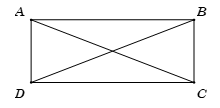
Ta có \(\left\{ \begin{array}{l}\left| {\overrightarrow {AB}  - \overrightarrow {AD} } \right| = \left| {\overrightarrow {DB} } \right| = BD\\\left| {\overrightarrow {AB}  + \overrightarrow {AD} } \right| = \left| {\overrightarrow {AC} } \right| = AC\end{array} \right..\)

$BD = AC \Rightarrow \left| {\overrightarrow {AB} - \overrightarrow {AD} } \right| = \left| {\overrightarrow {AB} + \overrightarrow {AD} } \right|.$

Ta có: $\overrightarrow {AB}  = \overrightarrow {CB}  - \overrightarrow {CA} $ (qui tắc 3 điểm).

Theo qui tắc $3$ điểm ta có: $\overrightarrow {OA}  = \overrightarrow {CA}  - \overrightarrow {CO} $.

Ta có: $\overrightarrow {IA}  - \overrightarrow {IB}  = \overrightarrow {BA}  \ne \overrightarrow 0 $ nên A sai.

Ta có: $\left| {\overrightarrow {OA}  - \overrightarrow {BO} } \right| = \left| {\overrightarrow {CO}  + \overrightarrow {OB} } \right| = \left| {\overrightarrow {CB} } \right| = a$.