Xét các số thực dương x,y thỏa mãn log√3x+yx2+y2+xy+2=x(x−3)+y(y−3)+xy. Tìm giá trị Pmax của biểu thức P=5x+4y+4x+y+3.
log√3x+yx2+y2+xy+2=x(x−3)+y(y−3)+xy(1)⇔log√3(x+y)−log√3(x2+y2+xy+2)=x2−3x+y2−3y+xy⇔log√3(x+y)+3x+3y=log√3(x2+y2+xy+2)+x2+y2+xy⇔log√3(x+y)+2+3x+3y=log√3(x2+y2+xy+2)+x2+y2+xy+2⇔log√3(3x+3y)+3x+3y=log√3(x2+y2+xy+2)+x2+y2+xy+2(2)
Đặt f(t)=log√3t+t(t>0)⇒f′(t)=1tln√3+1>0,∀t>0
⇒f(t) đồng biến trên (0;+∞)
(2)⇔f(3x+3y)=f(x2+y2+xy+2)⇔3x+3y=x2+y2+xy+2⇔4x2+4y2+4xy−12x−12y+8=0⇔(2x+y)2−6(2x+y)+5=−3(y−1)2≤0⇔1≤2x+y≤5
Khi đó, P=5x+4y+4x+y+3=3+2x+y−5x+y+3≤3, vì {2x+y−5≤0x+y+3>0
Vậy Pmax=3 khi và chỉ khi {2x+y−5=0y−1=0⇔{x=2y=1
Có bao nhiêu giá trị nguyên m để phương trình 91+x+91−x=(m+2)(32+x−32−x)+45−27m có nghiệm trên [0;1]
Đặt t=31+x−31−x=f(x),∀x∈[0;1] ta có f′(x)=31+xln3+31−xln3>0
⇒ hàm số đồng biến trên [0;1], ⇒f(0)=0≤f(x)≤f(1)=8⇒f(x)∈[0;8] hay t∈[0;8]
Ta có t2=91+x+91−x−2.31+x+1−x=91+x+91−x−18⇔91+x+91−x=t2+18
Khi đó phương trình trở thành t2+18=3(m+2)t+45−27m
⇔t2−3(m+2)t+27m−27=0∀t∈[0;8]⇔t2−6t−27−3m(t−9)=0⇔(t−9)(t+3)−3m(t−9)=0⇔(t−9)(t+3−3m)=0⇔[t=9∉[0;8]t=3m−3
Đề phương trình ban đầu có nghiệm x∈[0;1] thì phương trình (*) có nghiệm t∈[0;8] thì
0≤3m−3≤8⇔1≤m≤113m∈Z⇔m∈{1;2;3}
Vậy có 3 giá trị m nguyên để phương trình ban đầu có nghiệm thuộc [0;1]
Xét các số thực dương x,y thỏa mãn log13x+log13y≤log13(x2+y). Tìm giá trị nhỏ nhất Pmin của biểu thức P=3x+2y.
log13x+log13y≤log13(x2+y)⇔log13(xy)≤log13(x2+y)⇔xy≥x2+y⇔y(x−1)≥x2
Với x = 1 ta có 0≥1 (Vô lý) ⇒x≠1 .
Ta có y(x−1)=x2≥0, mà y>0⇒x−1≥0⇔x≥1. Vậy x>1
Khi đó ta có :
P=3x+2y=(3x+2y)(x−1)x−1=3x(x−1)+2y(x−1)x−1≥3x2−3x+2x2x−1=5x2−3xx−1=f(x)∀x>1
Xét hàm số f(x)=5x2−3xx−1=5x+2+2x−1(x>1) ta có
f′(x)=5−2(x−1)2=5(x−1)2−2(x−1)2=0⇔[x=1+√105x=1−√105
BBT :
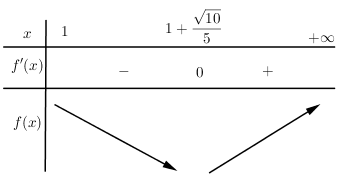
Dựa vào BBT ta thấy
min(1;+∞)f(x)=f(1+√105)=5(1+√105)+2+21+√105−1=5+√10+2+√10=7+2√10⇒P≥f(x)≥7+2√10⇒Pmin=7+2√10
Tìm tất cả các giá trị của tham số m để phương trình(3−√5)x2+m(3+√5)x2−2x2−1=0 có đúng hai nghiệm phân biệt?
(3−√5)x2+m(3+√5)x2−2x2−1=0⇔(3−√52)x2+m(3+√52)x2−12=0
Ta có (3−√52)x2.(3+√52)x2=(3−√52.3+√52)x2=1
Đặt (3−√52)x2=t⇔(3+√52)x2=1t⇒t=(23+√5)x2⇔x2=log23+√5t, khi đó phương trình trở thành: t+m1t−12=0⇔t2−12t+m=0 ⇔2t2−t+2m=0 ⇔m=t−2t22
Phương trình đã cho có hai nghiệm phân biệt ⇔x2>0⇔log23+√5t>0⇔0<t<1.
(ứng với mỗi nghiệm t thỏa mãn 0<t<1 thì ta có 2 nghiệm x)
Do đó ta cần tìm m để phương trình ẩn t chỉ có 1 nghiệm thuộc (0;1).
Xét hàm f(t)=t−2t22 có f′(t)=1−4t2=0⇔t=14
Bảng biến thiên:
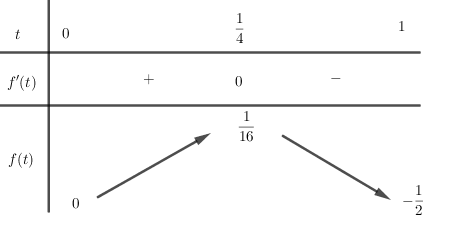
Vậy −12<m≤0 hoặc m=116
Cho f(x)=a.ln(x+√x2+1)+b.x2017+2018 với a,b∈R. Biết rằng f(log(loge))=2019. Tính giá trị của f(log(ln10)).
Đặt g(x)=f(x)−2018=a.ln(x+√x2+1)+b.x2017
⇒g(−x)=a.ln(−x+√x2+1)−b.x2017=a.ln(1x+√x2+1)−b.x2017=−a.ln(x+√x2+1)−b.x2017=−g(x)⇒f(−x)−2018=−(f(x)−2018)⇔f(−x)=−f(x)+4036
Ta có:
f(log(ln10))=f(log(log10loge))=f(log(1loge))=f(−log(loge))=−f(log(loge))+4036=−2019+4036=2017
Tập hợp S tất cả các giá trị của tham số m để phương trình2(x−1)2.log2(x2−2x+3)=4|x−m|.log2(2|x−m|+2) có đúng ba nghiệm phân biệt là
Ta có: 2(x−1)2.log2(x2−2x+3)=4|x−m|.log2(2|x−m|+2)
⇔2(x−1)2.log2((x−1)2+2)=22|x−m|.log2(2|x−m|+2) (1)
Xét hàm số y=f(t)=2t.log2(t+2) trên [0;+∞):
f′(t)=2tln2.log2(t+2)+2t.1ln2.(t+2)>0,∀t≥0⇒Hàm số đồng biến trên [0;+∞)
Phương trình (1)⇔f((x−1)2)=f(2|x−m|)⇔(x−1)2=2|x−m|(2)
Thử đáp án:
+) Nếu m=1 thì (2) trở thành (x−1)2=2|x−1|
Nếu x≥1 thì (x−1)2=2(x−1)⇔(x−1)(x−3)=0⇔[x=1(TM)x=3(TM)
Nếu x<1 thì (x−1)2=−2(x−1)⇔(x−1)(x+1)=0⇔[x=1(L)x=−1(TM)
Do đó (2) có 3 nghiệm phân biệt nên m=1 thỏa bài toán.
+) Nếu m=12 thì (2) trở thành (x−1)2=2|x−12|
Nếu x≥12 thì (x−1)2=2(x−12)⇔x2−4x+2=0⇔[x=2+√2(TM)x=2−√2(TM)
Nếu x<12 thì (x−1)2=−2(x−12)⇔x2=0⇔x=0(TM)
Do đó m=12 thỏa mãn bài toán.
+) Nếu m=32 thì (2) trở thành (x−1)2=2|x−32|
Nếu x≥32 thì (x−1)2=2(x−32)⇔x2−4x+4=0⇔x=2(TM)
Nếu x<32 thì (x−1)2=−2(x−32)⇔x2=2⇔x=±√2(TM)
Do đó m=32 thỏa mãn bài toán.
Vậy các giá trị m=1,m=12,m=32 đều thỏa mãn.
Cho phương trình 2x+m=log2(x−m) với m là tham số. Có bao nhiêu giá trị nguyên của m∈(−18;18) để phương trình đã cho có nghiệm?
Điều kiện: x−m>0⇔x>m.
Đặt: 2x+m=log2(x−m)=y⇒{2x+m=ylog2(x−m)=y⇔{2x+m=yx−m=2y⇔{2x+x=2y+y(∗)m=x−2y(∗∗).
Xét hàm số đặc trưng: f(t)=2t+t ta có: f′(t)=2tln2+1>0,∀t.
⇒f(t) đồng biến trên R.
Khi đó ta có: (∗)⇔f(x)=f(y)⇔x=y.
⇒(∗∗)⇔m=x−2x(∗∗∗).
Xét hàm số: g(x)=x−2x có: g′(x)=1−2xln2.
⇒g′(x)=0⇔1−2xln2=0⇔2x=1ln2 ⇔x=log2(1ln2)=−log2(ln2).
Ta có BBT:
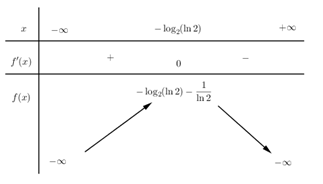
⇒(∗∗∗) có nghiệm ⇔m≤−log2(ln2)−1ln2≈−0,914
Với m∈(−18;18) và m∈Z⇒m∈{−17;−16;....;−2;−1}.
Vậy có 17 giá trị m thỏa mãn.
Cho a>0,b>0 thỏa mãn log2a+2b+1(4a2+b2+1)+log4ab+1(2a+2b+1)=2. Giá trị của a+2b bằng:
Ta có: log2a+2b+1(4a2+b2+1)+log4ab+1(2a+2b+1)=2
⇔log2a+2b+1(4a2+b2+1)+1log2a+2b+1(4ab+1)=2.
Có: (2a)2+b2≥2.2a.b⇔4a2+b2≥4ab.
⇒4a2+b2+1≥4ab+1.
Dấu “=” xảy ra ⇔2a=b.
Theo giả thiết ta có: {a>0b>0⇒{2a+2b+1>14ab+1>1⇒{log2a+2b+1(4a2+b2+1)≥log2a+2b+1(4ab+1)log4ab+1(2a+2b+1)=1log2a+2b+1(4ab+1)
Áp dụng bất đẳng thức Cauchy ta có:
log2a+2b+1(4a2+b2+1)+1log2a+2b+1(4ab+1)≥log2a+2b+1(4ab+1)+1log2a+2b+1(4ab+1) ≥2.√log2a+2b+1(4ab+1).1log2a+2b+1(4ab+1)=2.
Dấu “=” xảy ra ⇔{2a=blog2a+2b+1(4ab+1)=1log2a+2b+1(4ab+1)
⇔{2a=blog23b+1(2b2+1)=1 ⇔{2a=blog3b+1(2b2+1)=1 (vì log3b+1(2b2+1)>0)
⇔{b=2a2b2+1=3b+1⇔{b=2a2b2−3b=0 ⇔{b=2a[b=0(ktm)b=32(tm)⇔{a=34(tm)b=32
Vậy a+2b=34+3=154.
Cho phương trình 12log2(x+2)+x+3=log22x+1x+(1+1x)2+2√x+2, gọi S là tổng tất cả các nghiệm của nó. Khi đó, giá trị của S là:
Điều kiện: x>0 hoặc −2<x<−12
12log2(x+2)+x+3=log22x+1x+(1+1x)2+2√x+2⇔log2√x+2+x+2+1−2√x+2=log2(2+1x)+1+2x+1x2⇔log2√x+2+(√x+2)2−2√x+2=log2(2+1x)+2x+1x2⇔log2√x+2+(√x+2)2−2√x+2=log2(2+1x)+(2+1x)2−2(2+1x)(∗)
Xét hàm số: f\left( t \right) = {\log _2}t + {t^2} - 2\sqrt t ,\left( {t > 0} \right).
Ta có: f'\left( t \right) = \dfrac{1}{{t.\ln 2}} + 2t - \dfrac{1}{{\sqrt t }} > 0,\forall t > 0 \Rightarrow Hàm số đồng biến trên \left( {0; + \infty } \right)
Mà theo \left( * \right) ta có: f\left( {\sqrt {x + 2} } \right) = f\left( {2 + \dfrac{1}{x}} \right)
\begin{array}{l} \Leftrightarrow \sqrt {x + 2} = 2 + \dfrac{1}{x}\\ \Leftrightarrow x + 2 = 4 + \dfrac{4}{x} + \dfrac{1}{{{x^2}}}\\ \Leftrightarrow {x^3} + 2{x^2} - 4{x^2} - 4x - 1 = 0\\ \Leftrightarrow {x^3} - 2{x^2} - 4x - 1 = 0\\ \Leftrightarrow \left( {x + 1} \right)\left( {{x^2} - 3x - 1} \right) = 0 \Leftrightarrow \left[ \begin{array}{l}x = - 1\;\;\;\left( {tm} \right)\\x = \dfrac{{3 + \sqrt {13} }}{2}\;\;\;\left( {tm} \right)\\x = \dfrac{{3 - \sqrt {13} }}{2}\;\;\left( {ktm} \right)\end{array} \right..\\ \Rightarrow S = - 1 + \dfrac{{3 + \sqrt {13} }}{2} = \dfrac{{1 + \sqrt {13} }}{2}\end{array}
Cho hàm số f\left( x \right) = \dfrac{{{4^x}}}{{{4^x} + 2}}. Tính tổng S = f\left( {\dfrac{1}{{2019}}} \right) + f\left( {\dfrac{2}{{2019}}} \right) + \,\,...\,\, + f\left( {\dfrac{{2018}}{{2019}}} \right) + f\left( 1 \right).
Ta có f\left( x \right) = \dfrac{{{4^x}}}{{{4^x} + 2}} \Rightarrow f\left( {1 - x} \right) = \dfrac{{{4^{1\, - \,x}}}}{{{4^{1\, - \,x}} + 2}}
\Rightarrow f\left( x \right) + f\left( {1 - x} \right) = \dfrac{{{4^x}}}{{{4^x} + 2}} + \dfrac{{{4^{1 - x}}}}{{{4^{1 - x}} + 2}} = \dfrac{{{4^x}}}{{{4^x} + 2}} + \dfrac{4}{{4 + {{2.4}^x}}} = \dfrac{{{4^x}}}{{{4^x} + 2}} + \dfrac{2}{{{4^x} + 2}} = 1
Khi đó f\left( {\dfrac{1}{{2019}}} \right) + f\left( {\dfrac{{2018}}{{2019}}} \right) = 1; f\left( {\dfrac{2}{{2019}}} \right) + f\left( {\dfrac{{2017}}{{2019}}} \right) = 1; … và f\left( 1 \right) = \dfrac{4}{6}.
Vậy S = f\left( {\dfrac{1}{{2019}}} \right) + f\left( {\dfrac{2}{{2019}}} \right) + \,\,...\,\, + f\left( {\dfrac{{2018}}{{2019}}} \right) + f\left( 1 \right) = \dfrac{{2018}}{2}.1 + \dfrac{4}{6} = \dfrac{{3029}}{3}.
Cho hệ \left\{ \begin{array}{l}9{x^2} - 4{y^2} = 5\\{\log _m}(3x + 2y) - {\log _3}(3x - 2y) = 1\end{array} \right. có nghiệm \left( {x;y} \right) thỏa mãn 3x + 2y \le 5. Khi đó giá trị lớn nhất của m là:
Ta có: 9{x^2} - 4{y^2} = 5 \Leftrightarrow (3x - 2y)(3x + 2y) = 5 \Leftrightarrow 3x - 2y = \dfrac{5}{{3x + 2y}}
Khi đó, ta có:
\begin{array}{l}{\log _m}(3x + 2y) - {\log _3}(3x - 2y) = 1 \Leftrightarrow {\log _m}(3x + 2y) - {\log _3}\dfrac{5}{{3x + 2y}} = 1\\ \Leftrightarrow \dfrac{{{{\log }_3}(3x + 2y)}}{{{{\log }_3}m}} - {\log _3}5 + {\log _3}(3x + 2y) = 1\\ \Leftrightarrow \dfrac{{{{\log }_3}(3x + 2y)}}{{{{\log }_3}m}} = {\log _3}5 - {\log _3}(3x + 2y) + 1\\ \Leftrightarrow {\log _3}m = \dfrac{{{{\log }_3}(3x + 2y)}}{{{{\log }_3}5 - {{\log }_3}(3x + 2y) + 1}}\,\,(1)\end{array}
Đặt t = {\log _3}\left( {3x + 2y} \right) \le {\log _3}5 thì \left( 1 \right) trở thành {\log _3}m = \dfrac{t}{{{{\log }_3}5 - t + 1}}
Xét hàm số y = f(t) = \dfrac{t}{{{{\log }_3}5 - t + 1}},t \in \left( { - \infty ;{{\log }_3}5} \right]
f'(t) = \dfrac{{{{\log }_3}5 + 1}}{{{{({{\log }_3}5 - t + 1)}^2}}} > 0,\,\,\forall t \in \left( { - \infty ;{{\log }_3}5} \right]
Bảng biến thiên:
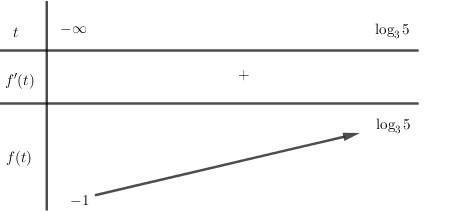
Để \left( 1 \right) có nghiệm thì - 1 < \log { _3}m \le {\log _3}5\, \Rightarrow \dfrac{1}{3} < m \le 5
Vậy giá trị lớn nhất của m thỏa mãn yêu cầu đề bài là 5.
Biết rằng a là số thực dương sao cho bất đẳng thức {3^x} + {a^x} \ge {6^x} + {9^x} đúng với mọi số thực x. Mệnh đề nào sau đây đúng?
Ta có {3^x} + {a^x} \ge {6^x} + {9^x} \Leftrightarrow f\left( x \right) = {3^x} + {a^x} - {6^x} - {9^x} \ge 0;\,\,\forall x \in \mathbb{R}.
Xét f\left( x \right) = {3^x} + {a^x} - {6^x} - {9^x} trên \mathbb{R}, có:
f'\left( x \right) = {3^x}.\ln 3 + {a^x}.\ln a - {6^x}.\ln 6 - {9^x}.\ln 9; f''\left( x \right) = {3^x}{\ln ^2}3 + {a^x}{\ln ^2}a - {6^x}{\ln ^2}6 - {9^x}{\ln ^2}9
Dễ thấy f\left( 0 \right) = 0 nên f\left( x \right) \ge 0,\forall x \in R \Leftrightarrow f\left( x \right) \ge f\left( 0 \right),\forall x \in R
Điều này cũng có nghĩa x = 0 là điểm cực tiểu của hàm số.
\Leftrightarrow \left\{ \begin{array}{l}f'\left( 0 \right) = 0\\f''\left( 0 \right) > 0\end{array} \right. \Leftrightarrow \,\left\{ \begin{array}{l}\ln a = \ln \dfrac{{6\,\, \times \,\,9}}{3}\\{\ln ^2}3 + {\ln ^2}a - {\ln ^2}6 - {\ln ^2}9 > 0\end{array} \right.\, \Leftrightarrow a = 18

