Hàm số y=f(x) có nguyên hàm trên (a;b) đồng thời thỏa mãn f(a)=f(b). Lựa chọn phương án đúng:
Đặt t=f(x)⇒dt=f′(x)dx
Đổi cận: {x=a⇒t=f(a)x=b⇒t=f(b)
Khi đó I=f(b)∫f(a)etdt=0 (Vì f(a)=f(b))
Cho hàm số y=f(x) có đồ thị như hình vẽ bên. Tính tích phân I=3∫−1f(2x−1)dx.
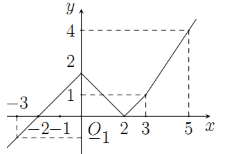
Đặt 2x−1=t⇒2dx=dt. Đổi cận {x=−1⇒t=−3x=3⇒t=5.
Khi đó: I=3∫−1f(2x−1)dx=5∫−3f(t)12dt=125∫−3f(t)dt
=12(−2∫−3f(x)dx+0∫−2f(x)dx+2∫0f(x)dx+3∫2f(x)dx+5∫3f(x)dx)=12(−SΔABC+SΔOCD+SΔOED+SΔEFI+SIFHK)=12(−12+2+2+12+5)=92.
Cho hàm số y=f(x) liên tục trên R, thỏa mãn f(2x3+x2+1)=x+2 ∀x∈R. Tính tích phân ∫41f(x)dx
Bước 1: Biểu diễn (6x2+2x)f(2x3+x2+1)
Khi x≥0, ta có:
f(2x3+x2+1)=x+2⇔(6x2+2x)f(2x3+x2+1)=(6x2+2x)(x+2)(∗)
Bước 2: Sử dụng phương pháp đổi biến số tính ∫41f(x)dx
Lấy tích phân từ 0 đến 1 hai vế của (∗) ta được
∫10(6x2+2x)f(2x3+x2+1)dx=∫10(6x2+2x)(x+2)dx
⇔∫10f(2x3+x2+1)d(2x3+x2+1)=496
Đặt t=2x3+x2+1
Đổi cận:x=0⇒t=1;x=1⇒t=4
⇒∫41f(t)dt=496⇔∫41f(x)dx=496
Cho hàm số f(x) liên tục trên R và 4∫−2f(x)dx=2 . Mệnh đề nào sau đây là sai?
Dựa vào các đáp án, xét:
2∫−1f(2x)dx=122∫−1f(2x)d(2x)=124∫−2f(x)dx=1
3∫−3f(x+1)dx=3∫−3f(x+1)d(x+1)=4∫−2f(x)dx=2
6∫012f(x−2)dx=6∫012f(x−2)d(x−2)=124∫−2f(x)dx=1
Do đó các đáp án B, C, D đều đúng, đáp án A sai.
Đề thi THPT QG 2019 – mã đề 104
Cho hàm số f(x) có đạo hàm liên tục trên R. Biết f(3)=1 và 1∫0xf(3x)dx=1, khi đó 3∫0x2f′(x)dx bằng:
Xét 1∫0xf(3x)dx=1.
Đặt t=3x⇔dt=3dx. Đổi cận: {x=0⇒t=0x=1⇒t=3.
⇒1∫0xf(3x)dx=1⇔3∫0t3f(t)dt3=1⇔193∫0tf(t)dt=1
⇔3∫0tf(t)dt=9⇔23∫0xf(x)dx=18⇒3∫02xf(x)dx=18
Ta có: 3∫0[x2f′(x)+2xf(x)]dx=3∫0x2f′(x)dx+3∫02xf(x)dx
⇔3∫0(x2f(x))′dx=3∫0x2f′(x)dx+18⇔x2f(x)|30=3∫0x2f′(x)dx+18⇔9f(3)=3∫0x2f′(x)dx+18⇔9.1=3∫0x2f′(x)dx+18⇔3∫0x2f′(x)dx=9−18=−9
Cho tích phân I=1∫03√1−xdx. Với cách đặt t=3√1−x ta được:
Đặt t=3√1−x⇒t3=1−x⇒3t2dt=−dx⇔dx=−3t2dt
Với x=0⇒t=1 ; x=1⇒t=0
Khi đó I=0∫1t.(−3t2)dt=31∫0t3dt
Đổi biến x=4sint của tích phân I=√8∫0√16−x2dx ta được:
Bước 1:
Đặt x=4sint
Bước 2:
Đổi cận: {x=0⇒sint=0⇒t=0x=√8⇒sint=√22⇒t=π4
Bước 3:
Ta có: x=4sint⇒dx=d(4sint)=(4sint)′dt=4costdt
Bước 4:
Khi đó ta có: I=4π4∫0√16−16sin2tcostdt=4π4∫04.cos2tdt=16π4∫0cos2tdt =8π4∫0(1+cos2t)dt
Cho 3∫1f(x)dx=4. Tính 1∫0f(2x+1)dx.
Ta có: 3∫1f(x)dx=4.
Đặt 2x+1=t⇒dt=2dx⇒dx=12dt
Đổi cận: {x=0⇒t=1x=1⇒t=3
⇒I=1∫0f(2x+1)dx=123∫1f(t)dt=12.4=2.
Cho hàm số f(x) liên tục trên R và thỏa mãn 1∫−5f(x)dx=9. Tính tích phân 2∫0[f(1−3x)+9]dx.
Ta có
I=2∫0[f(1−3x)+9]dx⇔I=2∫0f(1−3x)dx+2∫09dx
Đặt I1=2∫0f(1−3x)dx.
Đặt t=1−3x⇒dt=−3dx.
Đổi cận: {x=0⇒t=1x=2⇒t=−5.
⇒I1=−13−5∫1f(t).dt=131∫−5f(x)dx=13.9=3.
Đặt I2=2∫09dx=9x|20=18.
Vậy I=I1+I2=3+18=21.
Cho f(x) là hàm liên tục trên R thỏa mãn 1∫0f(x)dx=4 và 1∫0f(3x)dx=6. Tích phân 3∫1f(x)dx bằng:
Ta có: 1∫0f(x)dx=4 và 1∫0f(3x)dx=6.
Đặt t=3x⇒dt=3dx⇒dx=13dt
Đổi cận: {x=0⇒t=0x=1⇒t=3
⇒1∫0f(3x)dx=6⇔3∫013f(t)dt=6⇔3∫0f(t)dt=18⇒3∫0f(x)dx=18⇒3∫1f(x)dx=3∫0f(x)dx−1∫0f(x)dx⇔3∫1f(x)dx=18−4=14.
Cho tích phân I=4∫0x√x2+9dx. Khi đặt t=√x2+9 thì tích phân đã cho trở thành:
Đặt t=√x2+9⇒t2=x2+9 ⇒tdt=xdx.
Đổi cận: {x=0⇒t=3x=4⇒t=5.
Vậy I=4∫0x√x2+9dx=5∫3t.tdt=5∫3t2dt.
Cho tích phân I=e∫1√1+3lnxxdx, đặt t=√1+3lnx. Khẳng định nào dưới đây đúng ?
Đặt t=√1+3lnx⇒t2=1+3lnx⇒2tdt=3xdx⇒1xdx=23tdt
Đổi cận: {x=1⇒t=1x=e⇒t=2.
Khi đó ta có: I=2∫1t.23tdt=232∫1t2dt
Cho I=3∫0x1+√x+1dx. Nếu đặt t=√x+1 thì I=2∫1f(t)dt, trong đó f(t) bằng:
Ta có: I=3∫0x1+√x+1dx
Đặt t=√x+1 ⇒t2=x+1⇒dx=2tdt
Đổi cận: {x=0⇒t=1x=3⇒t=2
⇒I=2∫0t2−11+t2tdt =22∫0(t−1)(t+1)t+1tdt = 2\int\limits_0^2 {t\left( {t - 1} \right)dt = 2\int\limits_0^2 {\left( {{t^2} - t} \right)dt} }
\Rightarrow f\left( t \right) = 2\left( {{t^2} - t} \right) = 2{t^2} - 2t.
Biết \int\limits_0^{\ln 2} {\dfrac{{{e^{2x}}}}{{{e^x} + 1}}dx} = a + \ln \dfrac{b}{c} với a,\,\,b,\,\,c \in {\mathbb{N}^*} và \dfrac{b}{c} là phân số tối giản. Giá trị a - b + c bằng:
Đặt t = {e^x} + 1 \Rightarrow dt = {e^x}dx.
Đổi cận: \left\{ \begin{array}{l}x = 0 \Rightarrow t = 2\\x = \ln 2 \Rightarrow t = 3\end{array} \right..
Khi đó ta có
\begin{array}{l}\int\limits_0^{\ln 2} {\dfrac{{{e^{2x}}}}{{{e^x} + 1}}dx} = \int\limits_0^{\ln 2} {\dfrac{{{e^x}}}{{{e^x} + 1}}.{e^x}dx} = \int\limits_2^3 {\dfrac{{t - 1}}{t}dt} \\ = \int\limits_2^3 {\left( {1 - \dfrac{1}{t}} \right)dt} = \left. {\left( {t - \ln t} \right)} \right|_2^3 = 1 - \ln 3 + \ln 2 = 1 + \ln \dfrac{2}{3}\\ \Rightarrow a = 1,\,\,b = 2,\,\,c = 3\end{array}
Vậy a - b + c = 1 - 2 + 3 = 2.
Cho hàm số y = f\left( x \right) liên tục trên tập số thực thỏa mãn f\left( x \right) + \left( {5x - 2} \right)f\left( {5{x^2} - 4x} \right) = 50{x^3} - 60{x^2} + 23x - 1 \forall x \in \mathbb{R}. Giá trị của biểu thức \int\limits_0^1 {f\left( x \right)dx} bằng:
Lấy tích phân từ 0 đến 1 hai vế ta được:
\int\limits_0^1 {f\left( x \right)dx} + \int\limits_0^1 {\left( {5x - 2} \right)f\left( {5{x^2} - 4x} \right)dx} = \int\limits_0^1 {\left( {50{x^3} - 60{x^2} + 23x - 1} \right)dx}
Xét tích phân I = \int\limits_0^1 {\left( {5x - 2} \right)f\left( {5{x^2} - 4x} \right)dx} .
Đặt t = 5{x^2} - 4x ta có dt = \left( {10x - 4} \right)dx \Leftrightarrow \left( {5x - 2} \right)dx = \dfrac{1}{2}dt.
Đổi cận: \left\{ \begin{array}{l}x = 0 \Rightarrow t = 0\\x = 1 \Rightarrow t = 1\end{array} \right..
\Rightarrow I = \int\limits_0^1 {\dfrac{1}{2}f\left( t \right)dt} = \dfrac{1}{2}\int\limits_0^1 {f\left( x \right)dx}
Xét tích phân J = \int\limits_0^1 {\left( {50{x^3} - 60{x^2} + 23x - 1} \right)dx} ta có: J = \left. {\left( {\dfrac{{50{x^4}}}{4} - \dfrac{{60{x^3}}}{3} + \dfrac{{23{x^2}}}{2} - x} \right)} \right|_0^1 = 3
Khi đó ta có \int\limits_0^1 {f\left( x \right)dx} + \dfrac{1}{2}\int\limits_0^1 {f\left( x \right)dx} = 3 \Leftrightarrow \dfrac{3}{2}\int\limits_0^1 {f\left( x \right)dx} = 3 \Leftrightarrow \int\limits_0^1 {f\left( x \right)dx} = 2.
Cho f\left( x \right) là hàm số liên tục trên tập số thực \mathbb{R} và thỏa mãn f\left( {{x^2} + 3x + 1} \right) = x + 2. Tính I = \int\limits_1^5 {f\left( x \right)dx}
Theo bài ra ta có
\begin{array}{l}f\left( {{x^2} + 3x + 1} \right) = x + 2\\ \Rightarrow f\left( {{x^2} + 3x + 1} \right)\left( {2x + 3} \right) = \left( {x + 2} \right)\left( {2x + 3} \right)\\ \Rightarrow \int\limits_0^1 {f\left( {{x^2} + 3x + 1} \right)\left( {2x + 3} \right)dx} = \int\limits_0^1 {\left( {x + 2} \right)\left( {2x + 3} \right)dx} \\ \Rightarrow \int\limits_0^1 {f\left( {{x^2} + 3x + 1} \right)\left( {2x + 3} \right)dx} = \dfrac{{61}}{6}\end{array}
Đặt t = {x^2} + 3x + 1 \Rightarrow dt = \left( {2x + 3} \right)dx.
Đổi cận: \left\{ \begin{array}{l}x = 0 \Rightarrow t = 1\\x = 1 \Rightarrow t = 5\end{array} \right..
\Rightarrow \int\limits_0^1 {f\left( {{x^2} + 3x + 1} \right)\left( {2x + 3} \right)dx} = \int\limits_1^5 {f\left( t \right)dt} = \int\limits_1^5 {f\left( x \right)dx} .
Vậy \int\limits_1^5 {f\left( x \right)dx} = \dfrac{{61}}{6}.
Cho hàm số y = f\left( x \right) liên tục trên \mathbb{R} thỏa mãn \int\limits_0^7 {f\left( x \right)dx} = 10 và \int\limits_0^3 {f\left( x \right)dx} = 6. Tính I = \int\limits_{ - 2}^3 {f\left| {3 - 2x} \right|dx} .
Đặt t = 3 - 2x \Rightarrow dt = - 2dx. Đổi cận \left\{ \begin{array}{l}x = - 2 \Rightarrow t = 7\\x = 3 \Rightarrow t = - 3\end{array} \right..
Khi đó ta có:
\begin{array}{l}I = - \dfrac{1}{2}\int\limits_7^{ - 3} {f\left( {\left| t \right|} \right)dt} = \dfrac{1}{2}\int\limits_{ - 3}^7 {f\left( {\left| t \right|} \right)dt} \\\,\,\,\, = \dfrac{1}{2}\left( {\int\limits_{ - 3}^0 {f\left( { - t} \right)dt} + \int\limits_0^7 {f\left( t \right)dt} } \right)\\\,\,\,\, = \dfrac{1}{2}\left( { - \int\limits_3^0 {f\left( x \right)dx} + \int\limits_0^7 {f\left( x \right)dx} } \right)\\\,\,\,\, = \dfrac{1}{2}\left( {\int\limits_0^3 {f\left( x \right)dx} + \int\limits_0^7 {f\left( x \right)dx} } \right)\\\,\,\,\, = \dfrac{1}{2}\left( {6 + 10} \right) = 8\end{array}.
Cho \int\limits_0^{\frac{\pi }{2}} {\dfrac{{\cos x}}{{{{\sin }^2}x - 5\sin x + 6}}dx} = a\ln \dfrac{4}{b}. Giá trị của a + b bằng:
Đặt t = \sin x \Rightarrow dt = \cos xdx.
Đổi cận: \left\{ \begin{array}{l}x = 0 \Rightarrow t = 0\\x = \dfrac{\pi }{2} \Rightarrow t = 1\end{array} \right., khi đó
\begin{array}{l}\int\limits_0^{\frac{\pi }{2}} {\dfrac{{\cos x}}{{{{\sin }^2}x - 5\sin x + 6}}dx} = \int\limits_0^1 {\dfrac{{dt}}{{{t^2} - 5t + 6}}} \\ = \int\limits_0^1 {\dfrac{{dt}}{{\left( {t - 2} \right)\left( {t - 3} \right)}}} = \int\limits_0^1 {\left( {\dfrac{1}{{t - 3}} - \dfrac{1}{{t - 2}}} \right)dt} \\ = \left. {\left( {\ln \left| {t - 3} \right| - \ln \left| {t - 2} \right|} \right)} \right|_0^1 = \left. {\ln \left| {\dfrac{{t - 3}}{{t - 2}}} \right|} \right|_0^1\\ = \ln 2 - \ln \dfrac{3}{2} = \ln \dfrac{4}{3}\end{array}
\Rightarrow a = 1,\,\,b = 3.
Vậy a + b = 1 + 3 = 4.
Cho \int\limits_0^1 {\dfrac{x}{{{{\left( {x + 2} \right)}^2}}}dx} = a + b\ln 2 + c\ln 3, với a, b, c là các số hữu tỷ. Giá trị của 3a + b + c bằng :
\begin{array}{l}\int\limits_0^1 {\dfrac{{xdx}}{{{{\left( {x + 2} \right)}^2}}} = \int\limits_0^1 {\dfrac{{x + 2}}{{{{\left( {x + 2} \right)}^2}}}dx} - \int\limits_0^1 {\dfrac{2}{{{{\left( {x + 2} \right)}^2}}}dx} } \\= \left. {\left( {\ln \left| {x + 2} \right| + \dfrac{2}{{x + 2}}} \right)} \right|_0^1\\ = \ln 3 + \dfrac{2}{3} - \ln 2 - 1 = \ln 3 - \ln 2 - \dfrac{1}{3}\\ \Rightarrow \left\{ \begin{array}{l}a = - \dfrac{1}{3}\\b = - 1\\c = 1\end{array} \right. \Rightarrow 3a + b + c = 3.\left( { - \dfrac{1}{3}} \right) - 1 + 1 = - 1.\end{array}
Biết \int\limits_{\dfrac{\pi }{4}}^{\dfrac{\pi }{3}} {\dfrac{{{{\cos }^2}x + \sin x\cos x + 1}}{{{{\cos }^4}x + \sin x{{\cos }^3}x}}dx} = a + b\ln 2 + c\ln \left( {1 + \sqrt 3 } \right), với a,\,\,b,\,\,c là các số hữu tỉ. Giá trị của abc bằng:
I = \int\limits_{\dfrac{\pi }{4}}^{\dfrac{\pi }{3}} {\dfrac{{{{\cos }^2}x + \sin x\cos x + 1}}{{{{\cos }^4}x + \sin x{{\cos }^3}x}}dx} = \int\limits_{\dfrac{\pi }{4}}^{\dfrac{\pi }{3}} {\dfrac{{1 + \tan x + 1 + {{\tan }^2}x}}{{{{\cos }^2}x\left( {1 + \tan x} \right)}}dx} = \int\limits_{\dfrac{\pi }{4}}^{\dfrac{\pi }{3}} {\dfrac{{{{\tan }^2}x + \tan x + 2}}{{{{\cos }^2}x\left( {1 + \tan x} \right)}}dx}
Đặt t = \tan x \Rightarrow dt = \dfrac{1}{{{{\cos }^2}x}}dx. Đổi cận \left\{ \begin{array}{l}x = \dfrac{\pi }{4} \Rightarrow t = 1\\x = \dfrac{\pi }{3} \Rightarrow t = \sqrt 3 \end{array} \right..
\begin{array}{l} \Rightarrow I = \int\limits_1^{\sqrt 3 } {\dfrac{{{t^2} + t + 2}}{{t + 1}}dt} = \int\limits_1^{\sqrt 3 } {\left( {t + \dfrac{2}{{t + 1}}} \right)dt} \\ = \left. {\dfrac{{{t^2}}}{2} + 2\ln \left| {t + 1} \right|} \right|_1^{\sqrt 3 } = \dfrac{3}{2} + 2\ln \left( {\sqrt 3 + 1} \right) - \dfrac{1}{2} - 2\ln 2 = 1 - 2\ln 2 + 2\ln \left( {1 + \sqrt 3 } \right)\\ \Rightarrow \left\{ \begin{array}{l}a = 1\\b = - 2\\c = 2\end{array} \right. \Rightarrow abc = 1.\left( { - 2} \right).2 = - 4\end{array}

