Phương trình logarit và một số phương pháp giải

Đặt ${\log _4}a = {\log _6}b = {\log _9}\left( {a + b} \right) = x \Leftrightarrow \left\{ \begin{array}{l}a = {4^x}\\b = {6^x}\\a + b = {9^x}\end{array} \right. \Rightarrow \left\{ \begin{array}{l}\dfrac{a}{b} = {\left( {\dfrac{4}{6}} \right)^x} = {\left( {\dfrac{2}{3}} \right)^x} > 0\\{4^x} + {6^x} = {9^x}(1)\end{array} \right.$

giải (1) \({4^x} + {6^x} = {9^x} \Leftrightarrow {\left( {\dfrac{2}{3}} \right)^{2x}} + {\left( {\dfrac{2}{3}} \right)^x} - 1 = 0 \Leftrightarrow \left[ \begin{array}{l}{\left( {\dfrac{2}{3}} \right)^x} = \dfrac{{ - 1 + \sqrt 5 }}{2}\\{\left( {\dfrac{2}{3}} \right)^x} = \dfrac{{ - 1 - \sqrt 5 }}{2} < 0(loai)\end{array} \right. \Rightarrow \dfrac{a}{b} = \dfrac{{ - 1 + \sqrt 5 }}{2}\)

Điều kiện: \(\left\{ \begin{array}{l}{x^2} - 4x + 3 > 0\\4x - 4 > 0\end{array} \right. \Leftrightarrow x > 3.\)

\({\log _2}\left( {{x^2} - 4x + 3} \right) = {\log _2}\left( {4x - 4} \right) \Leftrightarrow {x^2} - 4x + 3 = 4x - 4 \Leftrightarrow \left[ \begin{array}{l}x = 1(l)\\x = 7\end{array} \right.\) .

Vậy \(S = \left\{ 7 \right\}\) .

Điều kiện $\left\{ {\begin{array}{*{20}{c}}{x + 1 > 0}\\{x - 3 > 0}\end{array}} \right. \Leftrightarrow x > 3$

Ta có

$\begin{array}{l}{\log _4}\left( {x + 1} \right) + {\log _4}\left( {x - 3} \right) = 3 \Leftrightarrow {\log _4}\left( {x + 1} \right)\left( {x - 3} \right) = 3\\ \Leftrightarrow \left( {x + 1} \right)\left( {x - 3} \right) = {4^3} \Leftrightarrow {x^2} - 2{\rm{x}} - 67 = 0 \Leftrightarrow x = 1 \pm 2\sqrt {17} \end{array}$

So sánh với điều kiện nghiệm của pt là $x = 1 + 2\sqrt {17} $

Điều kiện:  \(x >  - \dfrac{{{3^{50}}}}{2}\)

Phương trình đã cho tương đương với:

\(\begin{array}{l}{\log _3}\left( {{9^{50}} + 6{x^2}} \right) = {\log _3}\left( {{9^{50}} + 4x{{.3}^{50}} + 4{x^2}} \right)\\ \Leftrightarrow 6{x^2} = 4x{.3^{50}} + 4{x^2} \Leftrightarrow {x^2} = 2x{.3^{50}} \Leftrightarrow \left[ \begin{array}{l}x = 0\\x = {2.3^{50}}\end{array} \right.\end{array}\)

Phương trình đã cho tương đương với:

$\begin{array}{l}{\log _2}({2^x} - 1)[\log _{4}2 + \log _{4}({2^x} - 1)] = 1 \Leftrightarrow {\log _2}\left( {{2^x} - 1} \right)\left[ {\dfrac{1}{2} + \dfrac{1}{2}{{\log }_2}\left( {{2^x} - 1} \right)} \right] = 1\\ \Leftrightarrow {\log _2}\left( {{2^x} - 1} \right)\left[ {1 + {{\log }_2}\left( {{2^x} - 1} \right)} \right] = 2 \Leftrightarrow \log _2^2\left( {{2^x} - 1} \right) + {\log _2}\left( {{2^x} - 1} \right) - 2 = 0\\ \Leftrightarrow \left[ \begin{array}{l}{\log _2}\left( {{2^x} - 1} \right) = 1\\{\log _2}\left( {{2^x} - 1} \right) =  - 2\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}{2^x} - 1 = 2\\{2^x} - 1 = \dfrac{1}{4}\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}{2^x} = 3\\{2^x} = \dfrac{5}{4}\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}x = {\log _2}3\\x = {\log _2}\dfrac{5}{4}\end{array} \right.\end{array}$

\({\log _4}\left( {{{3.2}^x} - 1} \right) = x - 1 \Leftrightarrow {3.2^x} - 1 = {4^{x - 1}} \Leftrightarrow {4^x} - {12.2^x} + 4 = 0\)

Đặt \(t = {2^x}\) khi đó phương trình trở thành \({t^2} - 12t + 4 = 0\) , phương trình có hai nghiệm \({t_1},{t_2}\) thỏa mãn \({t_1}{t_2} = 4 \Leftrightarrow {2^{{x_1}}}{.2^{{x_2}}} = 4 \Leftrightarrow {2^{{x_1} + {x_2}}} = {2^2} \Leftrightarrow {x_1} + {x_2} = 2\)

Điều kiện \(x > 0\)

Ta đặt \({\log _3}x = u;{\log _5}x = v \Rightarrow u.v = u + v\)

Khi đó \(x = {3^u} = {5^v}\) suy ra \({\log _3}{3^u} = {\log _3}{5^v} \Leftrightarrow u = v{\log _3}5\)

\( \Rightarrow uv = u + v \Leftrightarrow {v^2}{\log _3}5 = v{\log _3}5 + v\) \( \Leftrightarrow {v^2}{\log _3}5 - v\left( {{{\log }_3}5 + 1} \right) = 0\) \( \Leftrightarrow v\left( {v{{\log }_3}5 - {{\log }_3}5 - 1} \right) = 0\)

\( \Leftrightarrow \left[ \begin{array}{l}v = 0\\v{\log _3}5 - {\log _3}5 - 1 = 0\end{array} \right.\) \( \Leftrightarrow \left[ \begin{array}{l}v = 0\\v = \dfrac{{{{\log }_3}5 + 1}}{{{{\log }_3}5}} = 1 + \dfrac{1}{{{{\log }_3}5}}\end{array} \right.\)   

\( \Rightarrow \left[ \begin{array}{l}u = 0\\u = 1 + {\log _3}5\end{array} \right. \Rightarrow \left[ \begin{array}{l}x = 1\left( {TM} \right)\\x = {3^{1 + {{\log }_3}5}} = 15\left( {TM} \right)\end{array} \right.\)

Do đó phương trình có hai nghiệm \({x_1} = 1,{x_2} = 15\) và tổng hai nghiệm bằng \(16\) là một số chính phương.

TXĐ : \(D = R\).

\(2{\log _2}\left| x \right| + {\log _2}\left| {x + 3} \right| = m \Leftrightarrow {\log _2}{\left| x \right|^2} + {\log _2}\left| {x + 3} \right| = m \)

$\Leftrightarrow {\log _2}\left( {{{\left| x \right|}^2}.\left| {x + 3} \right|} \right) = m \Leftrightarrow {\left| x \right|^2}.\left| {x + 3} \right| = {2^m}$

\( \Leftrightarrow {x^2}.\left| {x + 3} \right| = {2^m}\).

Xét hàm \(f(x) = {x^2}.\left| {x + 3} \right|\). Ta có : \(f(x) = {x^2}.\left| {x + 3} \right| = \left| {{x^3} + 3{x^2}} \right|\)

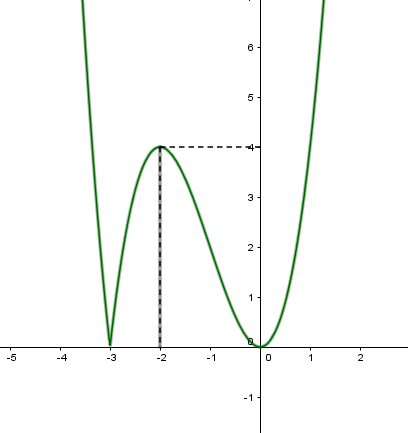
Để phương trình có 3 nghiệm phân biệt thì \({2^m} = 4 \Leftrightarrow m = 2\)

\(4\log _a^2x - 8{\log _b}x.{\log _a}x + 3\log _b^2x = 0\)

Ta có: \(\Delta ' = {(4{\log _b}x)^2} - 3.4.{\log _b}x = 4\log _b^2x > 0 \Rightarrow \left[ \begin{array}{l}{\log _a}x = \dfrac{3}{2}{\log _b}x\\{\log _a}x = \dfrac{1}{2}{\log _b}x\end{array} \right.\).

Suy ra

\({\log _a}x = \dfrac{3}{2}{\log _b}x \Rightarrow {\log _a}x = {\log _{\sqrt[3]{{{b^2}}}}}x \Rightarrow a = \sqrt[3]{{{b^2}}} \Rightarrow {a^3} = {b^2}\)

\({\log _a}x = \dfrac{1}{2}{\log _b}x \Rightarrow {\log _a}x = {\log _{{b^2}}}x \Rightarrow a = {b^2}\)

\( \begin{array}{l} VT= {\log _x}2 + {\log _x}3 + {\log _x}4 + ... + {\log _x}2017 = {\log _x}(2.3.4...2017)\\ \Rightarrow {x^M} = 2017! \Rightarrow x = \sqrt[M]{{2017!}}\end{array}\)

Điều kiện: \(x > 0;x \ne 1\)

${\log _3}x + \dfrac{1}{{{{\log }_9}x}} = 3 \Leftrightarrow {\log _3}x + \dfrac{2}{{{{\log }_3}x}} = 3 \Leftrightarrow {\left( {{{\log }_3}x} \right)^2} - 3{\log _3}x + 2 = 0$

\( \Leftrightarrow \left[ \begin{array}{l}{\log _3}x = 1\\{\log _3}x = 2\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}x = 3\\x = {3^2} = 9\end{array} \right.\)

Phương trình đã cho tương đương với $\left\{ \begin{array}{l}{\log _2}\left( {\dfrac{x}{{x - 2}}} \right) = m\\x > 2\end{array} \right.$

Để phương trình đã cho có nghiệm thì đường thẳng $y = m $ cắt đồ thị hàm số $y = {\log _2}f\left( x \right)$ với $f\left( x \right) = \dfrac{x}{{x - 2}}$ trên khoảng $\left( {2; + \infty } \right)$

Có $f'\left( x \right) =  - \dfrac{2}{{{{\left( {x - 2} \right)}^2}}} < 0, ∀x > 2$ và $\mathop {\lim }\limits_{x \to {2^ + }} f\left( x \right) =  + \infty ;\mathop {\lim }\limits_{x \to  + \infty } f\left( x \right) = 1$ nên ta có các tập giá trị của các hàm số là $f\left( x \right) \in \left( {1; + \infty } \right) \Rightarrow {\log _2}f\left( x \right) \in \left( {0; + \infty } \right)$

Vậy $0 < m < +∞$.

\({\log _3}\left( {7 - {3^x}} \right) = 2 - x\)

Điều kiện: \(7 - {3^x} > 0\)

\(pt \Leftrightarrow 7 - {3^x} = {3^{2 - x}} \Leftrightarrow 7 - {3^x} = \dfrac{9}{{{3^x}}} \Leftrightarrow {7.3^x} - {\left( {{3^x}} \right)^2} = 9\, \Leftrightarrow {3^{2x}} - {7.3^x} + 9 = 0\,\,\left( * \right)\)

Đặt \(t = {3^x}\;\;\left( {t > 0} \right) \Rightarrow x = {\log _3}t\) . Thay vào phương trình (*) ta có:

\( \Leftrightarrow {t^2} - 7t + 9 = 0\,\,\,\,\left( {**} \right)\)

Nhận thấy (**) có: \(\Delta  = 13 > 0,\;\;S = 7 > 0,\;\;P = 9 > 0 \Rightarrow \)  phương trình (**) có 2 nghiệm dương phân biệt giả sử là: \({t_1};{t_2}\)

Áp dụng hệ thức Vi-et cho phương trình  (**)  ta được: \(\left\{ \begin{array}{l}{t_1} + {t_2} = 7\\{t_1}{t_2} = 9\end{array} \right.\)

Khi đó ta có: \({x_1} + {x_2} = {\log _3}{t_1} + {\log _3}{t_2} = {\log _3}\left( {{t_1}{t_2}} \right) = {\log _3}9 = 2\)

Ta có: \({\log _2}\left( {2x + 2} \right) + x - 3y = {8^y} \Leftrightarrow {\log _2}\left( {x + 1} \right) + x + 1 = {2^{3y}} + 3y\) (*)

Xét hàm số \(y = f\left( x \right) = {2^x} + x\) có \(f'\left( x \right) = {2^x}\ln 2 + 1 > 0\,\,\forall x \in \mathbb{R}\) \( \Rightarrow \) Hàm số đồng biến trên \(\mathbb{R}.\)

\( \Rightarrow \) Phương trình (*)\( \Leftrightarrow f\left( {{{\log }_2}\left( {x + 1} \right)} \right) = f\left( {3y} \right)\)\( \Leftrightarrow {\log _2}\left( {x + 1} \right) = 3y\)

Do \(0 \le x \le 2020\) nên \(0 \le {\log _2}\left( {x + 1} \right) \le {\log _2}2021 \Rightarrow 0 \le 3y \le {\log _2}2021\)

                             \( \Leftrightarrow 0 \le y \le \dfrac{{{{\log }_2}2021}}{3} \Rightarrow y \in \left\{ {0;1;2;3} \right\}\)

Với mỗi giá trị y vừa tìm được đều tìm được đúng 1 giá trị x nguyên thỏa mãn

\( \Rightarrow \) Có 4 cặp số \(\left( {x;y} \right)\) nguyên thỏa mãn các điều kiện trên.

$({x^2} - 4)({\log _2}x + {\log _3}x + {\log _4}x + ... + {\log _{19}}x - \log _{20}^2x) = 0(*)$

Đkxđ: $x>0$

$(*) \Leftrightarrow \left[ \begin{array}{l}x = 2(tm)\\x =  - 2(ktm)\\{\log _2}x + {\log _3}x + {\log _4}x + ... + {\log _{19}}x - \log _{20}^2x = 0(**)\end{array} \right.$

$\begin{array}{l}(**) \Leftrightarrow \dfrac{{\log {\rm{x}}}}{{\log 2}} + \dfrac{{\log {\rm{x}}}}{{\log 3}} + \dfrac{{\log {\rm{x}}}}{{\log 4}} + ... + \dfrac{{\log {\rm{x}}}}{{\log 19}} - {\left( {\dfrac{{\log {\rm{x}}}}{{\log 20}}} \right)^2} = 0\\ \Leftrightarrow \log {\rm{x}}(\dfrac{1}{{\log 2}} + \dfrac{1}{{\log 3}} + \dfrac{1}{{\log 4}} + ... + \dfrac{1}{{\log 19}} - \dfrac{{\log {\rm{x}}}}{{{{\log }^2}20}}) = 0\\ \Leftrightarrow \left[ \begin{array}{l}\log {\rm{x}} = 0\\\dfrac{1}{{\log 2}} + \dfrac{1}{{\log 3}} + \dfrac{1}{{\log 4}} + ... + \dfrac{1}{{\log 19}} - \dfrac{{\log {\rm{x}}}}{{{{\log }^2}20}} = 0\end{array} \right. \\ \Leftrightarrow \left[ \begin{array}{l}x = 1\\\dfrac{1}{{\log 2}} + \dfrac{1}{{\log 3}} + \dfrac{1}{{\log 4}} + ... + \dfrac{1}{{\log 19}} = \dfrac{{\log {\rm{x}}}}{{{{\log }^2}20}}\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}x = 1\\(\dfrac{1}{{\log 2}} + \dfrac{1}{{\log 3}} + \dfrac{1}{{\log 4}} + ... + \dfrac{1}{{\log 19}}){\log ^2}20 = \log {\rm{x}}\end{array} \right. \\ \Leftrightarrow \left[ \begin{array}{l}x = 1(tm)\\x = {10^{(\dfrac{1}{{\log 2}} + \dfrac{1}{{\log 3}} + \dfrac{1}{{\log 4}} + ... + \dfrac{1}{{\log 19}}){{\log }^2}20}}(tm)\end{array} \right.\end{array}$ 

Phương trình (*) có $3$ nghiệm.

ĐKXĐ: \(\cos x > 0\)

Ta có: \(f\left( x \right) = {\log _2}\left( {\cos x} \right) \Rightarrow f'\left( x \right) = \dfrac{{ - \sin x}}{{\cos x.\ln 2}}\)

\(f'\left( x \right) = 0 \Leftrightarrow \)\(\dfrac{{ - \sin x}}{{\cos x.\ln 2}} = 0 \Leftrightarrow \tan x = 0 \Leftrightarrow x = k\pi ,\,k \in \mathbb{Z}\).

Với \(k\) chẵn, đặt \(k = 2m\,\,\left( {m \in \mathbb{Z}} \right)\), khi đó ta có \(x = m2\pi \,\,\left( {m \in \mathbb{Z}} \right)\).

Với \(k\) lẻ, đặt \(k = 2n + 1\,\,\left( {n \in \mathbb{Z}} \right)\), khi đó ta có \(x = \left( {2n + 1} \right)\pi  = \pi  + n2\pi \,\,\left( {n \in \mathbb{Z}} \right)\).

Kiểm tra ĐKXĐ:

\(x = m2\pi  \Rightarrow \cos x = 1 > 0\): thỏa mãn.

\(x = \pi  + k2\pi  \Rightarrow \cos x =  - 1 < 0\): loại.

Suy ra nghiệm của phương trình là \(x = m2\pi ,\,\,m \in \mathbb{Z}\).

Theo bài ra ta có: \(x \in \left( {0;2020\pi } \right) \Rightarrow 0 < m2\pi  < 2020\pi  \Leftrightarrow 0 < m < 1010 \Rightarrow \) Có 1009 giá trị nguyên của m thỏa mãn.

Vậy phương trình \(f'\left( x \right) = 0\) có 1009 nghiệm trong khoảng \(\left( {0;2020\pi } \right)\).

\(\dfrac{1}{{\ln \left( {x + 5} \right)}} + \dfrac{1}{{{3^x} - 1}} = x + a \Leftrightarrow f\left( x \right) = \dfrac{1}{{\ln \left( {x + 5} \right)}} + \dfrac{1}{{{3^x} - 1}} - x = a\,\,\left( * \right)\).

Xét hàm số \(f\left( x \right) = \dfrac{1}{{\ln \left( {x + 5} \right)}} + \dfrac{1}{{{3^x} - 1}} - x\).

ĐKXĐ: \(\left\{ \begin{array}{l}x + 5 > 0\\\ln \left( {x + 5} \right) \ne 0\\{3^x} - 1 \ne 0\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x >  - 5\\x + 5 \ne 1\\{3^x} \ne 1\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x >  - 5\\x \ne  - 4\\x \ne 0\end{array} \right.\)

\( \Rightarrow D = \left( { - 5; - 4} \right) \cup \left( { - 4;0} \right) \cup \left( {0; + \infty } \right)\).

Ta có:

$f'\left( x \right) = - \frac{1}{{\left( {x + 5} \right){{\ln }^2}\left( {x + 5} \right)}} - \frac{{{3^x}\ln 3}}{{{{\left( {{3^x} - 1} \right)}^2}}} - 1 < 0,\forall x \in D$

BBT:

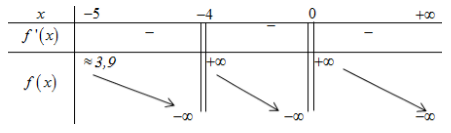
Từ BBT suy ra phương trình (*) có 2 nghiệm \( \Leftrightarrow a \ge 4\).

Kết hợp ĐK \( \Rightarrow a \in \left\{ {4;...;2018} \right\}\). Vậy có 2015 giá trị của \(a\) thỏa mãn.

Ta có: $\int\limits_0^2 {\left( {t - {{\log }_2}x} \right)dt}  = \left. {\left( {\dfrac{{{t^2}}}{2} - {{\log }_2}x.t} \right)} \right|_0^2 = 2 - 2{\log _2}x$

Phương trình: $2 - 2{\log _2}x = 2{\log _2}\dfrac{2}{x}$ có điều kiện là $x > 0$

$ \Leftrightarrow {\log _2}\dfrac{2}{x} + {\log _2}x = 1 \Leftrightarrow {\log _2}\left( {\dfrac{2}{x}.x} \right) = 1$ (luôn đúng)

Vậy tập nghiệm của phương trình là $(0; +\infty )$

Điều kiện : \(\left\{ \begin{array}{l}\cot x > 0\\\cos x > 0\end{array} \right.(1)\).

Ta có : $2{\log _3}\left( {\cot x} \right) = {\log _2}\left( {\cos x} \right) \Leftrightarrow {\log _3}{\left( {\cot x} \right)^2} = {\log _2}\left( {\cos x} \right) = t$

$ \Rightarrow \left\{ \begin{array}{l}{\left( {\cot x} \right)^2} = {3^t}\\{\cos ^2}x = {4^t}\end{array} \right. \Rightarrow \left\{ \begin{array}{l}\dfrac{{{{\cos }^2}x}}{{{{\sin }^2}x}} = {3^t}\\{\cos ^2}x = {4^t}\end{array} \right.$

\( \Rightarrow \dfrac{{{4^t}}}{{1 - {4^t}}} = {3^t} \Leftrightarrow {4^t} - {3^t} + {12^t} = 0 \Leftrightarrow {\left( {\dfrac{4}{3}} \right)^t} + {4^t} = 1\)

Đặt \(f(t) = {\left( {\dfrac{4}{3}} \right)^t} + {\left( 4 \right)^t} \Rightarrow f'(t) = {\left( {\dfrac{4}{3}} \right)^t}\ln \dfrac{4}{3} + {\left( 4 \right)^t}\ln 4 > 0\) suy ra $f(t)= 1$ có tối đa $1$ nghiệm.

Nhận thấy $t=-1$ là nghiệm của phương trình \( \Rightarrow {\log _2}\left( {\cos x} \right) =  - 1 \Rightarrow \cos x = \dfrac{1}{2} \Rightarrow x =  \pm \dfrac{\pi }{3} + k2\pi  \Rightarrow x = \dfrac{\pi }{3} + k2\pi \)( do đk (1)).

Ta có : \(0 < \dfrac{\pi }{3} + k2\pi  < 2017\pi  \Leftrightarrow  - \dfrac{1}{6} < k < \dfrac{{3025}}{3}\). Do $k$ nguyên nên $k= 0, 1, …, 1008$.

Vậy phương trình có $1009$ nghiệm.

ĐK: $x>-1;mx>0$

$\begin{array}{l}\log (m{\rm{x}}) = 2\log (x + 1) \Leftrightarrow m{\rm{x}} = {(x + 1)^2} \Leftrightarrow {x^2} + (2 - m)x + 1 = 0\\\Delta  = {m^2} - 4m + 4 - 4 = {m^2} - 4m\end{array}$

Để phương trình đã cho có nghiệm duy nhất thì có 2 TH:

TH1: Phương trình trên có nghiệm duy nhất: ${m^2} = 4m \Leftrightarrow \left[ \begin{array}{l}m = 0\\m = 4\end{array} \right..$

Tuy nhiên giá trị $m = 0$ loại do khi đó nghiệm là $x = -1$.

TH2: Phương trình trên có 2 nghiệm thỏa: ${x_1} \le  - 1 < {x_2}$

Nếu có ${x_1} =  - 1 \to 1 - (2 - m) + 1 = 0 \to m = 0$, thay lại vô lý

$\begin{array}{l}{x_1} <  - 1 < {x_2} \to ({x_1} + 1)({x_2} + 1) < 0 \Leftrightarrow {x_1}{x_2} + {x_1} + {x_2} + 1 < 0\\ \to 1 + m - 2 + 1 < 0 \Leftrightarrow m < 0.\end{array}$

Như vậy sẽ có các giá trị $-2017; - 2016; …… -1$ và $4$.

Có $2018 $ giá trị.