Nguyên hàm

Ta có:

\(F\left( x \right) = \int {f\left( x \right)dx}  = \int {{e^{ - 2018x + 2017}}dx}  = \dfrac{1}{{ - 2018}}{e^{ - 2018x + 2017}} + C\)

Với \(x = 1\) thì \( - \dfrac{1}{{2018}}{e^{ - 1}} + C = e \Leftrightarrow C = e + \dfrac{1}{{2018}}{e^{ - 1}}\)

Vậy \(F\left( x \right) =  - \dfrac{1}{{2018}}{e^{ - 2018x + 2017}} + e + \dfrac{1}{{2018e}}\).

Vì \(F\left( x \right) = {x^2}\) là nguyên hàm của hàm số \(f\left( x \right){e^{4x}}\) nên:

\(\begin{array}{l}f\left( x \right){e^{4x}} = F'\left( x \right) = 2x\\ \Rightarrow f\left( x \right) = \dfrac{{2x}}{{{e^{4x}}}}\end{array}\)

\(\begin{array}{l} \Rightarrow f'\left( x \right) = \dfrac{{2{e^{4x}} - 8x.{e^{4x}}}}{{{{\left( {{e^{4x}}} \right)}^2}}} = \dfrac{{2 - 8x}}{{{e^{4x}}}}\\ \Rightarrow f'\left( x \right){e^{4x}} = 2 - 8x\\ \Rightarrow \int {f'\left( x \right){e^{4x}}dx = \int {\left( {2 - 8x} \right)dx =  - 4{x^2} + 2x + C} } \end{array}\)

Bước 1:

Vì \(F\left( x \right)\) là 1 nguyên hàm của hàm số \(f\left( x \right)\) nên ta có \(F'\left( x \right) = f\left( x \right)\).

\(\begin{array}{l}F'\left( x \right) = \left( {2ax + b} \right){e^x} + \left( {a{x^2} + bx + c} \right){e^x}\\F'\left( x \right) = \left( {a{x^2} + bx + c + 2ax + b} \right){e^x}\\F'\left( x \right) = \left[ {a{x^2} + \left( {2a + b} \right)x + b + c} \right]{e^x}\\=x^2.e^x\end{array}\)

Bước 2:

Ta có:

\(\begin{array}{l}{x^2} =  {1.{x^2} + 0.x + 0}\\\left[ {a.{x^2} + \left( {2a + b} \right)x + b + c} \right]{e^x} = {x^2}.{e^x}\\ \Leftrightarrow a.{x^2} + \left( {2a + b} \right)x + b + c = {x^2}\\ \Leftrightarrow a.{x^2} + \left( {2a + b} \right)x + b + c = 1.{x^2} + 0.x + 0\end{array}\)

Đồng nhất hệ số ta có: \(\left\{ \begin{array}{l}a = 1\\2a + b = 0\\b + c = 0\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}a = 1\\b =  - 2\\c = 2\end{array} \right.\).

Vậy \(P = abc = 1.\left( { - 2} \right).2 =  - 4.\)

Ta có: $F'\left( x \right) = 3{x^2} + 2x-1 \Rightarrow F\left( x \right) = \int {F'\left( x \right)dx}  = \int {\left( {3{x^2} + 2x-1} \right)dx}  = {x^3} + {x^2} - x + C$

Tại \(x = 0\) thì $y=2$ suy ra \(2 = C \Rightarrow F\left( x \right) = {x^3} + {x^2} - x + 2\) và tổng các hệ số của \(F\left( x \right)\) là \(3\).

Theo bài ra ta có: \(f'\left( x \right) = {x^3}{f^2}\left( x \right)\,\,\forall x \in \mathbb{R} \Leftrightarrow \dfrac{{f'\left( x \right)}}{{{f^2}\left( x \right)}} = {x^3}\,\,\forall x \in \mathbb{R}\).

Lấy nguyên hàm hai vế ta có: \(\int {\dfrac{{f'\left( x \right)}}{{{f^2}\left( x \right)}}dx}  = \int {{x^3}dx} \) \( \Leftrightarrow  - \dfrac{1}{{f\left( x \right)}} = \dfrac{{{x^4}}}{4} + C\).

Lại có: \(f\left( 2 \right) =  - \dfrac{4}{{19}}\)\( \Leftrightarrow  - \dfrac{1}{{f\left( 2 \right)}} = 4 + C \Leftrightarrow \dfrac{{19}}{4} = 4 + C\) \( \Leftrightarrow C = \dfrac{3}{4}\).

Do đó \( - \dfrac{1}{{f\left( x \right)}} = \dfrac{{{x^4}}}{4} + \dfrac{3}{4}\).

Thay \(x = 1\) ta có \( - \dfrac{1}{{f\left( 1 \right)}} = \dfrac{1}{4} + \dfrac{3}{4} = 1\). Vậy \(f\left( 1 \right) =  - 1\).

\(\dfrac{{2x + 3}}{{2{x^2} - x - 1}} = \dfrac{{2x + 3}}{{\left( {2x + 1} \right)\left( {x - 1} \right)}}\)

Do đó, ta cần biến đổi \(\dfrac{{2x + 3}}{{2{x^2} - x - 1}} = \dfrac{a}{{2x + 1}} + \dfrac{b}{{x - 1}}\) để tính được nguyên hàm.

Ta có:

\(\begin{array}{l}\dfrac{a}{{2x + 1}} + \dfrac{b}{{x - 1}} = \dfrac{{a\left( {x - 1} \right) + b\left( {2x + 1} \right)}}{{\left( {2x + 1} \right)\left( {x - 1} \right)}}\\ = \dfrac{{ax - a + 2bx + b}}{{\left( {2x + 1} \right)\left( {x - 1} \right)}} = \dfrac{{\left( {a + 2b} \right)x - a + b}}{{\left( {2x + 1} \right)\left( {x - 1} \right)}}\end{array}\)

\(\begin{array}{l} \Rightarrow \dfrac{{2x + 3}}{{2{x^2} - x - 1}} = \dfrac{{\left( {a + 2b} \right)x - a + b}}{{\left( {2x + 1} \right)\left( {x - 1} \right)}}\\ \Leftrightarrow \left\{ \begin{array}{l}a + 2b = 2\\ - a + b = 3\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}a =  - \dfrac{4}{3}\\b = \dfrac{5}{3}\end{array} \right.\end{array}\)

 Do đó:

\(\int {\dfrac{{2x + 3}}{{2{x^2} - x - 1}}dx} {\rm{\;}}\)\( = \int {\left[ { - \dfrac{4}{3}.\dfrac{1}{{\left( {2x + 1} \right)}} + \dfrac{5}{3}.\dfrac{1}{{\left( {x - 1} \right)}}} \right]dx} {\rm{\;}}\)\( = {\rm{\;}} - \dfrac{4}{3}\int {\dfrac{1}{{\left( {2x + 1} \right)}}dx} {\rm{\;}} + \dfrac{5}{3}\int {\dfrac{1}{{\left( {x - 1} \right)}}dx} \)\( = {\rm{\;}} - \dfrac{4}{3}.\dfrac{1}{2}\ln \left| {2x + 1} \right| + \dfrac{5}{3}\ln \left| {x - 1} \right| + C\)\( = {\rm{\;}} - \dfrac{2}{3}\ln \left| {2x + 1} \right| + \dfrac{5}{3}\ln \left| {x - 1} \right| + C\)

Đáp án B: $\dfrac{{{x^2} - x - 1}}{{x + 1}}=\dfrac{{{x^2} -( x + 1)}}{{x + 1}}$$=\dfrac{{{x^2}}}{{x + 1}}-1$

Đáp án C: $\dfrac{{{x^2} + x + 1}}{{x + 1}}=\dfrac{{{x^2} +( x + 1)}}{{x + 1}}$$=\dfrac{{{x^2}}}{{x + 1}}+1$

Đáp án D: $\dfrac{{{x^2}}}{{x + 1}}$

Như thế, các hàm số ở ý B, C, D hơn kém nhau một số đơn vị do nên chúng là nguyên hàm của cùng một hàm số.

Ta có: \( N(t)=\int {N'(t)dt} = \int {\dfrac{{4000}}{{0,5t + 1}}dt}  \)\(= \dfrac{{4000}}{{0,5}}\ln \left| {0,5t + 1} \right| + C = 8000\ln \left| {0,5t + 1} \right| + C\).

Với \(t = 0\) thì \(250000 = 8000\ln 1 + C \)\(\Leftrightarrow C = 250000\).

Vậy \(N\left( t \right) = 8000\ln \left| {0,5t + 1} \right| + 250000 \)\(\Rightarrow N\left( {10} \right) \approx 264334\) 

Ta có $f'\left( x \right) = \dfrac{{x.f\left( x \right)}}{{\sqrt {{x^2} + 1} }} \Leftrightarrow \dfrac{{f'\left( x \right)}}{{f\left( x \right)}} = \dfrac{x}{{\sqrt {{x^2} + 1} }}$$ \Leftrightarrow \int {\dfrac{{f'\left( x \right)}}{{f\left( x \right)}}{\rm{d}}x}  = \int {\dfrac{x}{{\sqrt {{x^2} + 1} }}{\rm{d}}x} $

$ \Leftrightarrow \int {\dfrac{{{\rm{d}}\left( {f\left( x \right)} \right)}}{{f\left( x \right)}}}  = \int {\dfrac{{{\rm{d}}\left( {{x^2} + 1} \right)}}{{2\sqrt {{x^2} + 1} }}}  = \sqrt {{x^2} + 1}  + C$$ \Leftrightarrow \ln f\left( x \right) = \sqrt {{x^2} + 1}  + C \Leftrightarrow f\left( x \right) = {e^{\sqrt {{x^2}{\kern 1pt}  + {\kern 1pt} 1} {\kern 1pt}  + {\kern 1pt} {\kern 1pt} C}}$

Mà $f\left( 0 \right) = e$$ \Rightarrow $${e^{C{\kern 1pt}  + {\kern 1pt} 1}} = e \Rightarrow C = 0.$

Vậy $f\left( {\sqrt 3 } \right) = {e^2}.$

Ta có: \(f\left( x \right).f'\left( x \right) = \left( {2x + 1} \right)\sqrt {1 + {f^2}\left( x \right)} \)

\( \Rightarrow \dfrac{{f\left( x \right).f'\left( x \right)}}{{\sqrt {1 + {f^2}\left( x \right)} }} = 2x + 1 \Rightarrow \int {\dfrac{{f\left( x \right).f'\left( x \right)}}{{\sqrt {1 + {f^2}\left( x \right)} }}dx}  = \int {\left( {2x + 1} \right)dx} \)

Tính \(\int {\dfrac{{f\left( x \right).f'\left( x \right)}}{{\sqrt {1 + {f^2}\left( x \right)} }}dx} \) ta đặt \(\sqrt {1 + {f^2}\left( x \right)}  = t \Rightarrow 1 + {f^2}\left( x \right) = {t^2} \Rightarrow 2f\left( x \right)f'\left( x \right)dx = 2tdt\) \( \Rightarrow f\left( x \right)f'\left( x \right)dx = tdt\)

Thay vào ta được \(\int {\dfrac{{f\left( x \right).f'\left( x \right)}}{{\sqrt {1 + {f^2}\left( x \right)} }}dx}  = \int {\dfrac{{tdt}}{t}}  = \int {dt}  = t + C = \sqrt {1 + {f^2}\left( x \right)}  + C\)

Do đó \(\sqrt {1 + {f^2}\left( x \right)}  + C = {x^2} + x\).

\(f\left( 0 \right) = 2\sqrt 2  \Rightarrow \sqrt {1 + {{\left( {2\sqrt 2 } \right)}^2}}  + C = 0 \Leftrightarrow C =  - 3\).

Từ đó:

\(\begin{array}{l}\sqrt {1 + {f^2}\left( x \right)}  - 3 = {x^2} + x \Rightarrow \sqrt {1 + {f^2}\left( 1 \right)}  - 3 = 1 + 1 \Leftrightarrow \sqrt {1 + {f^2}\left( 1 \right)}  = 5\\ \Leftrightarrow 1 + {f^2}\left( 1 \right) = 25 \Leftrightarrow {f^2}\left( 1 \right) = 24 \Leftrightarrow f\left( 1 \right) = \sqrt {24} \end{array}\)

Ta có:

\(\begin{array}{l}F'\left( x \right) = f\left( x \right)\\ \Leftrightarrow f'\left( x \right) - {e^x} - 1 = f\left( x \right)\\ \Leftrightarrow f'\left( x \right) - f\left( x \right) = {e^x} + 1\\ \Leftrightarrow {e^{ - x}}.f'\left( x \right) - {e^{ - x}}.f\left( x \right) = 1 + {e^{ - x}}\\ \Leftrightarrow \left[ {{e^{ - x}}.f\left( x \right)} \right]' = 1 + {e^{ - x}}\\ \Leftrightarrow \int {\left[ {{e^{ - x}}.f\left( x \right)} \right]'dx}  = \int {\left( {1 + {e^{ - x}}} \right)dx} \\ \Leftrightarrow {e^{ - x}}.f\left( x \right) = x - {e^{ - x}} + C\\ \Leftrightarrow f\left( x \right) = x.{e^x} - 1 + C.{e^x}\end{array}\)

Thay \(x = 0\) ta có: \(f\left( 0 \right) =  - 1 + C = 1 \Leftrightarrow C = 2\).

\(\begin{array}{l} \Rightarrow f\left( x \right) = x.{e^x} - 1 + 2{e^x} = \left( {x + 2} \right){e^x} - 1\\ \Rightarrow \int {f\left( x \right)dx}  = \int {\left[ {\left( {x + 2} \right){e^x} - 1} \right]dx} \\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \int {\left( {x + 2} \right){e^x}dx}  - \int {dx} \\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \left( {x + 2} \right){e^x} - \int {{e^x}dx}  - x + C\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \left( {x + 2} \right){e^x} - {e^x} - x + C\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \left( {x + 1} \right){e^x} - x + C\end{array}\)

\(\int {\left( {{x^2} + 4} \right)dx}  = \dfrac{{{x^3}}}{3} + 4x + C\).

Ta có \(\int {\left( {{e^x} + 2} \right)dx}  = {e^x} + 2x + C\).

Ta có \(f\left( x \right) = \dfrac{{{x^2} - 2x + 1}}{{x - 2}} = x + \dfrac{1}{{x - 2}}\).

\( \Rightarrow \int {f\left( x \right)dx}  = \int {\left( {x + \dfrac{1}{{x - 2}}} \right)dx} \)$=\int xdx + \int { \dfrac{1}{{x - 2}}dx  }  $\( = \dfrac{{{x^2}}}{2} + \ln \left| {x - 2} \right| + C\).

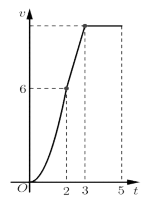
Trong 2 giây đầu, \({v_1} = a{t^2}\), lại có khi \(t = 2\,\,\left( s \right) \Rightarrow {v_1} = 60\,\,\left( {m/s} \right)\) nên \(60 = a{.2^2} \Leftrightarrow a = 15\), suy ra \({v_1} = 15{t^2}\).

Quãng đường vật đi được trong 2 giây đầu là \({s_1} = \int\limits_0^2 {{v_1}\left( t \right)dt}  = \int\limits_0^2 {15{t^2}dt}  = 40\,\,\left( m \right)\).

Trong giây tiếp theo, \({v_2} = mt + n\).

Ta có \(\left\{ \begin{array}{l}t = 2 \Rightarrow v = 60\\t = 3 \Rightarrow v = 360km/h = 100m/s\end{array} \right.\), nên ta có hệ phương trình \(\left\{ \begin{array}{l}2m + n = 60\\3m + n = 100\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}m = 40\\n =  - 20\end{array} \right.\) \( \Rightarrow {v_2}\left( t \right) = 40t - 20\).

Quãng đường vật đi được trong giây tiếp theo là \({s_2} = \int\limits_2^3 {{v_2}\left( t \right)dt}  = \int\limits_2^3 {\left( {40t - 20} \right)dt}  = 80\,\,\left( m \right)\).

Trong 2 giây cuối, \({v_3} = 100\,\,\left( {m/s} \right)\).

Quãng đường vật đi được trong 2 giây cuối là \({s_3} = \int\limits_3^5 {{v_3}\left( t \right)dt}  = \int\limits_3^5 {100dt}  = 200\,\,\left( m \right)\).

Vậy trong 5 giây đó xe đã đi được quãng đường là: \(40 + 80 + 200 = 320\,\,\left( m \right)\).

Xét hàm số: \(f\left( x \right) = \dfrac{1}{{{x^2} - 2x}}\) trong \(\left( {2; + \infty } \right)\) ta có:

\( \dfrac{1}{{{x^2} - 2x}}=\dfrac{1}{{x\left( {x - 2} \right)}}=\dfrac{1}{{2\left( {x - 2} \right)}} - \dfrac{1}{{2x}} \)

Khi đó:

\(\begin{array}{l}\,\,\,\,\int {f\left( x \right)dx}  = \int {\dfrac{1}{{{x^2} - 2x}}dx} \\ = \int {\left( {\dfrac{1}{{2\left( {x - 2} \right)}} - \dfrac{1}{{2x}}} \right)dx} \\ = \dfrac{1}{2}\ln \left| {x - 2} \right| - \dfrac{1}{2}\ln \left| x \right| + C\\ = \dfrac{1}{2}\left( {\ln \left| {x - 2} \right| - \ln \left| x \right|} \right) + C.\end{array}\)

Vì \(x \in \left( {2; + \infty } \right) \Rightarrow \left\{ \begin{array}{l}\left| {x - 2} \right| = x - 2\\\left| x \right| = x\end{array} \right.\)

Do đó \(\int {f\left( x \right)dx}  = \dfrac{{\ln \left( {x - 2} \right) - \ln x}}{2} + C\).

Ta có \(f(x) = \int {{f^\prime }} (x)dx = \int {\left( {12{x^2} + 2} \right)} dx\) \( = 4{x^3} + 2x + C\)

Với \(f(1) = 3 \Rightarrow {4.1^3} + 2.1 + C = 3 \Rightarrow C =  - 3\)

Vậy \(f(x) = 4{x^3} + 2x - 3\)

Ta có \(F(x) = \int f (x)dx = \int {\left( {4{x^3} + 2x - 3} \right)} dx\) \( = {x^4} + {x^2} - 3x + C\)

Với \(F(0) = 2 \Rightarrow {0^4} + {0^2} - 3.0 + C = 2 \Rightarrow C = 2\)

Vậy \(F(x) = {x^4} + {x^2} - 3x + 2\)

khi đó \(F(1) = {1^4} + {1^2} - 3.1 + 2 = 1\)