Tổng hợp câu hay và khó chương 4 - Phần 1

Ta tính giới hạn của các dãy số trong từng đáp án:

+) Đáp án A:           

$\mathop {\lim }\limits_{} {u_n} = \mathop {\lim }\limits_{} \dfrac{{{{\left( {2017 - n} \right)}^{2018}}}}{{n{{\left( {2018 - n} \right)}^{2017}}}} = \mathop {\lim }\limits_{} \left[ {\dfrac{{2017 - n}}{n}.{{\left( {\dfrac{{2017 - n}}{{2018 - n}}} \right)}^{2017}}} \right]$

$= \mathop {\lim }\limits_{} \left[ {\left( {\dfrac{{2017}}{n} - 1} \right){{\left( {\dfrac{{\dfrac{{2017}}{n} - 1}}{{\dfrac{{2018}}{n} - 1}}} \right)}^{2017}}} \right] =  - 1$.

+) Đáp án B:

$\mathop {\lim }\limits_{} {u_n} = \mathop {\lim }\limits_{} n\left( {\sqrt {{n^2} + 2018}  - \sqrt {{n^2} + 2016} } \right) = \mathop {\lim }\limits_{} \dfrac{{n\left( {{n^2} + 2018 - {n^2} - 2016} \right)}}{{\sqrt {{n^2} + 2018}  + \sqrt {{n^2} + 2016} }}$

$= \mathop {\lim }\limits_{} \dfrac{{2n}}{{\sqrt {{n^2} + 2018}  + \sqrt {{n^2} + 2016} }} = \mathop {\lim }\limits_{} \dfrac{2}{{\sqrt {1 + \dfrac{{2018}}{{{n^2}}}}  + \sqrt {1 + \dfrac{{2016}}{{{n^2}}}} }} = 1$.

+) Đáp án C:           

Ta có ${u_{n + 1}} - 1 = \dfrac{1}{2}\left( {{u_n} - 1} \right)$

$\begin{array}{l}{u_n} - 1 = \dfrac{1}{2}\left( {{u_{n - 1}} - 1} \right)\\{u_{n - 1}} - 1 = \dfrac{1}{2}\left( {{u_{n - 2}} - 1} \right)\\{u_{n - 2}} - 1 = \dfrac{1}{2}\left( {{u_{n - 3}} - 1} \right)\\...\\{u_2} - 1 = \dfrac{1}{2}\left( {{u_1} - 1} \right)\end{array}$

$\Rightarrow \left( {{u_n} - 1} \right)\left( {{u_{n - 1}} - 1} \right)\left( {{u_{n - 2}} - 1} \right)...\left( {{u_2} - 1} \right)$ $= \dfrac{1}{2}\left( {{u_{n - 1}} - 1} \right).\dfrac{1}{2}\left( {{u_{n - 2}} - 1} \right).\dfrac{1}{2}\left( {{u_{n - 3}} - 1} \right)...\dfrac{1}{2}\left( {{u_1} - 1} \right)$

$= \dfrac{1}{{{2^{n - 1}}}}\left( {{u_{n - 1}} - 1} \right)\left( {{u_{n - 2}} - 1} \right)...\left( {{u_2} - 1} \right)\left( {{u_1} - 1} \right)$

$\Rightarrow {u_n} - 1 = \dfrac{1}{{{2^{n - 1}}}}\left( {{u_1} - 1} \right)$

$\Rightarrow {u_n} = \dfrac{{2016}}{{{2^{n - 1}}}} + 1 \Leftrightarrow {u_n} = 4032.{\left( {\dfrac{1}{2}} \right)^n} + 1$$\Rightarrow \mathop {\lim }\limits_{} {u_n} = 1$.

Ở câu C, các em cũng có thể giả sử $\lim {u_n} = a$ thì ${u_{n + 1}} \to a$ khi $n \to \infty$, do đó ta có $a = \dfrac{1}{2}\left( {a + 1} \right) \Leftrightarrow a = 1$

+) Đáp án D:

Ta có ${u_n} = \dfrac{1}{{1.2}} + \dfrac{1}{{2.3}} + \dfrac{1}{{3.4}} + ... + \dfrac{1}{{n\left( {n + 1} \right)}} = 1 - \dfrac{1}{2} + \dfrac{1}{2} - \dfrac{1}{3} + ... + \dfrac{1}{n} - \dfrac{1}{{n + 1}} = 1 - \dfrac{1}{{n + 1}} = \dfrac{n}{{n + 1}}$

$\Rightarrow \mathop {\lim }\limits_{} {u_n} = \mathop {\lim }\limits_{} \dfrac{n}{{n + 1}} = 1$.

Ta có $\mathop {\lim }\limits_{x \to 1} f\left( x \right) = \mathop {\lim }\limits_{x \to 1} \dfrac{{{x^{2016}} + x - 2}}{{\sqrt {2018x + 1}  - \sqrt {x + 2018} }}$ $= \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {{x^{2016}} + x - 2} \right)\left( {\sqrt {2018x + 1}  + \sqrt {x + 2018} } \right)}}{{2018x + 1 - x - 2018}}$

$= \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {x - 1} \right)\left( {{x^{2015}} + {x^{2014}} + ... + x + 2} \right)\left( {\sqrt {2018x + 1}  + \sqrt {x + 2018} } \right)}}{{2017\left( {x - 1} \right)}}$

$= \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {{x^{2015}} + {x^{2014}} + ... + x + 2} \right)\left( {\sqrt {2018x + 1}  + \sqrt {x + 2018} } \right)}}{{2017}} = 2\sqrt {2019}$

Mà $f\left( 1 \right) = k$

Suy ra hàm số liên tục tại $x = 1$ $\Leftrightarrow k = 2\sqrt {2019}$.

Vì hàm số có giới hạn hữu hạn tại $x = 1$ nên biểu thức tử nhận $x = 1$ làm nghiệm, hay $1 + a + b = 0$.

Áp dụng vào giả thiết, được $\mathop {\lim }\limits_{x \to 1} \dfrac{{{x^2} + ax - 1 - a}}{{{x^2} - 1}} = \dfrac{{ - 1}}{2} \Leftrightarrow \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {x - 1} \right)\left( {x + 1 + a} \right)}}{{\left( {x - 1} \right)\left( {x + 1} \right)}} =  - \dfrac{1}{2}$.

$\Leftrightarrow \mathop {\lim }\limits_{x \to 1} \dfrac{{x + 1 + a}}{{x + 1}} =  - \dfrac{1}{2} \Leftrightarrow \dfrac{{2 + a}}{2} =  - \dfrac{1}{2} \Leftrightarrow a =  - 3$.

Suy ra $b = 2$.

Vậy ${a^2} + {b^2} = 13$.

Bước 1:

Ta có: $f\left( { - 2} \right) =  - 11$, $\mathop {\lim }\limits_{x \to  - {2^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to  - {2^ - }} \left( {3x - 5} \right) =  - 11$, $\mathop {\lim }\limits_{x \to  - {2^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to  - {2^ - }} \left( {ax - 1} \right) =  - 2a - 1$.

Bước 2:

Để hàm số liên tục tại $x =  - 2$ thì $f\left( { - 2} \right) = \mathop {\lim }\limits_{x \to  - {2^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to  - {2^ + }} f\left( x \right)$

$\Leftrightarrow  - 2a - 1 =  - 11 \Leftrightarrow a = 5$.

Vậy hàm số liên tục tại $x =  - 2$ khi $a = 5$.

Cách 1 (Đặc biệt hóa)

Chọn $f\left( x \right) = 10x$, ta có $\mathop {\lim }\limits_{x \to 2} \dfrac{{f\left( x \right) - 20}}{{x - 2}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{10x - 20}}{{x - 2}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{10\left( {x - 2} \right)}}{{x - 2}} = 10$

Lúc đó $T = \mathop {\lim }\limits_{x \to 2} \dfrac{{\sqrt[3]{{6f\left( x \right) + 5}} - 5}}{{{x^2} + x - 6}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{\sqrt[3]{{60x + 5}} - 5}}{{{x^2} + x - 6}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{\sqrt[3]{{60x + 5}} - 5}}{{\left( {x - 2} \right)\left( {x + 3} \right)}}$

$= \mathop {\lim }\limits_{x \to 2} \dfrac{{60x + 5 - {5^3}}}{{\left( {x - 2} \right)\left( {x + 3} \right)\left( {{{\sqrt[3]{{60x + 5}}}^2} + 5\sqrt[3]{{60x + 5}} + 25} \right)}}$

$= \mathop {\lim }\limits_{x \to 2} \dfrac{{60\left( {x - 2} \right)}}{{\left( {x - 2} \right)\left( {x + 3} \right)\left( {{{\sqrt[3]{{60x + 5}}}^2} + 5\sqrt[3]{{60x + 5}} + 25} \right)}}$

$= \mathop {\lim }\limits_{x \to 2} \dfrac{{60}}{{\left( {x + 3} \right)\left( {{{\sqrt[3]{{60x + 5}}}^2} + 5\sqrt[3]{{60x + 5}} + 25} \right)}} = \dfrac{4}{{25}}$

Cách 2:

Chọn $f\left( x \right) = 10x$, ta có $\mathop {\lim }\limits_{x \to 2} \dfrac{{f\left( x \right) - 20}}{{x - 2}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{10x - 20}}{{x - 2}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{10\left( {x - 2} \right)}}{{x - 2}} = 10$

Sử dụng CASIO (chức năng CALC), nhập hàm cần tính giới hạn

Màn hình hiển thị

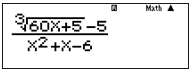
Thay giá trị $x = 1,9999999$ vào

Màn hình hiển thị

Thay tiếp giá trị $x = 2,0000001$ vào

Màn hình hiển thị

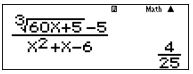
Cách 3:

Theo giả thiết có $\mathop {\lim }\limits_{x \to 2} \left( {f\left( x \right) - 20} \right) = 0$ hay $\mathop {\lim }\limits_{x \to 2} f\left( x \right) = 20$ $\left( * \right)$

Khi đó $T = \mathop {\lim }\limits_{x \to 2} \dfrac{{\sqrt[3]{{6f\left( x \right) + 5}} - 5}}{{{x^2} + x - 6}} = \mathop {\lim }\limits_{x \to 2} \dfrac{{6f\left( x \right) + 5 - 125}}{{\left( {{x^2} + x - 6} \right)\left[ {{{\left( {\sqrt[3]{{6f\left( x \right) + 5}}} \right)}^2} + 5\left( {\sqrt[3]{{6f\left( x \right) + 5}}} \right) + 25} \right]}}$

$T = \mathop {\lim }\limits_{x \to 2} \dfrac{{6\left[ {f\left( x \right) - 20} \right]}}{{\left( {x - 2} \right)\left( {x + 3} \right)\left[ {{{\left( {\sqrt[3]{{6f\left( x \right) + 5}}} \right)}^2} + 5\left( {\sqrt[3]{{6f\left( x \right) + 5}}} \right) + 25} \right]}}$

$\mathop {\lim }\limits_{x \to 2} \dfrac{{f\left( x \right) - 20}}{{x - 2}} = 10$

$\begin{array}{l}\mathop {\lim }\limits_{x \to 2} f\left( x \right) = 20\\ \Rightarrow \mathop {\lim }\limits_{x \to 2} \dfrac{6}{{\left( {x - 3} \right).\left[ {{{\left( {\sqrt[3]{{6f\left( x \right) + 5}}} \right)}^2} + 5\left( {\sqrt[3]{{6f\left( x \right) + 5}}} \right) + 25} \right]}}\\ = \dfrac{6}{{\left( {2 + 3} \right).\left[ {{{\left( {\sqrt[3]{{6.20 + 5}}} \right)}^2} + 5.\left( {\sqrt[3]{{6.20 + 5}}} \right) + 25} \right]}} = \dfrac{6}{{5.75}}\end{array}$

$T = \dfrac{{10.6}}{{5.75}} = \dfrac{4}{{25}}$

Đặt $f\left( x \right) = {x^3} - 3{x^2} + \left( {2m - 2} \right)x + m - 3$. Ta thấy hàm số liên tục trên $\mathbb{R}$.

Dễ thấy nếu $x \to  - \infty$thì $f\left( x \right) \to  - \infty$ hay $f\left( x \right) < 0$

Suy ra điều kiện cần để $f\left( x \right) = 0$ có $3$ nghiệm thỏa ${x_1} <  - 1 < {x_2} < {x_3}$ là $f\left( { - 1} \right) > 0 \Leftrightarrow  - m - 5 > 0 \Leftrightarrow m <  - 5$.

Điều kiện đủ: với $m <  - 5$ ta có

*) $\mathop {\lim }\limits_{x \to  - \infty } f\left( x \right) =  - \infty$ nên tồn tại $a <  - 1$ sao cho $f\left( a \right) < 0$

Mặt khác $f\left( { - 1} \right) =  - m - 5 > 0$. Suy ra $f\left( a \right).f\left( { - 1} \right) < 0$.

Do đó tồn tại ${x_1} \in \left( {a; - 1} \right)$ sao cho $f\left( {{x_1}} \right) = 0$.

*) $f\left( 0 \right) = m - 3 < 0$, $f\left( { - 1} \right) > 0$. Suy ra $f\left( 0 \right).f\left( { - 1} \right) < 0$.

Do đó tồn tại ${x_2} \in \left( { - 1;0} \right)$ sao cho $f\left( {{x_2}} \right) = 0$.

*) $\mathop {\lim }\limits_{x \to  + \infty } f\left( x \right) =  + \infty$ nên tồn tại $b > 0$ sao cho $f\left( b \right) > 0$

Mặt khác $f\left( 0 \right) < 0$. Suy ra $f\left( 0 \right).f\left( b \right) < 0$.

Do đó tồn tại ${x_3} \in \left( {0;b} \right)$ sao cho $f\left( {{x_3}} \right) = 0$.

Vậy $m <  - 5$ thỏa mãn yêu cầu bài toán.

Đặt $L = \mathop {\lim }\limits_{x \to 0} \left( {\dfrac{x}{{\sqrt[7]{{x + 1}}.\sqrt {x + 4}  - 2}}} \right) = \dfrac{a}{b}$ thì $\dfrac{1}{L} = \lim \left( {\dfrac{{\sqrt[7]{{x + 1}}.\sqrt {x + 4}  - 2}}{x}} \right) = \dfrac{b}{a}$.

Ta có

$\dfrac{b}{a} = \mathop {\lim }\limits_{x \to 0} \left( {\dfrac{{\sqrt[7]{{x + 1}}.\sqrt {x + 4}  - \sqrt {x + 4}  + \sqrt {x + 4}  - 2}}{x}} \right) = \mathop {\lim }\limits_{x \to 0} \left( {\dfrac{{\sqrt[7]{{x + 1}}.\sqrt {x + 4}  - \sqrt {x + 4} }}{x}} \right) + \mathop {\lim }\limits_{x \to 0} \left( {\dfrac{{\sqrt {x + 4}  - 2}}{x}} \right)$

Xét ${L_1} = \mathop {\lim }\limits_{x \to 0} \left( {\dfrac{{\sqrt {x + 4} \left( {\sqrt[7]{{x + 1}} - 1} \right)}}{x}} \right)$. Đặt $t = \sqrt[7]{{x + 1}}$. Khi đó :$\left\{ \begin{array}{l}x = {t^7} - 1\\x \to 0 \Rightarrow t \to 1\end{array} \right.$

${L_1} = \mathop {\lim }\limits_{t \to 1} \dfrac{{\sqrt {{t^7} + 3} \left( {t - 1} \right)}}{{{t^7} - 1}} = \mathop {\lim }\limits_{t \to 1} \dfrac{{\sqrt {{t^7} + 3} }}{{\left( {{t^6} + {t^5} + {t^4} + {t^3} + {t^2} + t + 1} \right)}} = \dfrac{2}{7}$

Xét ${L_2} = \mathop {\lim }\limits_{x \to 0} \left( {\dfrac{{\sqrt {x + 4}  - 2}}{x}} \right) = \mathop {\lim }\limits_{x \to 0} \dfrac{{\left( {\sqrt {x + 4}  - 2} \right)\left( {\sqrt {x + 4}  + 2} \right)}}{{x\left( {\sqrt {x + 4}  + 2} \right)}} = \mathop {\lim }\limits_{x \to 0} \dfrac{1}{{\sqrt {x + 4}  + 2}} = \dfrac{1}{4}$

Vậy $\dfrac{b}{a} = \dfrac{2}{7} + \dfrac{1}{4} = \dfrac{{15}}{{28}}$$\Rightarrow a = 28,b = 15 \Rightarrow a + b = 43$ $\Rightarrow a + b = 43$.

Ta có

$\begin{array}{l}{u_2} = {u_1} + 4.1 + 3\\{u_3} = {u_2} + 4.2 + 3\\...\\{u_n} = {u_{n - 1}} + 4.\left( {n - 1} \right) + 3\end{array}$

Cộng vế theo vế và rút gọn ta được

${u_n} = {u_1} + 4.\left( {1 + 2 + ... + n - 1} \right) + 3\left( {n - 1} \right)$$= 4\dfrac{{n\left( {n - 1} \right)}}{2} + 3\left( {n - 1} \right)$$= 2{n^2} + n - 3$, với mọi $n \ge 1$.

Suy ra                                                           

$\begin{array}{l}{u_{2n}} = 2{\left( {2n} \right)^2} + 2n - 3\\{u_{{2^2}n}} = 2{\left( {{2^2}n} \right)^2} + {2^2}n - 3\\...\\{u_{{2^{2018}}n}} = 2{\left( {{2^{2018}}n} \right)^2} + {2^{2018}}n - 3\end{array}$

Và

$\begin{array}{l}{u_{4n}} = 2{\left( {4n} \right)^2} + 4n - 3\\{u_{{4^2}n}} = 2{\left( {{4^2}n} \right)^2} + {4^2}n - 3\\...\\{u_{{4^{2018}}n}} = 2{\left( {{4^{2018}}n} \right)^2} + {4^{2018}}n - 3\end{array}$

Do đó $\lim \dfrac{{\sqrt {{u_n}}  + \sqrt {{u_{4n}}}  + \sqrt {{u_{{4^2}n}}}  + ... + \sqrt {{u_{{4^{2018}}n}}} }}{{\sqrt {{u_n}}  + \sqrt {{u_{2n}}}  + \sqrt {{u_{{2^2}n}}}  + ... + \sqrt {{u_{{2^{2018}}n}}} }}$

$= \lim \dfrac{{\sqrt {2 + \dfrac{1}{n} - \dfrac{3}{{{n^2}}}}  + \sqrt {{{2.4}^2} + \dfrac{4}{n} - \dfrac{3}{{{n^2}}}}  + ... + \sqrt {2{{\left( {{4^{2018}}} \right)}^2} + \dfrac{{{4^{2018}}}}{n} - \dfrac{3}{{{n^2}}}} }}{{\sqrt {2 + \dfrac{1}{n} - \dfrac{3}{{{n^2}}}}  + \sqrt {{{2.2}^2} + \dfrac{2}{n} - \dfrac{3}{{{n^2}}}}  + ... + \sqrt {2{{\left( {{2^{2018}}} \right)}^2} + \dfrac{{{2^{2018}}}}{n} - \dfrac{3}{{{n^2}}}} }}$

$= \dfrac{{\sqrt 2 \left( {1 + 4 + {4^2} + ... + {4^{2018}}} \right)}}{{\sqrt 2 \left( {1 + 2 + {2^2} + ... + {2^{2018}}} \right)}}$$= \dfrac{{1\dfrac{{1 - {4^{2019}}}}{{1 - 4}}}}{{\dfrac{{1 - {2^{2019}}}}{{1 - 2}}}}$$= \dfrac{1}{3}\dfrac{{{4^{2019}} - 1}}{{{2^{2019}} - 1}}$$= \dfrac{{{2^{2019}} + 1}}{3}$.

Vì ${2^{2019}} > 2019$ cho nên sự xác định ở trên là duy nhất nên $\left\{ \begin{array}{l}a = 2\\b = 1\\c = 3\end{array} \right.$

Vậy $S = a + b - c = 0$.

Ta có $\dfrac{1}{{n\sqrt {n + 1}  + \left( {n + 1} \right)\sqrt n }}$$= \dfrac{1}{{\sqrt n \sqrt {n + 1} \left( {\sqrt n  + \sqrt {n + 1} } \right)}}$ $= \dfrac{{\sqrt {n + 1}  - \sqrt n }}{{\sqrt n \sqrt {n + 1} }} = \dfrac{1}{{\sqrt n }} - \dfrac{1}{{\sqrt {n + 1} }}$

Suy ra

${S_n} = \dfrac{1}{{1\sqrt 2  + 2\sqrt 1 }} + \dfrac{1}{{2\sqrt 3  + 3\sqrt 2 }} + ... + \dfrac{1}{{n\sqrt {n + 1}  + \left( {n + 1} \right)\sqrt n }}$

$= \dfrac{1}{1} - \dfrac{1}{{\sqrt 2 }} + \dfrac{1}{{\sqrt 2 }} - \dfrac{1}{{\sqrt 3 }} + ... + \dfrac{1}{{\sqrt n }} - \dfrac{1}{{\sqrt {n + 1} }} = 1 - \dfrac{1}{{\sqrt {n + 1} }}$

Suy ra $\lim {S_n} = 1$

Cách 1:

Xét dãy số $\left( {{u_n}} \right)$, với ${u_n} = \left( {1 - \dfrac{1}{{{2^2}}}} \right)\left( {1 - \dfrac{1}{{{3^2}}}} \right)...\left( {1 - \dfrac{1}{{{n^2}}}} \right)$, $n \ge 2,\,n \in \mathbb{N}$.

Ta có:

${u_2} = 1 - \dfrac{1}{{{2^2}}} = \dfrac{3}{4} = \dfrac{{2 + 1}}{{2.2}}$;

${u_3} = \left( {1 - \dfrac{1}{{{2^2}}}} \right).\left( {1 - \dfrac{1}{{{3^2}}}} \right) = \dfrac{3}{4}.\dfrac{8}{9} = \dfrac{4}{6} = \dfrac{{3 + 1}}{{2.3}}$;

${u_4} = \left( {1 - \dfrac{1}{{{2^2}}}} \right).\left( {1 - \dfrac{1}{{{3^2}}}} \right)\left( {1 - \dfrac{1}{{{4^2}}}} \right) = \dfrac{3}{4}.\dfrac{8}{9}.\dfrac{{15}}{{16}} = \dfrac{5}{8} = \dfrac{{4 + 1}}{{2.4}}$

$\cdots  \cdots$

${u_n} = \dfrac{{n + 1}}{{2n}}$.

Dễ dàng chứng minh bằng phương pháp qui nạp để khẳng định ${u_n} = \dfrac{{n + 1}}{{2n}},\,\forall n \ge 2$

Khi đó $\lim \left[ {\left( {1 - \dfrac{1}{{{2^2}}}} \right)\left( {1 - \dfrac{1}{{{3^2}}}} \right)...\left( {1 - \dfrac{1}{{{n^2}}}} \right)} \right] = \lim \dfrac{{n + 1}}{{2n}} = \dfrac{1}{2}$.

$I = \mathop {\lim }\limits_{x \to 1} \dfrac{{2x - \sqrt {x + 3} }}{{{x^2} - 1}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {2x - \sqrt {x + 3} } \right)\left( {2x + \sqrt {x + 3} } \right)}}{{\left( {x - 1} \right)\left( {x + 1} \right)\left( {2x + \sqrt {x + 3} } \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{4{x^2} - x - 3}}{{\left( {x - 1} \right)\left( {x + 1} \right)\left( {2x + \sqrt {x + 3} } \right)}}$

$= \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {x - 1} \right)\left( {4x + 3} \right)}}{{\left( {x - 1} \right)\left( {x + 1} \right)\left( {2x + \sqrt {x + 3} } \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{4x + 3}}{{\left( {x + 1} \right)\left( {2x + \sqrt {x + 3} } \right)}} = \dfrac{7}{8}$.

Ta có $\mathop {\lim }\limits_{x \to 1} \dfrac{{\sqrt {{x^2} + x + 2}  - \sqrt[3]{{7x + 1}}}}{{\sqrt 2 \left( {x - 1} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{\sqrt {{x^2} + x + 2}  - 2 + 2 - \sqrt[3]{{7x + 1}}}}{{\sqrt 2 \left( {x - 1} \right)}}$

$= \mathop {\lim }\limits_{x \to 1} \dfrac{{\sqrt {{x^2} + x + 2}  - 2}}{{\sqrt 2 \left( {x - 1} \right)}} + \mathop {\lim }\limits_{x \to 1} \dfrac{{2 - \sqrt[3]{{7x + 1}}}}{{\sqrt 2 \left( {x - 1} \right)}} = I + J$.

Tính $I = \mathop {\lim }\limits_{x \to 1} \dfrac{{\sqrt {{x^2} + x + 2}  - 2}}{{\sqrt 2 \left( {x - 1} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{{x^2} + x + 2 - 4}}{{\sqrt 2 \left( {x - 1} \right)\left( {\sqrt {{x^2} + x + 2}  + 2} \right)}}$

$= \mathop {\lim }\limits_{x \to 1} \dfrac{{\left( {x - 1} \right)\left( {x + 2} \right)}}{{\sqrt 2 \left( {x - 1} \right)\left( {\sqrt {{x^2} + x + 2}  + 2} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{x + 2}}{{\sqrt 2 \left( {\sqrt {{x^2} + x + 2}  + 2} \right)}} = \dfrac{3}{{4\sqrt 2 }}$.

và $J = \mathop {\lim }\limits_{x \to 1} \dfrac{{2 - \sqrt[3]{{7x + 1}}}}{{\sqrt 2 \left( {x - 1} \right)}} = \mathop {\lim }\limits_{x \to 1} \dfrac{{8 - 7x - 1}}{{\sqrt 2 \left( {x - 1} \right)\left[ {4 + 2\sqrt[3]{{7x + 1}} + {{\left( {\sqrt[3]{{7x + 1}}} \right)}^2}} \right]}}$

$\mathop { = \lim }\limits_{x \to 1} \dfrac{{ - 7}}{{\sqrt 2 \left[ {4 + 2\sqrt[3]{{7x + 1}} + {{\left( {\sqrt[3]{{7x + 1}}} \right)}^2}} \right]}} = \dfrac{{ - 7}}{{12\sqrt 2 }}$.

Do đó $\mathop {\lim }\limits_{x \to 1} \dfrac{{\sqrt {{x^2} + x + 2}  - \sqrt[3]{{7x + 1}}}}{{\sqrt 2 \left( {x - 1} \right)}} = I + J = \dfrac{{\sqrt 2 }}{{12}}$

Suy ra $a = 1$, $b = 12$, $c = 0$. Vậy $a + b + c = 13$.