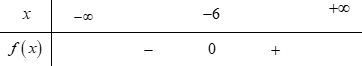Cho f(x)=ax2+bx+c(a≠0). Điều kiện để f(x)>0,∀x∈R là
Ta có:
f(x)>0,∀x∈R khi a>0 và Δ<0.
Cho f(x)=ax2+bx+c(a≠0). Điều kiện để f(x)≤0,∀x∈R là
Ta có:
f(x)≤0,∀x∈R khi a<0 và Δ≤0.
Cho f(x)=ax2+bx+c(a≠0) có Δ=b2−4ac<0. Khi đó mệnh đề nào đúng?
Đáp án A, B sai vì chưa biết dấu của a nên chưa kết luận được dấu của f(x)
Vì Δ<0 và a≠0 nên f(x) không đổi dấu trên R.
Tam thức bậc hai f(x)=2x2+2x+5 nhận giá trị dương khi và chỉ khi
Ta có {a=2>0Δ′=1−2.5=−9<0⇒f(x)>0,∀x∈R.
Cho các tam thức f(x)=2x2−3x+4;g(x)=−x2+3x−4;h(x)=4−3x2. Số tam thức đổi dấu trên R là:
Vì f(x)=0 vô nghiệm do Δ=9−4.2.4=−23<0
g(x)=0 vô nghiệm do Δ=9−4.(−1).(−4)=−7<0
h(x)=0 có hai nghiệm phân biệt do:
4−3x2=0⇔3x2=4 ⇔x2=43⇔x=±2√3
Nên chỉ có h(x) đổi dấu trên R.
Tam thức bậc hai f(x)=x2+(1−√3)x−8−5√3:
Ta có f(x)=0⇔[x=−2−√3x=1+2√3.
Bảng xét dấu
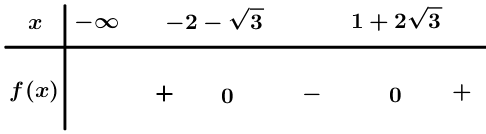
Dựa vào bảng xét dấu f(x)<0⇔−2−√3<x<1+2√3.
Bảng xét dấu nào sau đây là của tam thức f(x)=x2+12x+36?
Ta có:
f(x)=x2+12x+36Δ=122−4.1.36=0
Do đó, tam thức bậc hai f(x) có một nghiệm duy nhất x=−122.1=−6
a=1>0 nên f(x)>0,∀x≠−6 hay f(x)≥0 với mọi x.
Do đó ta có bảng xét dấu cần tìm.
Cho tam thức bậc hai f(x)=x2−bx+3. Với giá trị nào của b thì tam thức f(x) có hai nghiệm phân biệt?
Ta có f(x)=x2−bx+3 có hai nghiệm phân biệt khi Δ=b2−12>0⇔[b<−2√3b>2√3.
Giá trị nào của m thì phương trình (m−3)x2+(m+3)x−(m+1)=0 (1) có hai nghiệm phân biệt?
Ta có (1) có hai nghiệm phân biệt khi {a≠0Δ>0⇔{m≠35m2−2m−3>0⇔{m≠3(m−1)(5m+3)>0⇔{m≠3[m<−35m>1
Tìm tập xác định của hàm số y=√2x2−5x+2.
Điều kiện 2x2−5x+2≥0⇔[x≥2x≤12.
Vậy tập xác định của hàm số là (−∞;12]∪[2;+∞).
Các giá trị m để tam thức f(x)=x2−(m+2)x+8m+1 đổi dấu 2 lần là
Tam thức f(x)=x2−(m+2)x+8m+1 đổi dấu 2 lần khi và chỉ khi
Δ>0⇔(m+2)2−4(8m+1)>0⇔m2−28m>0⇔[m>28m<0.
Tập nghiệm của hệ bất phương trình {x2−4x+3>0x2−6x+8>0 là
Ta có: {x2−4x+3>0x2−6x+8>0 ⇔{[x<1x>3[x<2x>4 ⇔[{x<1x<2{x<1x>4(VN){x>3x<2(VN){x>3x>4 ⇔[x<1x>4.
Tìm m để (m+1)x2+mx+m<0,∀x∈R?
Với m=−1 thì bpt trở thành −x−1<0⇔x>−1 nên bpt không đúng với mọi x (loại)
Do đó m=-1 không thỏa mãn.
Với m≠−1, (m+1)x2+mx+m<0,∀x∈R⇔{a<0Δ<0⇔{m+1<0m2−4m(m+1)<0
⇔{m+1<0−3m2−4m<0⇔{m<−1[m<−43m>0⇔[{m<−1m<−43{m<−1m>0(VN)⇔{m<−1m<−43 ⇔m<−43.
Tìm m để f(x)=x2−2(2m−3)x+4m−3>0,∀x∈R?
f(x)=x2−2(2m−3)x+4m−3>0,∀x∈R
⇔{a=1>0(đúng)Δ′<0⇔(2m−3)2−1.(4m−3)<0⇔4m2−12m+9−4m+3<0⇔4m2−16m+12<0⇔1<m<3
Với giá trị nào của a thì bất phương trình ax2−x+a≥0 nghiệm đúng với ∀x∈R ?
Để bất phương trình ax2−x+a≥0,∀x∈R⇔{Δ≤0a>0⇔{1−4a2≤0a>0⇔{[a≥12a≤−12a>0⇔a≥12.
Với giá trị nào của m thì bất phương trình x2−x+m≤0 vô nghiệm?
Bất phương trình x2−x+m≤0 vô nghiệm khi và chỉ khi bất phương trình x2−x+m>0 nghiệm đúng với ∀x∈R ⇔{Δ<01>0⇔1−4m<0⇔m>14.
Tìm m để hệ {x2−2x+1−m≤0(1)x2−(2m+1)x+m2+m≤0(2) có nghiệm.
(1)⇔(x−1)2−m≤0⇔(x−1)2≤m
Do (x−1)2≥0,∀x nên để bpt trên có nghiệm thì m≥0.
Khi đó −√m≤x−1≤√m ⇔1−√m≤x≤1+√m
Tập nghiệm của (1) là S1=[1−√m;1+√m].
(2)⇔x2−2mx−x+m2+m≤0 ⇔(x2−2mx+m2)−(x−m)≤0
⇔(x−m)2−(x−m)≤0 ⇔(x−m)(x−m−1)≤0 ⇔m≤x≤m+1
Tập nghiệm của (2) là S2=[m;m+1]
Để hệ đã cho có nghiệm thì S1∩S2≠∅
⇔[1−√m;1+√m]∩[m;m+1]≠∅(∗)
Cách 1:
(∗)⇔{m≤1+√m1−√m≤m+1 ⇔{m−1≤√m(3)m+√m≥0(4)
(3)⇔[m−1<0{m−1≥0m2−2m+1≤m ⇔[m<1{m≥1m2−3m+1≤0 ⇔[m<1{m≥13−√52≤m≤3+√52 ⇔[m<11≤m≤3+√52 ⇔m≤3+√52
(4) luôn đúng vì m≥0 nên m+√m≥0.
Vậy 0≤m≤3+√52.
Tìm tất cả giá trị thực của tham số m để hệ bất phương trình {x2+10x+16≤0(1)mx≥3m+1(2) vô nghiệm.
Bất phương trình (1)⇔−8≤x≤−2. Suy ra S1=[−8;−2].
Giải bất phương trình (2)
Với m=0 thì bất phương trình (2) trở thành 0x≥1 : vô nghiệm .
Với m>0 thì bất phương trình (2) tương đương với x≥3m+1m .
Suy ra S2=[3m+1m;+∞).
Hệ vô nghiệm ⇔−2<3m+1m ⇔−2m<3m+1⇔m>−15.
Kết hợp m>0 ta được m>0.
+) Với m<0 thì bất phương trình (2) tương đương với x≤3m+1m.
Suy ra S2=(−∞;3m+1m].
Hệ vô nghiệm ⇔3m+1m<−8 ⇔3m+1>−8m⇔m>−111.
Kết hợp với m<0 ta được −111<m<0.
Vậy m>−111.
Có bao nhiêu giá trị m nguyên âm để mọi x>0 đều thoả bất phương trình (x2+x+m)2≥(x2−3x−m)2?
Ta có (x2+x+m)2≥(x2−3x−m)2⇔(x2+x+m)2−(x2−3x−m)2≥0
⇔4x(2x+m)(x−1)≥0
Với m<0 ta có bảng xét dấu
TH1: −m2≥1
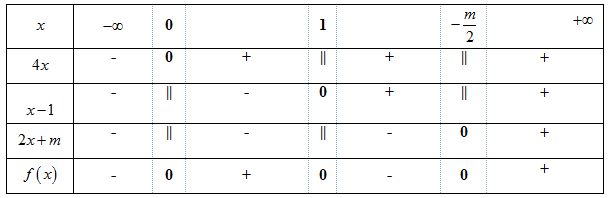
Từ Bảng xét dấu ta thấy để BPT nghiệm đúng với x>0 thì −m2=1⇔m=−2
TH 2: 0<−m2<1
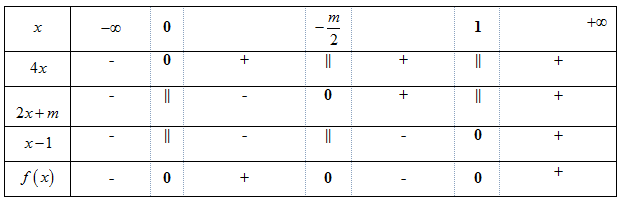
Từ Bảng xét dấu ta thấy để BPT nghiệm đúng với x>0 thì −m2=1⇔m=−2
Vậy có 1 giá trị
Tìm tập xác định D của hàm số y=√x2+5x+42x2+3x+1 là
Hàm số xác định khi và chỉ khi f(x)=x2+5x+42x2+3x+1≥0.
Phương trình x2+5x+4=0⇔[x=−1x=−4 và 2x2+3x+1=0⇔[x=−1x=−12.
Bảng xét dấu
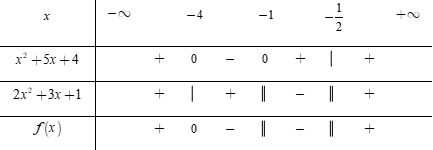
Dựa vào bảng xét dấu ta thấy x2+5x+42x2+3x+1≥0⇔x∈(−∞;−4]∪(−12;+∞)
Vậy tập xác định của hàm số là D=(−∞;−4]∪(−12;+∞).