Tìm x∈Z để P+1∈Z.
Theo câu trước ta có P=−3x+7x+4 với x≠1;x≠−4, nên
P+1=−3x+7x+4+1=−3x+7+x+4x+4=−2x+11x+4=−2+19x+4
x∈Z để P+1∈Z⇒(x+4)∈U(19)={±1;±19}
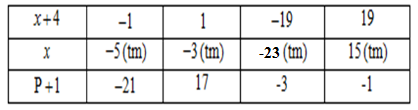
Vậy x∈{−23;−5;−3;15} thì P+1∈Z.
Tính giá trị của P khi x=−1.
Theo câu trước ta có: P=−3x+7x+4 với x≠1;x≠−4
Khi x=−1(t/m)⇒P=−3.(−1)+7−1+4=103
Vậy khi x=−1 thì P=103.
Rút gọn P ta được
ĐK: {x2+3x−4≠0x+4≠01−x≠0⇔{(x−1)(x+4)≠0x≠1x≠−4⇔{x≠1x≠−4.
P=10xx2+3x−4−2x−3x+4+x+11−x=10x(x−1)(x+4)−2x−3x+4−x+1x−1=10x−(2x−3)(x−1)−(x+1)(x+4)(x−1)(x+4)=10x−2x2+2x+3x−3−x2−4x−x−4(x−1)(x+4)=−3x2+10x−7(x−1)(x+4)=−−(x−1)(3x−7)(x−1)(x+4)=−3x+7x+4.
Vậy P=−3x+7x+4 với x≠1;x≠−4
Rút gọn P ta được
ĐK: {x2+3x−4≠0x+4≠01−x≠0⇔{(x−1)(x+4)≠0x≠1x≠−4⇔{x≠1x≠−4.
P=10xx2+3x−4−2x−3x+4+x+11−x=10x(x−1)(x+4)−2x−3x+4−x+1x−1=10x−(2x−3)(x−1)−(x+1)(x+4)(x−1)(x+4)=10x−2x2+2x+3x−3−x2−4x−x−4(x−1)(x+4)=−3x2+10x−7(x−1)(x+4)=−−(x−1)(3x−7)(x−1)(x+4)=−3x+7x+4.
Vậy P=−3x+7x+4 với x≠1;x≠−4
Phân thức 5x−73x2+6x xác định khi:
ĐK: 3x2+6x≠0⇔3x(x+2)≠0⇔{x≠0x≠−2
Đa thức thích hợp để điền vào chỗ trống trong đẳng thức x3−8......=x2+2x+43x là:
x2+2x+43x=(x−2)(x2+2x+4)3x(x−2)=x3−83x(x−2)⇒x3−83x(x−2)=x3−8......
Vậy đa thức cần tìm là 3x(x−2)
Đa thức P trong đẳng thức 5(y−x)25x2−5xy=x−yP là:
Ta có: 5(y−x)25x2−5xy=5(x−y)25x(x−y)=x−yx⇒x−yx=x−yP⇒P=x.
Kết quả của phép tính 3x−12xy−5x−22xy là:
3x−12xy−5x−22xy=3x−1−5x+22xy=−2x+12xy.
Thực hiện phép tính sau: x3x2+1+xx2+1
Ta có x3x2+1+xx2+1=x3+xx2+1=x(x2+1)x2+1=x.
Thực hiện phép tính sau 2x+55x2y2+85xy2+2x−1x2y2, ta được kết quả là:
2x+55x2y2+85xy2+2x−1x2y2=2x+5+8x+10x−55x2y2=20x5x2y2=4xy2.
Điền vào chỗ trống: 2x−6x+3−....=x+12.
Gọi phân thức cần điền là P, khi đó
P=2x−6x+3−x+12=2(2x−6)−(x+3)(x+1)2(x+3)=4x−12−x2−x−3x−32(x+3)=−x2−152(x+3).
Kết quả của phép tính 1x+1x(x+1)+...+1(x+9)(x+10) là:
Ta có : 1x+1x(x+1)+...+1(x+9)(x+10)
=1x+1x−1x+1+1x+1−1x+2...+1x+9−1x+10=1x+1x+0+...+0−1x+10=2x−1x+10=2x+20−xx(x+10)=x+20x(x+10).
Rút gọn biểu thức 1x+2+1(x+1)(x+2)+1(x+1)(2x+1) ta được
Điều kiện: x≠−1;x≠−2;x≠−12.
1x+2+1(x+1)(x+2)+1(x+1)(2x+1)=(2x+1)(x+1)+2x+1+x+2(x+1)(x+2)(2x+1)=2x2+x+2x+1+2x+1+x+2(x+1)(x+2)(2x+1)=2x2+6x+4(x+1)(x+2)(2x+1)=2(x2+3x+2)(x+1)(x+2)(2x+1)=2(x2+x+2x+2)(x+1)(x+2)(2x+1)=2[x(x+1)+2(x+1)](x+1)(x+2)(2x+1)=2(x+1)(x+2)(x+1)(x+2)(2x+1)=22x+1.
Chọn câu đúng.
Điều kiện: x≠1.
4x2−3x+5x3−1−1−2xx2+x+1−6x−1=4x2−3x+5−(1−2x)(x−1)−6(x2+x+1)(x−1)(x2+x+1)=4x2−3x+5−x+1+2x2−2x−6x2−6x−6(x−1)(x2+x+1)=−12xx3−1.
Tìm P biết: P+4x−12x3−3x2−4x+12=3x−3−x24−x2
ĐK: x≠{−2;2;3}.
P+4x−12x3−3x2−4x+12=3x−3−x24−x2P=3x−3−x24−x2−4x−12x3−3x2−4x+12P=3x−3+x2(x−2)(x+2)−4x−12x2(x−3)−4(x−3)P=3(x2−4)(x−3)(x2−4)+x2(x−3)(x−3)(x2−4)−4x−12(x−3)(x2−4)
P=3x2−12+x3−3x2−4x+12(x−3)(x2−4)
P=x3−4x(x−3)(x−2)(x+2)P=x(x2−4)(x−3)(x−2)(x+2)=xx−3
Thực hiện phép tính 3x+15x2−4:x+5x−2 ta được:
3x+15x2−4:x+5x−2=3x+15x2−4⋅x−2x+5=3(x+5)(x−2)(x+2)⋅x−2x+5=3x+2.
Rút gọn biểu thức x4+4x2+55x3+5⋅2xx2+4⋅3x3+3x4+4x2+5 ta được:
x4+4x2+55x3+5⋅2xx2+4⋅3x3+3x4+4x2+5=x4+4x2+55(x3+1)⋅2xx2+4⋅3(x3+1)x4+4x2+5=6x5(x2+4).
Biểu thức P=x−12−x:x−1x+2⋅x−24−x2 có kết quả rút gọn là:
P=x−12−x:x−1x+2⋅x−24−x2=x−12−x⋅x+2x−1⋅−(2−x)(x+2)(2−x)=−12−x=1x−2
Tìm biểu thức Q, biết: 5xx2+2x+1⋅Q=xx2−1
5xx2+2x+1⋅Q=xx2−1⇒Q=xx2−1:5xx2+2x+1=xx2−1⋅x2+2x+15x=x(x−1)(x+1)⋅(x+1)25x=x+15(x−1)
Tìm x, biết: 1x⋅xx+1⋅x+1x+2⋅x+2x+3⋅x+3x+4⋅x+4x+5⋅x+5x+6=1
Điều kiện: x≠{0;−1;−2;−3;−4;−5;−6}
1x⋅xx+1⋅x+1x+2⋅x+2x+3⋅x+3x+4⋅x+4x+5⋅x+5x+6=1⇔1x+6=1⇒x+6=1⇔x=−5(KTM)
Vậy phương trình vô nghiệm.

