Thực hiện phép tính \(\dfrac{{x - 6}}{{{x^2} + 1}}\,\, \cdot \,\,\dfrac{{3{x^2} - 3x + 3}}{{{x^2} - 36}} + \dfrac{{x - 6}}{{{x^2} + 1}}\,\, \cdot \,\,\dfrac{{3x}}{{{x^2} - 36}}\) ta được kết quả là
\(\dfrac{{x - 6}}{{{x^2} + 1}}\,\, \cdot \,\,\dfrac{{3{x^2} - 3x + 3}}{{{x^2} - 36}} + \dfrac{{x - 6}}{{{x^2} + 1}}\,\, \cdot \,\,\dfrac{{3x}}{{{x^2} - 36}}\)
\( = \dfrac{{x - 6}}{{{x^2} + 1}}\left( {\dfrac{{3{x^2} - 3x + 3}}{{{x^2} - 36}} + \dfrac{{3x}}{{{x^2} - 36}}} \right)\)
\( = \dfrac{{x - 6}}{{{x^2} + 1}} \cdot \dfrac{{3{x^2} - 3x + 3 + 3x}}{{{x^2} - 36}}\)
\( = \dfrac{{x - 6}}{{{x^2} + 1}} \cdot \dfrac{{3{x^2} + 3}}{{(x - 6)(x + 6)}}\)
\( = \dfrac{{x - 6}}{{{x^2} + 1}} \cdot \dfrac{{3({x^2} + 1)}}{{(x - 6)(x + 6)}} = \dfrac{3}{{x + 6}}.\)
Tìm biểu thức M, biết \(\dfrac{{x + 2y}}{{{x^3} - 8{y^3}}}\, \cdot \,M = \dfrac{{5{x^2} + 10xy}}{{{x^2} + 2xy + 4{y^2}}}\)
\(\begin{array}{l}\,\,\dfrac{{x + 2y}}{{{x^3} - 8{y^3}}}\, \cdot \,M = \dfrac{{5{x^2} + 10xy}}{{{x^2} + 2xy + 4{y^2}}}\\M = \dfrac{{5{x^2} + 10xy}}{{{x^2} + 2xy + 4{y^2}}}:\dfrac{{x + 2y}}{{{x^3} - 8{y^3}}}\\M = \dfrac{{5{x^2} + 10xy}}{{{x^2} + 2xy + 4{y^2}}} \cdot \dfrac{{{x^3} - 8{y^3}}}{{x + 2y}}\\M = \dfrac{{5x(x + 2y)}}{{{x^2} + 2xy + 4{y^2}}} \cdot \dfrac{{(x - 2y)({x^2} + 2xy + 4{y^2})}}{{x + 2y}}\\M = 5x(x - 2y).\end{array}\)
Thực hiện phép tính sau $\left( {\dfrac{{2x}}{{3x + 1}} - 1} \right):\left( {1 - \dfrac{{8{x^2}}}{{9{x^2} - 1}}} \right)$, ta được kết quả là:
$\begin{array}{l}\left( {\dfrac{{2x}}{{3x + 1}} - 1} \right):\left( {1 - \dfrac{{8{x^2}}}{{9{x^2} - 1}}} \right) = \left( {\dfrac{{2x - 3x - 1}}{{3x + 1}}} \right):\left( {\dfrac{{9{x^2} - 1 - 8{x^2}}}{{9{x^2} - 1}}} \right)\\ = \dfrac{{ - x - 1}}{{3x + 1}}:\dfrac{{{x^2} - 1}}{{9{x^2} - 1}} = \dfrac{{ - x - 1}}{{3x + 1}}.\dfrac{{9{x^2} - 1}}{{{x^2} - 1}}\\ = \dfrac{{ - (x + 1)}}{{3x + 1}}.\dfrac{{(3x + 1)(3x - 1)}}{{(x + 1)(x - 1)}} = \dfrac{{1 - 3x}}{{x - 1}}.\end{array}$
Thực hiện phép tính $C = \dfrac{{2{x^2} + 4x + 8}}{{{x^3} - 3{x^2} - x + 3}}:\dfrac{{{x^3} - 8}}{{(x + 1)(x - 3)}}$
$C = \dfrac{{2{x^2} + 4x + 8}}{{{x^3} - 3{x^2} - x + 3}}:\dfrac{{{x^3} - 8}}{{(x + 1)(x - 3)}}$
$\begin{array}{l}C = \dfrac{{2({x^2} + 2x + 4)}}{{{x^2}(x - 3) - (x - 3)}}.\dfrac{{(x + 1)(x - 3)}}{{(x - 2)({x^2} + 2x + 4)}}\\\,\,\,\,\, = \dfrac{{2(x + 1)(x - 3)}}{{(x - 3)({x^2} - 1)(x - 2)}}\\\,\,\,\,\, = \dfrac{2}{{(x - 1)(x - 2)}}.\end{array}$
Vậy \(C = \dfrac{2}{{\left( {x - 1} \right)\left( {x - 2} \right)}}\)
Cho $Q = \left( {\dfrac{{{x^2} + 3x}}{{{x^3} + 3{x^2} + 9x + 27}} + \dfrac{3}{{{x^2} + 9}}} \right):\left( {\dfrac{1}{{x - 3}} - \dfrac{{6x}}{{{x^3} - 3{x^2} + 9x - 27}}} \right).$ Rút gọn $Q$ ta được.
$Q = \left( {\dfrac{{{x^3} + 3x}}{{{x^3} + 3{x^2} + 9x + 27}} + \dfrac{3}{{{x^2} + 9}}} \right):\left( {\dfrac{1}{{x - 3}} - \dfrac{{6x}}{{{x^3} - 3{x^2} + 9x - 27}}} \right)$ (ĐK: \(x \ne \pm 3\))
$\begin{array}{l}Q = \left( {\dfrac{{{x^2} + 3x}}{{{x^3} + 3{x^2} + 9x + 27}} + \dfrac{3}{{{x^2} + 9}}} \right):\left( {\dfrac{1}{{x - 3}} - \dfrac{{6x}}{{{x^3} - 3{x^2} + 9x - 27}}} \right)\\\,\,\,\,\, = \left( {\dfrac{{{x^2} + 3x}}{{{x^2}(x + 3) + 9(x + 3)}} + \dfrac{3}{{{x^2} + 9}}} \right):\left( {\dfrac{1}{{x - 3}} - \dfrac{{6x}}{{{x^2}(x - 3) + 9(x - 3)}}} \right)\\\,\,\,\,\, = \dfrac{{{x^2} + 3x + 3x + 9}}{{\left( {{x^2} + 9} \right)\left( {x + 3} \right)}}:\dfrac{{{x^2} + 9 - 6x}}{{\left( {x - 3} \right)\left( {{x^2} + 9} \right)}}\\\,\,\,\,\,\, = \dfrac{{{{(x + 3)}^2}}}{{({x^2} + 9)(x + 3)}}.\dfrac{{(x - 3)({x^2} + 9)}}{{{{(x - 3)}^2}}}\\\,\,\,\,\,\, = \dfrac{{x + 3}}{{x - 3}}.\end{array}$
Cho biểu thức $P = \dfrac{{10x}}{{{x^2} + 3x - 4}} - \dfrac{{2x - 3}}{{x + 4}} + \dfrac{{x + 1}}{{1 - x}}$
Rút gọn \(P\) ta được
ĐK: \(\left\{ \begin{array}{l}{x^2} + 3x - 4 \ne 0\\x + 4 \ne 0\\1 - x \ne 0\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}\left( {x - 1} \right)\left( {x + 4} \right) \ne 0\\x \ne 1\\x \ne - 4\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x \ne 1\\x \ne - 4\end{array} \right..\)
$\begin{array}{l}P = \dfrac{{10x}}{{{x^2} + 3x - 4}} - \dfrac{{2x - 3}}{{x + 4}} + \dfrac{{x + 1}}{{1 - x}}\\ = \dfrac{{10x}}{{\left( {x - 1} \right)\left( {x + 4} \right)}} - \dfrac{{2x - 3}}{{x + 4}} - \dfrac{{x + 1}}{{x - 1}}\\ = \dfrac{{10x - (2x - 3)(x - 1) - (x + 1)(x + 4)}}{{(x - 1)(x + 4)}}\\ = \dfrac{{10x - 2{x^2} + 2x + 3x - 3 - {x^2} - 4x - x - 4}}{{(x - 1)(x + 4)}}\\ = \dfrac{{ - 3{x^2} + 10x - 7}}{{(x - 1)(x + 4)}}\\ = - \dfrac{{ - (x - 1)(3x - 7)}}{{(x - 1)(x + 4)}}\\ = \dfrac{{ - 3x + 7}}{{x + 4}}.\end{array}$
Vậy $P = \dfrac{{ - 3x + 7}}{{x + 4}}$ với \(x \ne 1;x \ne -4\)
Cho biểu thức $P = \dfrac{{10x}}{{{x^2} + 3x - 4}} - \dfrac{{2x - 3}}{{x + 4}} + \dfrac{{x + 1}}{{1 - x}}$
Tính giá trị của $P$ khi $x = - 1.$
Theo câu trước ta có: $P = \dfrac{{ - 3x + 7}}{{x + 4}}$ với \(x \ne 1;x \ne -4\)
Khi $x = - 1(t/m) \Rightarrow P = \dfrac{{ - 3.( - 1) + 7}}{{ - 1 + 4}} = \dfrac{{10}}{3}$
Vậy khi $x = - 1$ thì $P = \dfrac{{10}}{3}.$
Cho biểu thức $P = \dfrac{{10x}}{{{x^2} + 3x - 4}} - \dfrac{{2x - 3}}{{x + 4}} + \dfrac{{x + 1}}{{1 - x}}$
Tìm $x \in \mathbb{Z}$ để $P + 1 \in \mathbb{Z}$.
Theo câu trước ta có $P = \dfrac{{ - 3x + 7}}{{x + 4}}$ với \(x \ne 1;x \ne -4\), nên
$P + 1 = \dfrac{{ - 3x + 7}}{{x + 4}} + 1 = \dfrac{{ - 3x + 7 + x + 4}}{{x + 4}} = \dfrac{{ - 2x + 11}}{{x + 4}} = - 2 + \dfrac{{19}}{{x + 4}}$
$x \in Z$ để $P + 1 \in Z \Rightarrow \left( {x + 4} \right) \in U\left( {19} \right) = \left\{ { \pm 1;\, \pm 19} \right\}$
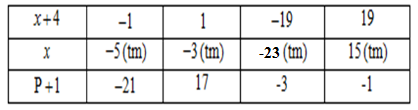
Vậy $x \in \left\{ { - 23; - 5; - 3;15} \right\}$ thì $P + 1 \in Z$.
Cho $x;y;z \ne 0$ thỏa mãn $x + y + z = 0$. Chọn câu đúng về biểu thức$A = \dfrac{{xy}}{{{x^2} + {y^2} - {z^2}}} + \dfrac{{yz}}{{{y^2} + {z^2} - {x^2}}} + \dfrac{{zx}}{{{z^2} + {x^2} - {y^2}}}$.
Từ $x + y + z = 0 \Rightarrow x + y = - z \Rightarrow {x^2} + 2xy + {y^2} = {z^2} \Rightarrow {x^2} + {y^2} - {z^2} = - 2xy$.
Tương tự ta có : $\left\{ \begin{array}{l}{y^2} + {z^2} - {x^2} = - 2yz\\{z^2} + {x^2} - {y^2} = - 2zx\end{array} \right.$
Do đó: $A = \dfrac{{xy}}{{ - 2xy}} + \dfrac{{yz}}{{ - 2yz}} + \dfrac{{zx}}{{ - 2zx}} = - \dfrac{1}{2} - \dfrac{1}{2} - \dfrac{1}{2} = - \dfrac{3}{2}$
Vậy $A = - \dfrac{3}{2}.$
Suy ra \(A < - 1.\)
Giá trị lớn nhất của phân thức $\dfrac{5}{{{x^2} - 6x + 10}}$ là :
Ta có: $\dfrac{5}{{{x^2} - 6x + 10}} = \dfrac{5}{{{x^2} - 6x + 9 + 1}} = \dfrac{5}{{{{(x - 3)}^2} + 1}}$
Vì ${(x - 3)^2} \ge 0 \Rightarrow {(x - 3)^2} + 1 \ge 1 \Rightarrow \dfrac{1}{{{{(x - 3)}^2} + 1}} \le 1 \Rightarrow \dfrac{5}{{{{(x - 3)}^2} + 1}} \le 5$
Vậy GTLN của phân thức là $5$.
Dấu “=” xảy ra khi \({\left( {x - 3} \right)^2} = 0\) hay \(x = 3\).

