Phân tích đa thức sau thành nhân tử: \({x^3} - 5x + 4\) ta được
\(\begin{array}{l}\,\,\,\,\,{x^3} - 5x + 4\\ = {x^3} - x - 4x + 4\\ = x\left( {{x^2} - 1} \right) - 4\left( {x - 1} \right)\\ = x\left( {x - 1} \right)\left( {x + 1} \right) - 4\left( {x - 1} \right)\\ = \left( {x - 1} \right)\left[ {x\left( {x + 1} \right) - 4} \right]\\ = \left( {x - 1} \right)\left( {{x^2} + x - 4} \right).\end{array}\)
Thực hiện phép tính: \(\left( {4{x^4} - 4{x^3} + 3x - 3} \right):\left( {x - 1} \right)\)
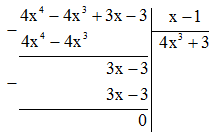
$\left( {4{x^4} - 4{x^3} + 3x - 3} \right):\left( {x - 1} \right) = 4{x^3} + 3$
Rút gọn biểu thức: $A = \dfrac{{4{x^3} - 5{x^2} + 1}}{{{x} - 1}}$
$\begin{array}{l}A = \dfrac{{4{x^3} - 5{x^2} + 1}}{{x - 1}}\\\,\,\,\,\,\, = \dfrac{{4{x^3} - 4{x^2} - {x^2} + 1}}{{x - 1}}\\\,\,\,\,\,\, = \dfrac{{4{x^2}\left( {x - 1} \right) - \left( {{x^2} - 1} \right)}}{{x - 1}}\\\,\,\,\,\,\, = \dfrac{{4{x^2}\left( {x - 1} \right) - \left( {x - 1} \right)\left( {x + 1} \right)}}{{x - 1}}\\\,\,\,\,\,\, = \dfrac{{\left( {x - 1} \right)\left( {4{x^2} - x - 1} \right)}}{{x - 1}} = 4{x^2} - x - 1.\end{array}$
Thực hiện phép tính \(A = \left( {6{x^3} - 5{x^2} + 4x - 1} \right):\left( {2{x^2} - x + 1} \right)\) ta được
\(\left( {6{x^3} - 5{x^2} + 4x - 1} \right):\left( {2{x^2} - x + 1} \right)\)
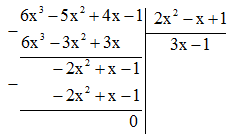
\(\left( {6{x^3} - 5{x^2} + 4x - 1} \right):\left( {2{x^2} - x + 1} \right) = 3x - 1.\)
Phân tích đa thức thành nhân tử ta được \({x^3} + 7{x^2} + 12x + 4 = \left( {x + 2} \right)\left( {{x^2} + a.x + 2} \right)\) . Khi đó giá trị của \(a\) là:
\(\begin{array}{l} + )\;{x^3} + 7{x^2} + 12x + 4\\ = {x^3} + 6{x^2} + {x^2} + 12x + 8 - 4\\ = \left( {{x^3} + 6{x^2} + 12x + 8} \right) + \left( {{x^2} - 4} \right)\\ = \left( {{x^3} + 3.2.{x^2} + {{3.2}^2}.x + {2^3}} \right) + \left( {{x^2} - 4} \right)\\ = {\left( {x + 2} \right)^3} + \left( {x - 2} \right)\left( {x + 2} \right)\\ = \left( {x + 2} \right)\left( {{{\left( {x + 2} \right)}^2} + x - 2} \right)\\ = \left( {x + 2} \right)\left( {{x^2} + 4x + 4 + x - 2} \right)\\ = \left( {x + 2} \right)\left( {{x^2} + 5x + 2} \right)\end{array}\)
Có bao nhiêu giá trị của $x$ thỏa mãn \(\,2{x^3}\left( {2x - 3} \right) - {x^2}\left( {4{x^2} - 6x + 2} \right) = 0\)
\(\begin{array}{l}2{x^3}\left( {2x - 3} \right) - {x^2}\left( {4{x^2} - 6x + 2} \right) = 0\\ \Leftrightarrow 4{x^4} - 6{x^3} - 4{x^4} + 6{x^3} - 2{x^2} = 0\\ \Leftrightarrow - 2{x^2} = 0\\ \Leftrightarrow x = 0\end{array}\)
Vậy $x = 0.$
Có \(1\) giá trị của \(x\) thỏa mãn đề bài.
Giá trị nhỏ nhất của biểu thức \(A = {x^2} - x + 1\) là:
\(\begin{array}{l}A = {x^2} - x + 1 = {x^2} - 2.x.\dfrac{1}{2} + {\left( {\dfrac{1}{2}} \right)^2} + \dfrac{3}{4} = {\left( {x - \dfrac{1}{2}} \right)^2} + \dfrac{3}{4} \ge \dfrac{3}{4}\\ \Rightarrow \;Min\;A = \dfrac{3}{4}\;\end{array}\)
Dấu “=” xảy ra khi \({\left( {x - \dfrac{1}{2}} \right)^2} = 0\) hay \(x = \dfrac{1}{2}\).
Giá trị lớn nhất của biểu thức \(B = - 9{x^2} + 2x - \dfrac{2}{9}\) là:
\(B = - 9{x^2} + 2x - \dfrac{2}{9} \)\(= - {\left( {3x} \right)^2} + 2.3x.\dfrac{1}{3} - {\left( {\dfrac{1}{3}} \right)^2} - \dfrac{1}{9} \)\(= - \left( {{{\left( {3x} \right)}^2} - 2.3x.\dfrac{1}{3} + {{\left( {\dfrac{1}{3}} \right)}^2}} \right) - \dfrac{1}{9} \)\(= - {\left( {3x - \dfrac{1}{3}} \right)^2} - \dfrac{1}{9} \le - \dfrac{1}{9}\)
\( \Rightarrow \;Max\;B = - \dfrac{1}{9}\).
Dấu “=” xảy ra khi \({\left( {3x - \dfrac{1}{3}} \right)^2} = 0\) hay \(x = \dfrac{1}{9}\).
Tính giá trị biểu thức \(P = \left( { - 4{x^3}{y^3} + {x^3}{y^4}} \right):2x{y^2} - xy\left( {2x - xy} \right)\) cho \(x = 1,y = \dfrac{{ - 1}}{2}\);
\(\begin{array}{l}\,\,\,\,\,\,\,\,P = \left( { - 4{x^3}{y^3} + {x^3}{y^4}} \right):2x{y^2} - xy\left( {2x - xy} \right)\\ \Leftrightarrow P = \left( { - 4{x^3}{y^3}} \right):2x{y^2} + {x^3}{y^4}:2x{y^2} - xy.2x + xy.xy\\ \Leftrightarrow P = - 2{x^2}y + \dfrac{1}{2}{x^2}{y^2} - 2{x^2}y + {x^2}{y^2}\\ \Leftrightarrow P = \dfrac{3}{2}{x^2}{y^2} - 4{x^2}y\\ \Leftrightarrow P = {x^2}y\left( {\dfrac{3}{2}y - 4} \right)\end{array}\)
Tại \(x = 1,y = \dfrac{{ - 1}}{2}\), ta có: \(P = {1^2}.\left( {\dfrac{{ - 1}}{2}} \right)\left( {\dfrac{3}{2}\left( {\dfrac{{ - 1}}{2}} \right) - 4} \right) = \left( {\dfrac{{ - 1}}{2}} \right)\left( {\dfrac{{ - 3}}{4} - 4} \right) = \left( {\dfrac{{ - 1}}{2}} \right)\left( {\dfrac{{ - 19}}{4}} \right) = \dfrac{{19}}{8}\)
Phân tích đa thức \({x^8} + {x^4} + 1\) thành nhân tử ta được
\(\begin{array}{l}{x^8} + {x^4} + 1\\ = {x^8} + 2{x^4} + 1 - {x^4}\\ = \left( {{x^8} + 2{x^4} + 1} \right) - {x^4}\\ = \left[ {{{\left( {{x^4}} \right)}^2} + 2.{x^4}.1 + {1^2}} \right] - {x^4}\\ = {\left( {{x^4} + 1} \right)^2} - {\left( {{x^2}} \right)^2}\\ = \left( {{x^4} + 1 - {x^2}} \right)\left( {{x^4} + 1 + {x^2}} \right)\\ = \left( {{x^4} - {x^2} + 1} \right)\left( {{x^4} + 2{x^2} - {x^2} + 1} \right)\end{array}\)
\(\begin{array}{l} = \left( {{x^4} - {x^2} + 1} \right)\left[ {\left( {{{\left( {{x^2}} \right)}^2} + 2.1.{x^2} + 1} \right) - {x^2}} \right]\\ = \left( {{x^4} - {x^2} + 1} \right)\left[ {{{\left( {{x^2} + 1} \right)}^2} - {x^2}} \right]\\ = \left( {{x^4} - {x^2} + 1} \right)\left( {{x^2} + 1 - x} \right)\left( {{x^2} + 1 + x} \right)\\ = \left( {{x^4} - {x^2} + 1} \right)\left( {{x^2} - x + 1} \right)\left( {{x^2} + x + 1} \right).\end{array}\)
Cho \(S = 1 + x + {x^2} + {x^3} + {x^4} + {x^5}\), chọn câu đúng
\(xS = x.(1 + x + {x^2} + {x^3} + {x^4} + {x^5}) = x + {x^2} + {x^3} + {x^4} + {x^5} + {x^6}.\)
\( \Rightarrow xS - S = x + {x^2} + {x^3} + {x^4} + {x^5} + {x^6} - 1 - x - {x^2} - {x^3} - {x^4} - {x^5} = {x^6} - 1.\)
Tìm giá trị nhỏ nhất của biểu thức \(A = {x^2} + 2{y^2} - 2xy + 2x - 10y\)
\(\begin{array}{l}\,\,\,\,\,\,\,\,A = {x^2} + 2{y^2} - 2xy + 2x - 10y\\ \Leftrightarrow A = {x^2} + {y^2} + 1 - 2xy + 2x - 2y + {y^2} - 8y + 16 - 17\\ \Leftrightarrow A = \left( {{x^2} + {y^2} + {1^2} - 2.x.y + 2.x.1 - 2.y.1} \right) + \left( {{y^2} - 2.4.y + {4^2}} \right) - 17\\ \Leftrightarrow A = {\left( {x - y + 1} \right)^2} + {\left( {y - 4} \right)^2} - 17.\end{array}\)
Vì \(\left\{ \begin{array}{l}{\left( {x - y + 1} \right)^2} \ge 0\\{\left( {y - 4} \right)^2} \ge 0\end{array} \right.\) với mọi $x;y$ nên \(A \ge - 17\) với mọi $x;y.$
\( \Rightarrow A = - 17 \Leftrightarrow \left\{ \begin{array}{l}x - y + 1 = 0\\y - 4 = 0\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x = y - 1\\y = 4\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x = 3\\y = 4\end{array} \right.\)
Vậy $A$ đạt giá trị nhỏ nhất là \(A = - 17\) tại \(\left\{ \begin{array}{l}x = 3\\y = 4\end{array} \right.\).
Cho: \({a^3} + {b^3} + {c^3} = 3abc\) thì
Từ đẳng thức đã cho suy ra \({a^3} + {b^3} + {c^3} - 3abc = 0\)
${b^3} + {c^3} = \left( {b + c} \right)\left( {{b^2} + {c^2} - bc} \right) $$= \left( {b + c} \right)\left[ {{{\left( {b + c} \right)}^2} - 3bc} \right] $$= {\left( {b + c} \right)^3} - 3bc\left( {b + c} \right)$
$ \Rightarrow {a^3} + {b^3} + {c^3} - 3abc $$= {a^3} + \left( {{b^3} + {c^3}} \right) - 3abc $
$ \Leftrightarrow {a^3} + {b^3} + {c^3} - 3abc= {a^3} + {\left( {b + c} \right)^3} - 3bc\left( {b + c} \right) - 3abc$$ \Leftrightarrow {a^3} + {b^3} + {c^3} - 3abc $$= \left( {a + b + c} \right)\left( {{a^2} - a\left( {b + c} \right) + {{\left( {b + c} \right)}^2}} \right) - \left[ {3bc\left( {b + c} \right) + 3abc} \right]$$ \Leftrightarrow {a^3} + {b^3} + {c^3} - 3abc = \left( {a + b + c} \right)\left( {{a^2} - a\left( {b + c} \right) + {{\left( {b + c} \right)}^2}} \right) - 3bc\left( {a + b + c} \right)$$ \Leftrightarrow {a^3} + {b^3} + {c^3} - 3abc = \left( {a + b + c} \right)\left( {{a^2} - a\left( {b + c} \right) + {{\left( {b + c} \right)}^2} - 3bc} \right)$$ \Leftrightarrow {a^3} + {b^3} + {c^3} - 3abc = \left( {a + b + c} \right)\left( {{a^2} - ab - ac + {b^2} + 2bc + {c^2} - 3bc} \right)$$ \Leftrightarrow {a^3} + {b^3} + {c^3} - 3abc = \left( {a + b + c} \right)\left( {{a^2} + {b^2} + {c^2} - ab - ac - bc} \right)$
Do đó nếu \({a^3} + {b^3} + {c^3} - 3abc = 0\) thì \(a + b + c = 0\) hoặc \({a^2} + {b^2} + {c^2} - ab - bc - ac = 0\)
Mà \({a^2} + {b^2} + {c^2} - ab - bc - ac = \dfrac{1}{2}.\left[ {{{\left( {a - b} \right)}^2} + {{\left( {a - c} \right)}^2} + {{\left( {b - c} \right)}^2}} \right]\) suy ra \(a = b = c\).

