Tìm \(m\) để bất phương trình \(\sqrt {x - {m^2} - m} \left( {3 - \dfrac{{x + 1}}{{{x^3} - {x^2} - 3x + 3}}} \right) < 0\,\,(*)\) có nghiệm .
Ta có: \(\left( * \right) \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{3 - \dfrac{{x + 1}}{{{x^3} - {x^2} - 3x + 3}} < 0}\\{x > {m^2} + m}\end{array}} \right.\)\( \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{\dfrac{{\left( {x - 2} \right)\left( {3{x^2} + 3x - 4} \right)}}{{\left( {x - 1} \right)\left( {{x^2} - 3} \right)}} < 0}\\{x > {m^2} + m}\end{array}} \right.\) \(\left( {**} \right)\)
Bảng xét dấu:
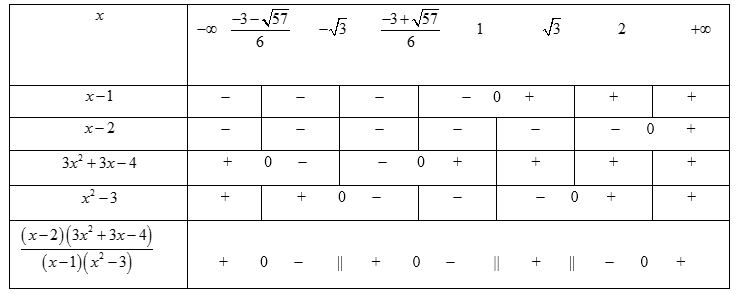
Tập nghiệm của bất phương trình \(\dfrac{{\left( {x - 2} \right)\left( {3{x^2} + 3x - 4} \right)}}{{\left( {x - 1} \right)\left( {{x^2} - 3} \right)}} < 0\) là \(S = \left( {\dfrac{{ - 3 - \sqrt {57} }}{6}; - \sqrt 3 } \right) \cup \left( {\dfrac{{ - 3 + \sqrt {57} }}{6};1} \right) \cup \left( {\sqrt 3 ;2} \right)\)
Do đó bất phương trình \(\left( * \right)\) có nghiệm khi và chỉ khi hệ bất phương trình\(\left( {**} \right)\) có nghiệm
\( \Leftrightarrow {m^2} + m < 2\)\( \Leftrightarrow {m^2} + m - 2 < 0\)\( \Leftrightarrow - 2 < m < 1\)
Vậy \( - 2 < m < 1\) là giá trị cần tìm.
Bất phương trình $\left| {x + 2} \right| - \left| {x - 1} \right| < x - \dfrac{3}{2}$ có tập nghiệm là
Xét bất phương trình $\left| {x + 2} \right| - \left| {x - 1} \right| \le x - \dfrac{3}{2}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left( * \right).$
Lập bảng xét dấu
TH1. Với $x < - \,2,$ khi đó $\left( * \right) \Leftrightarrow - \,x - 2 + x - 1 < x - \dfrac{3}{2} \Leftrightarrow x > - \dfrac{3}{2}.$
Kết hợp với điều kiện $x < - \,2,$ ta được tập nghiệm ${S_1} = \emptyset .$
TH2. Với $ - \,2 \le x < 1,$ khi đó $\left( * \right) \Leftrightarrow x + 2 + x - 1 < x - \dfrac{3}{2} \Leftrightarrow x < - \dfrac{5}{2}.$
Kết hợp với điều kiện $ - \,2 \le x < 1,$ ta được tập nghiệm ${S_2} = \emptyset .$
TH3. Với $x \ge 1,$ khi đó $\left( * \right) \Leftrightarrow x + 2 - x + 1 < x - \dfrac{3}{2} \Leftrightarrow x > \dfrac{9}{2}.$
Kết hợp với điều kiện $x \ge 1,$ ta được tập nghiệm ${S_3} = \left( {\dfrac{9}{2}; + \,\infty } \right).$
Vậy tập nghiệm của bất phương trình là $S = {S_1} \cup {S_2} \cup {S_3} = \left( {\dfrac{9}{2}; + \,\infty } \right).$
Một viên gạch hình vuông có cạnh thay đổi được đặt nội tiếp trong một hình vuông có cạnh bằng 20cm, tạo thành bốn tam giác xung quanh như hình vẽ.
Tìm tập hợp các giá trị của x để diện tích viên gạch không vượt quá \(208c{m^2}\).
Ta có: \(\angle CAB + \angle BAD + \angle DAE = {180^o}\)
\( \Rightarrow \angle CAB + \angle EAD = {90^o}\)
Mà \(\angle CAB + \angle CBA = {90^o}\) (\(\Delta CAB\) vuông tại C)
\( \Rightarrow \angle CBA = \angle EAD\) kết hợp \(AB = AD\,\,\,\left( {gt} \right)\)
\(\begin{array}{l} \Rightarrow \Delta CAB = \Delta EDA\,\,\,\left( {ch - gn} \right)\\ \Rightarrow CB = EA = x \Rightarrow CA = CE - EA = 20 - x\,\,\,\,\left( {cm} \right)\end{array}\)
Diện tích viên gạch là \(S = A{B^2} = C{B^2} + C{A^2} = {x^2} + {\left( {20 - x} \right)^2}\)
Vì \(S \le 208 \Leftrightarrow {x^2} + {\left( {20 - x} \right)^2} \le 208 \Leftrightarrow 2{x^2} - 40x + 192 \le 0 \Leftrightarrow 8 \le x \le 12\)Tập nghiệm của bất phương trình \(\left( {\sqrt {2x + 4} - \sqrt {x + 1} } \right)\left( {\sqrt {2x + 1} + \sqrt {x + 4} } \right) \le x + 3\) là tập con của tập hợp nào sau đây?
ĐKXĐ: \(x \ge - \dfrac{1}{2}\)
\(\begin{array}{l}\,\,\,\,\,\,\,\,\left( {\sqrt {2x + 4} - \sqrt {x + 1} } \right)\left( {\sqrt {2x + 1} + \sqrt {x + 4} } \right) \le x + 3\\ \Leftrightarrow \left( {\sqrt {2x + 4} - \sqrt {x + 1} } \right)\left( {\sqrt {2x + 4} + \sqrt {x + 1} } \right)\left( {\sqrt {2x + 1} + \sqrt {x + 4} } \right) \le \left( {x + 3} \right)\left( {\sqrt {2x + 4} + \sqrt {x + 1} } \right)\\ \Leftrightarrow \left( {x + 3} \right)\left( {\sqrt {2x + 1} + \sqrt {x + 4} } \right) \le \left( {x + 3} \right)\left( {\sqrt {2x + 4} + \sqrt {x + 1} } \right)\\ \Leftrightarrow \left( {x + 3} \right)\left( {\sqrt {2x + 1} + \sqrt {x + 4} - \sqrt {2x + 4} - \sqrt {x + 1} } \right) \le 0\\ \Leftrightarrow \sqrt {2x + 1} + \sqrt {x + 4} - \sqrt {2x + 4} - \sqrt {x + 1} \le 0\,\,\,\,\,\left( {do\,\,\,x + 3 > 0\,\,\,\forall x \ge - \dfrac{1}{2}} \right)\\ \Leftrightarrow \sqrt {2x + 1} + \sqrt {x + 4} \le \sqrt {2x + 4} + \sqrt {x + 1} \\ \Leftrightarrow 3x + 5 + 2\sqrt {\left( {2x + 1} \right)\left( {x + 4} \right)} \le 3x + 5 + 2\sqrt {\left( {2x + 4} \right)\left( {x + 1} \right)} \\ \Leftrightarrow \left( {2x + 1} \right)\left( {x + 4} \right) \le \left( {2x + 4} \right)\left( {x + 1} \right)\\ \Leftrightarrow 2{x^2} + 9x + 4 \le 2{x^2} + 6x + 4\\ \Leftrightarrow 3x \le 0 \Leftrightarrow x \le 0\end{array}\)
Kết hợp ĐKXĐ \( \Rightarrow x \in \left[ { - \dfrac{1}{2};0} \right] \subset \left( { - \dfrac{2}{3};\dfrac{1}{2}} \right)\)
Bạn An chọn một số nguyên, nhân số đó với 4 rồi trừ đi 30. Lấy kết quả có được nhân với 2 và cuối cùng trừ đi 10 thì được một số có hai chữ số. Số lớn nhất An có thể chọn được có hàng đơn vị bằng:
Gọi số nguyên lớn nhất bạn An có thể chọn là \(x\) \(\left( {x \in \mathbb{Z}} \right)\).
Theo bài ra ta có \(2\left( {4x - 30} \right) - 10\) là số có 2 chữ số.
\(\begin{array}{l} \Rightarrow \left[ \begin{array}{l}10 \le 2\left( {4x - 30} \right) - 10 \le 99\\ - 99 \le 2\left( {4x - 30} \right) - 10 \le - 10\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}20 \le 2\left( {4x - 30} \right) \le 109\\ - 89 \le 2\left( {4x - 30} \right) \le 0\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}10 \le 4x - 30 \le \dfrac{{109}}{2}\\ - \dfrac{{89}}{2} \le 4x - 30 \le 0\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}40 \le 4x \le \dfrac{{169}}{2}\\ - \dfrac{{29}}{2} \le 4x \le 30\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}10 \le x \le \dfrac{{169}}{8}\\ - \dfrac{{29}}{8} \le x \le \dfrac{{30}}{4}\end{array} \right.\end{array}\)
Vì \(x \in \mathbb{Z}\) và \(x\) là số lớn nhất nên \(x = 21\).
Vậy số lớn nhất An có thể chọn có hàng đơn vị bằng 1.
