Tập tất cả các giá trị của tham số \(a\) để hàm số \(y = {\left( {a - 2} \right)^x}\) nghịch biến trên \(\mathbb{R}\) là:
Hàm số \(y = {\left( {a - 2} \right)^x}\) nghịch biến trên \(\mathbb{R}\) khi và chỉ khi \(0 < a - 2 < 1 \Leftrightarrow 2 < a < 3\).
Vậy tập các giá trị của tham số \(a\) để hàm số đã cho nghịch biến trên \(\mathbb{R}\) là \(\left( {2;3} \right)\).
Hàm số nào sau đây nghịch biến trên \(\left( { - \infty ; + \infty } \right)\)?
Do \(0 < \dfrac{2}{e} < 1\) nên hàm số y =\({\left( {\dfrac{2}{e}} \right)^x}\) nghịch biến trên \(\left( { - \infty ; + \infty } \right)\).
Tính đạo hàm của hàm số \(y = {6^x}\).
\(y = {6^x} \Rightarrow \)\(y' = {6^x}\ln 6.\)
Cho hàm số \(y = {e^{2x}} - x\). Chọn khẳng định đúng.
TXĐ: \(D = \mathbb{R}\).
Ta có: \(y' = 2{e^{2x}} - 1 = 0 \Leftrightarrow {e^{2x}} = \dfrac{1}{2}\) \( \Leftrightarrow 2x = \ln \dfrac{1}{2} = - \ln 2 \Leftrightarrow x = - \dfrac{1}{2}\ln 2 = - \ln \sqrt 2 \).
BBT:
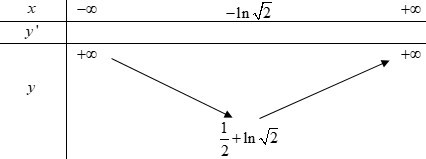
Dựa vào BBT ta thấy hàm số đồng biến trên \(\left( { - \ln \sqrt 2 ; + \infty } \right)\).
Tìm tất cả các giá trị thực của \(m\) để hàm số \(y = {2^{{x^3} - {x^2} + m\,x + 1}}\) đồng biến trên \(\left( {1;2} \right)\)
Ta có: \(y = {2^{{x^3} - {x^2} + mx + 1}}\) \( \Rightarrow y' = \left( {3{x^2} - 2x + m} \right){2^{{x^3} - {x^2} + mx + 1}}\)
\( \Rightarrow \) Hàm số đã cho đồng biến trên \(\left( {1;\,\,2} \right) \Leftrightarrow y' \ge 0\,\,\forall x \in \left( {1;\,\,2} \right)\)
\(\begin{array}{l} \Leftrightarrow \left( {3{x^2} - 2x + m} \right){2^{{x^3} - {x^2} + mx + 1}} \ge 0\,\,\,\forall x \in \left( {1;\,\,2} \right)\\ \Leftrightarrow 3{x^2} - 2x + m \ge \,0\,\,\,\forall x \in \left( {1;\,\,2} \right)\\ \Leftrightarrow \left[ \begin{array}{l}\Delta ' \le 0\\\left\{ \begin{array}{l}\Delta ' \ge 0\\\left[ \begin{array}{l}{x_1} < {x_2} \le 1\\2 \le {x_1} < {x_2}\end{array} \right.\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}\Delta ' \le 0\\\left\{ \begin{array}{l}\Delta ' \ge 0\\\left[ \begin{array}{l}\left\{ \begin{array}{l}{x_1} + {x_2} < 2\\\left( {{x_1} - 1} \right)\left( {{x_2} - 1} \right) \ge 0\end{array} \right.\\\left\{ \begin{array}{l}{x_1} + {x_2} > 4\\\left( {{x_1} - 2} \right)\left( {{x_2} - 2} \right) \ge 0\end{array} \right.\end{array} \right.\end{array} \right.\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}\Delta ' \le 0\\\left\{ \begin{array}{l}\Delta ' \ge 0\\\left[ \begin{array}{l}\left\{ \begin{array}{l}{x_1} + {x_2} < 2\\{x_1}{x_2} - \left( {{x_1} + {x_2}} \right) + 1 \ge 0\end{array} \right.\\\left\{ \begin{array}{l}{x_1} + {x_2} > 4\\{x_1}{x_2} - 2\left( {{x_1} + {x_2}} \right) + 4 \ge 0\end{array} \right.\end{array} \right.\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}1 - 3m \le 0\\\left\{ \begin{array}{l}1 - 3m \ge 0\\\left[ \begin{array}{l}\frac{2}{3} < 2\\\frac{m}{3} - \frac{2}{3} + 1 \ge 0\end{array} \right.\\\left[ \begin{array}{l}\frac{x}{3} > 4\,\,\,\left( {ktm} \right)\\\frac{m}{3} - \frac{4}{3} + 4 \ge 0\end{array} \right.\end{array} \right.\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}m \ge \frac{1}{3}\\\left\{ \begin{array}{l}m \le \frac{1}{3}\\\frac{m}{3} \ge - \frac{1}{3}\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}m \ge \frac{1}{3}\\\left\{ \begin{array}{l}m \le \frac{1}{3}\\m \ge - 1\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}m \ge \frac{1}{3}\\ - 1 \le m \le \frac{1}{3}\end{array} \right. \Leftrightarrow m \ge - 1.\end{array}\)
Cho hàm số \(f\left( x \right) = \dfrac{1}{{3 + {2^x}}} + \dfrac{1}{{3 + {2^{ - x}}}}\). Trong các khẳng định, có bao nhiêu khẳng định đúng?
1) \(f'\left( x \right) \ne 0,\forall x \in R\)
2) \(f\left( 1 \right) + f\left( 2 \right) + ... + f\left( {2017} \right) = 2017\)
3) \(f\left( {{x^2}} \right) = \dfrac{1}{{3 + {4^x}}} + \dfrac{1}{{3 + {4^{ - x}}}}\)
Ta có:
\(f'\left( x \right) = \dfrac{{ - {2^x}\ln 2}}{{{{\left( {3 + {2^x}} \right)}^2}}} + \dfrac{{{2^{ - x}}\ln 2}}{{{{\left( {3 + {2^{ - x}}} \right)}^2}}} \Rightarrow f'\left( 0 \right) = 0\) nên khẳng định (1) sai.
\(f\left( x \right) = \dfrac{{{2^x} + {2^{ - x}} + 6}}{{\left( {3 + {2^x}} \right)\left( {3 + {2^{ - x}}} \right)}} = \dfrac{{{2^x} + {2^{ - x}} + 6}}{{3\left( {{2^x} + {2^{ - x}}} \right) + 10}}\)
Đặt \(t = {2^x} + {2^{ - x}} \ge 2\sqrt {{2^x}{{.2}^{ - x}}} = 2\) thì \(\dfrac{{{2^x} + {2^{ - x}} + 6}}{{3\left( {{3^x} + {2^{ - x}}} \right) + 10}} = \dfrac{{t + 6}}{{3t + 10}}\)
Xét \(g\left( t \right) = \dfrac{{t + 6}}{{3t + 10}},g'\left( t \right) = - \dfrac{8}{{{{\left( {3t + 10} \right)}^2}}} < 0\) nên hàm số nghịch biến trên \(\left[ {2; + \infty } \right)\).
\( \Rightarrow g\left( t \right) \le g\left( 2 \right) = \dfrac{{2 + 6}}{{3.2 + 10}} = \dfrac{1}{2} < 1\) hay \(f\left( x \right) < 1,\forall x\).
Suy ra \(f\left( 1 \right) < 1,f\left( 2 \right) < 1,...,f\left( {2017} \right) < 1\).
\( \Rightarrow f\left( 1 \right) + f\left( 2 \right) + ... + f\left( {2017} \right) < 2017\) nên (2) sai.
\(f\left( {{x^2}} \right) = \dfrac{1}{{3 + {2^{{x^2}}}}} + \dfrac{1}{{3 + {2^{ - {x^2}}}}} \ne \dfrac{1}{{3 + {4^x}}} + \dfrac{1}{{3 + {4^{ - x}}}}\) (chẳng hạn \(x = 1\)) nên (3) sai.
Do đó không có khẳng định nào đúng.
Cho hàm số \(f(x) = {(3 - \sqrt 2 )^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}}\) . Xét các khẳng định sau:
Khẳng định 1: \(f(x) > 0 \Leftrightarrow {x^3} + {x^2} > 0\)
Khẳng định 2: \(f(x) > 0 \Leftrightarrow x > - 1\).
Khẳng định 3: \(f(x) < 3 - \sqrt 2 \Leftrightarrow {(3 - \sqrt 2 )^{{x^3} - 1}} < 1 + {\left( {\dfrac{{3 + \sqrt 2 }}{7}} \right)^{{x^2} + 1}}\)
Khẳng định 4:\(f(x) < 3 + \sqrt 2 \Leftrightarrow {(3 - \sqrt 2 )^{{x^3} + 1}} < {(3 - \sqrt 2 )^{1 - {x^2}}} + 7\)
Trong các khẳng định trên, có bao nhiêu khẳng định đúng?
Cơ số $3 - \sqrt 2 > 1$
Ta có $f\left( x \right) > 0 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} > 0 \Leftrightarrow {x^3} > - {x^2} \Leftrightarrow {x^3} + {x^2} > 0$ suy ra khẳng định 1 đúng.
Ta có $f\left( x \right) > 0 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} > 0 \Leftrightarrow {x^3} > - {x^2} \Leftrightarrow {x^3} + {x^2} > 0 $
$\Leftrightarrow {x^2}\left( {x + 1} \right) > 0 \Leftrightarrow \left\{ \begin{gathered}
x > - 1 \hfill \\
x \ne 0 \hfill \\
\end{gathered} \right.$ suy ra khẳng định 2 sai.
Ta có
$\begin{array}{l}f\left( x \right) < 3 - \sqrt 2 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} < 3 - \sqrt 2 \\ \Leftrightarrow \dfrac{{{{\left( {3 - \sqrt 2 } \right)}^{{x^3}}}}}{{3 - \sqrt 2 }} - \dfrac{{{{\left( {3 - \sqrt 2 } \right)}^{ - {x^2}}}}}{{3 - \sqrt 2 }} < 1 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} - 1}} < 1 + {\left( {3 - \sqrt 2 } \right)^{ - {x^2} - 1}} \\ \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} - 1}} < 1 + {\left( {\dfrac{1}{{3 - \sqrt 2 }}} \right)^{{x^2} + 1}} \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} - 1}} < 1 + {\left( {\dfrac{{3 + \sqrt 2 }}{7}} \right)^{{x^2} + 1}}\end{array}$
suy ra khẳng định 3 đúng.
Ta có
$\begin{array}{l}f\left( x \right) < 3 + \sqrt 2 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} < 3 + \sqrt 2 \\ \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}}\left( {3 - \sqrt 2 } \right) - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}}\left( {3 - \sqrt 2 } \right) < \left( {3 + \sqrt 2 } \right)\left( {3 - \sqrt 2 } \right)\\ \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} + 1}} < {\left( {3 - \sqrt 2 } \right)^{1 - {x^2}}} + 7\end{array}$
Suy ra khẳng định 4 đúng.
Vậy có $3$ khẳng định đúng.
Cho hai số thực dương $x, y$ thỏa mãn \({2^x} + {2^y} = 4\). Tìm giá trị lớn nhất \({P_{\max }}\) của biểu thức\(P = (2{x^2} + y)(2{y^2} + x) + 9xy\).
Ta có:
\(\begin{array}{l}4 = {2^x} + {2^y} \ge 2\sqrt {{2^x}{{.2}^y}} \Rightarrow 2 \ge \sqrt {{2^x}{2^y}} \\ \Rightarrow 4 \ge {2^{x + y}} \Rightarrow 0 < x + y \le 2\\ \Rightarrow {\left( {x + y} \right)^2} \le 4\end{array}\)
Lại có \(x + y \ge 2\sqrt {xy} \Rightarrow xy \le 1\)
\(\begin{array}{l} \Rightarrow P = 4{x^2}{y^2} + 2{x^3} + 2{y^3} + 10xy\\ = 4{\left( {xy} \right)^2} + 10xy + 2\left( {{x^3} + {y^3}} \right)\\ = 4{\left( {xy} \right)^2} + 10xy \\+ 2.\left( {x + y} \right).\left[ {{{\left( {x + y} \right)}^2} - 3xy} \right]\\ \Rightarrow P \le 4{\left( {xy} \right)^2} + 10xy + 2.2.\left( {4 - 3xy} \right)\\ \Rightarrow P \le 4{\left( {xy} \right)^2} - 2xy + 16\end{array}\)
Đặt \(xy = t \Rightarrow 0 < t \le 1\)
Xét hàm số \(f\left( t \right) = 4{t^2} - 2t + 16\) trên \(\left( {0;1} \right]\).
\( \Rightarrow f\left( t \right) \le \max \left\{ {f\left( 1 \right),f\left( 0 \right)} \right\} = 18\).
Dấu “=” xảy ra khi và chỉ khi \(x = y = 1\).
Vậy \({P_{\max }} = 18 \Leftrightarrow x = y = 1\).

