Tập nghiệm của bất phương trình 9log29x+xlog9x≤18 là:
ĐKXĐ: x>0.
Ta có:
9log29x+xlog9x≤18⇔9log9x.log9x+xlog9x≤18⇔(9log9x)log9x+xlog9x≤18⇔xlog9x+xlog9x≤18⇔2.xlog9x≤18⇔xlog9x≤9
Lấy logarit cơ số 9 cả 2 vế bất phương trình ta được:
log9(xlog9x)≤log99⇔log9x.log9x≤1⇔log29x≤1⇔−1≤log9x≤1⇔19≤x≤9
Kết hợp điều kiện xác định ta có x∈[19;9].
Vậy tập nghiệm của bất phương trình là: S=[19;9].
Tập nghiệm của bất phương trìnhlog2(x√x2+2+4−x2)+2x+√x2+2≤1 là (−√a;−√b]. Khi đó ab bằng
Điều kiện : x√x2+2+4−x2>0⇔x(√x2+2−x)+4>0⇔x.2√x2+2+x+4>0
⇔2x√x2+2+x+4(√x2+2+x)√x2+2+x>0⇒6x+4√x2+2>0 (vì √x2+2>x;∀x )
⇔2√x2+2>−3x⇔[−3x<0{−3x≥04(x2+2)>(−3x)2⇔[x>0{x≤05x2<8⇔[x>0−√405<x≤0
Khi đó ta có log2(x√x2+2+4−x2)+2x+√x2+2≤1
⇔log2(x(√x2+2−x)+4)+2x+√x2+2≤1⇔log2(2x√x2+2+x+4)+2x+√x2+2≤1⇔log2(6x+4√x2+2√x2+2+x)+2x+√x2+2≤1
⇔log2(6x+4√x2+2)−log2(√x2+2+x)+2x+√x2+2≤1⇔log2[2(3x+2√x2+2)]−log2(√x2+2+x)+2x+√x2+2≤1⇔log22+log2(3x+2√x2+2)−log2(√x2+2+x)+2x+√x2+2≤1⇔1+log2(3x+2√x2+2)−log2(√x2+2+x)+2x+√x2+2≤1⇔log2(3x+2√x2+2)+3x+2√x2+2≤log2(√x2+2+x)+x+√x2+2(∗)
Xét hàm số f(t)=t+log2t với t>0 ta có f′(t)=1+1t.ln2>0;∀t>0 nên f(t) là hàm đồng biến trên (0;+∞)
Từ đó
(∗)⇔f(3x+2√x2+2)≤f(√x2+2+x)⇔3x+2√x2+2≤√x2+2+x⇔√x2+2≤−2x⇔{−2x≥0x2+2≤4x2⇔{x≤03x2≥2⇔{x≤0[x≥√63x≤−√63⇔x≤−√63
Kết hợp điều kiện [x>0−√405<x≤0 ta có −√405<x≤−√63 hay −√85<x≤−√23
Tập nghiệm bất phương trình S=(−√85;−√23] nên a=85;b=23⇒a.b=85.23=1615.
Cho hàm số f(x) liên tục trên R và có đồ thị f′(x) như hình vẽ bên. Bất phương trình log5[f(x)+m+2]+f(x)>4−m đúng với mọi x∈(−1;4) khi và chỉ khi
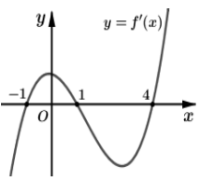
ĐK : f(x)+m+2>0
Ta có log5(f(x)+m+2)+f(x)>4−m ⇔log5(f(x)+m+2)+f(x)+m+2>6 (*)
Xét hàm số y=log5t+t(t>0) có y′=1t.ln5+1>0 với t>0
Nên hàm số y=log5t+t đồng biến trên (0;+∞), lại có y(5)=log55+5=6
Nên từ (*) suy ra y(f(x)+m+2)>y(5)⇔f(x)+m+2>5⇔f(x)>3−m (1)
Từ hình vẽ ta có BBT của hàm số f(x) như sau
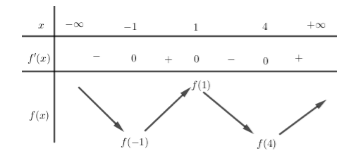
Từ hình vẽ ta có 1∫−1|f′(x)|dx<4∫1|f′(x)|dx⇔1∫−1f′(x)dx<−4∫1f′(x)dx
⇔f(x)|1−1<−f(x)|41⇔f(1)−f(−1)<f(1)−f(4) ⇔f(−1)>f(4) (2)
Từ (1) ; (2) và BBT ta thấy để phương trình đã cho đúng với x∈(−1;4) suy ra 3−m≤f(4)⇔m≥3−f(4).
Cho phương trình log7(x2+2x+2)+1>log7(x2+6x+5+m). Có tất cả bao nhiêu giá trị nguyên của tham số m để bất phương trình trên có tập nghiệm chứa khoảng (1;3)?
ĐK: x2+6x+5+m>0.
log7(x2+2x+2)+1>log7(x2+6x+5+m)⇔log77(x2+2x+2)>log7(x2+6x+5+m)⇔7(x2+2x+2)>x2+6x+5+m⇔7x2+14x+14−x2−6x−5−m>0⇔6x2+8x+9−m>0
Bất phương trình đã cho có tập nghiệm chứa (1;3)
⇔{x2+6x+5+m>0,∀x∈(1;3)6x2+8x+9−m>0,∀x∈(1;3)⇔{m>−x2−6x−5,∀x∈(1;3)m<6x2+8x+9,∀x∈(1;3)(∗)
⇔{m≥max
với f\left( x \right) = - {x^2} - 6x - 5 và g\left( x \right) = 6{x^2} + 8x + 9
Ta có:
f'\left( x \right) = - 2x - 6 = 0 \Leftrightarrow x = - 3 \notin \left( {1;3} \right) và f'\left( x \right) < 0,\forall x \in \left( {1;3} \right) nên hàm số y = f\left( x \right) nghịch biến trên \left( {1;3} \right)
\Rightarrow \mathop {\max }\limits_{\left[ {1;3} \right]} f\left( x \right) = f\left( 1 \right) = - 12 \Rightarrow m \ge - 12
g'\left( x \right) = 12x + 8 = 0 \Leftrightarrow x = - \frac{2}{3} \notin \left( {1;3} \right) và g'\left( x \right) > 0,\forall x \in \left( {1;3} \right) nên hàm số y = g\left( x \right) đồng biến trên \left( {1;3} \right)
\Rightarrow \mathop {\min }\limits_{\left[ {1;3} \right]} g\left( x \right) = g\left( 1 \right) = 23 \Rightarrow m \le 23
Vậy - 12 \le m \le 23.
Mà m \in \mathbb{Z} \Rightarrow m \in \left\{ { - 12; - 11;...;23} \right\} hay có 23 - \left( { - 12} \right) + 1 = 36 giá trị.
Cho hàm số y = f\left( x \right). Hàm số y = f'\left( x \right) có đồ thị như hình bên. Biết f\left( { - 1} \right) = 1,f\left( { - \dfrac{1}{e}} \right) = 2. Tìm tất cả các giá trị của m để bất phương trình f\left( x \right) < \ln \left( { - x} \right) + m nghiệm đúng với mọi x \in \left( { - 1; - \dfrac{1}{e}} \right).
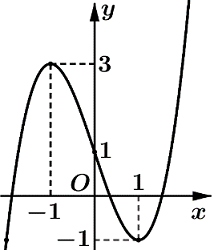
ĐKXĐ: - x > 0 \Leftrightarrow x < 0.
Ta có: f\left( x \right) < \ln \left( { - x} \right) + m \Leftrightarrow m > f\left( x \right) - \ln \left( { - x} \right) (*)
Xét hàm số g\left( x \right) = f\left( x \right) - \ln \left( { - x} \right) trên khoảng \left( { - 1; - \dfrac{1}{e}} \right) có:
\,g'\left( x \right) = f'\left( x \right) - \dfrac{{ - 1}}{{ - x}} = f'\left( x \right) - \dfrac{1}{x}
Ta biểu diễn đồ thị hàm số y = \dfrac{1}{x} (nét màu đỏ) trên hình vẽ như sau:
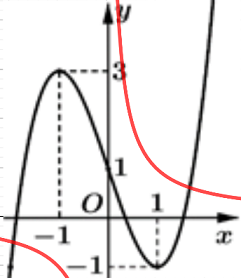
Quan sát đồ thị hàm số ta thấy
\,g'\left( x \right) = f'\left( x \right) - \dfrac{1}{x} > 0,\,\,\forall x \in \left( { - 1; - \dfrac{1}{e}} \right) \Rightarrow Hàm số y = g\left( x \right) đồng biến trên \left( { - 1; - \dfrac{1}{e}} \right).
Ta có: \left\{ \begin{array}{l}g\left( { - 1} \right) = f\left( { - 1} \right) - \ln \left( 1 \right) = 1\\g\left( { - \dfrac{1}{e}} \right) = f\left( { - \dfrac{1}{e}} \right) - \ln \dfrac{1}{e} = 2 + 1 = 3\end{array} \right. .
Để (*) nghiệm đúng với mọi x \in \left( { - 1; - \dfrac{1}{e}} \right) thì \Leftrightarrow m \ge \mathop {max}\limits_{\left[ { - 1; - \dfrac{1}{e}} \right]} g\left( x \right) \Leftrightarrow m \ge 3.
Tập nghiệm của bất phương trình {\log _{\frac{2}{3}}}\,\left( {3x - 2} \right) > \,{\log _{\frac{2}{3}}}\left( {2x+ 1} \right) là
\begin{array}{l}\,\,\,\,\,\,{\log _{\dfrac{2}{3}}}\left( {3x - 2} \right) > {\log _{\dfrac{2}{3}}}\left( {2x + 1} \right)\\ \Leftrightarrow 0 < 3x - 2 < 2x + 1\\ \Leftrightarrow \left\{ \begin{array}{l}x > \dfrac{2}{3}\\x - 3 < 0\end{array} \right. \Leftrightarrow \dfrac{2}{3} < x < 3\end{array}
Vậy tập nghiệm của bất phương trình đã cho là: S = \left( {\dfrac{2}{3};\,\,3} \right).
Xét các số thực không âm a,\,b thỏa mãn 2a + b \le {\log _2}\left( {2a + b} \right) + 1. Giá trị nhỏ nhất của {a^2} + {b^2} bằng bao nhiêu?
Đáp án:
Đáp án:
Bước 1: Đặt t = 2a + b\;\left( {t \ge 0} \right), đưa bất phương trình về dạng f\left( t \right) \ge 0
Đặt t = 2a + b\;\left( {t \ge 0} \right), ta có giả thiết đã cho tương đương với f\left( t \right) = {\log _2}t - t + 1 \ge 0
Ta có f'\left( t \right) = \dfrac{1}{{t\ln 2}} - 1 > 0 \Leftrightarrow t < \dfrac{1}{{\ln 2}} . Hàm số đồng biến trên \left( {0;\dfrac{1}{{\ln 2}}} \right)
Bước 2: Chứng minh t \ge 1.
Ta chứng minh t \ge 1 .
Thật vậy, giả sử t < 1 thì f\left( t \right) < f\left( 1 \right) = 0 (mâu thuẫn)
Vậy 2a + b \ge 1
Áp dụng BĐT Cauchy – Schwarz ta có
\begin{array}{l}{\left( {2a + b} \right)^2} \le \left( {{2^2} + {1^2}} \right)\left( {{a^2} + {b^2}} \right) = 5\left( {{a^2} + {b^2}} \right)\\ \Rightarrow {a^2} + {b^2} \ge \dfrac{{{{\left( {2a + b} \right)}^2}}}{5} \ge \dfrac{1}{5}\end{array}
Dấu bằng xảy ra \left\{ \begin{array}{l}2a + b = 1\\\dfrac{a}{2} = \dfrac{b}{1}\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}a = \dfrac{2}{5}\\b = \dfrac{1}{5}\end{array} \right.
Có tất cả bao nhiêu giá trị nguyên của y sao cho tương ứng với mọi y luôn tồn tại không quá 63 số nguyên x thỏa mãn điều kiện {\log _{2020}}\left( {x + {y^2}} \right) + {\log _{2021}}\left( {{y^2} + y + 64} \right) \ge {\log _4}\left( {x - y} \right).
Đáp án:
Đáp án:
Bước 1: Đặt f\left( x \right) = {\log _{2020}}\left( {x + {y^2}} \right) + {\log _{2021}}\left( {{y^2} + y + 64} \right) - {\log _4}\left( {x - y} \right) và tìm điều kiện xác định.
Đặt f\left( x \right) = {\log _{2020}}\left( {x + {y^2}} \right) + {\log _{2021}}\left( {{y^2} + y + 64} \right) - {\log _4}\left( {x - y} \right) (coi y là tham số).
Điều kiện xác định của f\left( x \right) là:
\left\{ {\begin{array}{*{20}{c}}{x + {y^2} > 0}\\{{y^2} + y + 64 > 0}\\{x - y > 0}\end{array}} \right.
Do x,\;y nguyên nên x > y \ge - {y^2}. Cũng vì x,\;y nguyên nên ta chỉ xét f\left( x \right) trên nửa khoảng \left[ {y + 1; + \infty } \right).
Bước 2: Xét hàm số trên \left[ {y + 1; + \infty } \right)
Ta có:
f'\left( x \right) = \dfrac{1}{{\left( {x + {y^2}} \right)\ln 2020}} - \dfrac{1}{{\left( {x - y} \right)\ln 2021}} - \dfrac{1}{{\left( {x - y} \right)\ln 4}} < 0,\;\forall x \ge y + 1
Bước 3: Lập bảng biến thiên
Ta có bảng biến thiên của hàm số f\left( x \right):
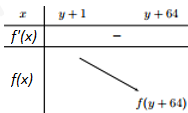
Bước 4: Tìm y nguyên f\left( {y + 64} \right) < 0
Yêu cầu bài toán trở thành:
f\left( {y + 64} \right) < 0
\Leftrightarrow {\log _{2020}}\left( {{y^2} + y + 64} \right) + {\log _{2021}}\left( {{y^2} + y + 64} \right) < {\log _4}64
\Leftrightarrow {\log _{2021}}\left( {{y^2} + y + 64} \right)\left( {{{\log }_{2020}}2021 + 1} \right) < 3
\Leftrightarrow {y^2} + y + 64 - {2021^{\dfrac{3}{{{{\log }_{2020}}2021 + 1}}}} < 0
\Leftrightarrow - 301,76 < y < 300,76
Mà y nguyên nên y \in \left\{ { - 301; - 300; \ldots ;299;300} \right\}.
Vậy có 602 giá trị nguyên của y thỏa mãn yêu cầu.

