Tính đạo hàm của hàm số \(y = {6^x}\).
\(y = {6^x} \Rightarrow \)\(y' = {6^x}\ln 6.\)
Cho hàm số \(y = {e^{2x}} - x\). Chọn khẳng định đúng.
TXĐ: \(D = \mathbb{R}\).
Ta có: \(y' = 2{e^{2x}} - 1 = 0 \Leftrightarrow {e^{2x}} = \dfrac{1}{2}\) \( \Leftrightarrow 2x = \ln \dfrac{1}{2} = - \ln 2 \Leftrightarrow x = - \dfrac{1}{2}\ln 2 = - \ln \sqrt 2 \).
BBT:
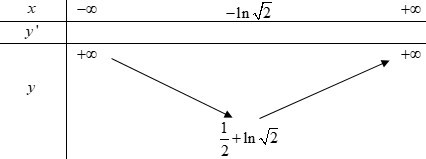
Dựa vào BBT ta thấy hàm số đồng biến trên \(\left( { - \ln \sqrt 2 ; + \infty } \right)\).
Tìm tất cả các giá trị thực của \(m\) để hàm số \(y = {2^{{x^3} - {x^2} + m\,x + 1}}\) đồng biến trên \(\left( {1;2} \right)\)
Ta có: \(y = {2^{{x^3} - {x^2} + mx + 1}}\) \( \Rightarrow y' = \left( {3{x^2} - 2x + m} \right){2^{{x^3} - {x^2} + mx + 1}}\)
\( \Rightarrow \) Hàm số đã cho đồng biến trên \(\left( {1;\,\,2} \right) \Leftrightarrow y' \ge 0\,\,\forall x \in \left( {1;\,\,2} \right)\)
\(\begin{array}{l} \Leftrightarrow \left( {3{x^2} - 2x + m} \right){2^{{x^3} - {x^2} + mx + 1}} \ge 0\,\,\,\forall x \in \left( {1;\,\,2} \right)\\ \Leftrightarrow 3{x^2} - 2x + m \ge \,0\,\,\,\forall x \in \left( {1;\,\,2} \right)\\ \Leftrightarrow \left[ \begin{array}{l}\Delta ' \le 0\\\left\{ \begin{array}{l}\Delta ' \ge 0\\\left[ \begin{array}{l}{x_1} < {x_2} \le 1\\2 \le {x_1} < {x_2}\end{array} \right.\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}\Delta ' \le 0\\\left\{ \begin{array}{l}\Delta ' \ge 0\\\left[ \begin{array}{l}\left\{ \begin{array}{l}{x_1} + {x_2} < 2\\\left( {{x_1} - 1} \right)\left( {{x_2} - 1} \right) \ge 0\end{array} \right.\\\left\{ \begin{array}{l}{x_1} + {x_2} > 4\\\left( {{x_1} - 2} \right)\left( {{x_2} - 2} \right) \ge 0\end{array} \right.\end{array} \right.\end{array} \right.\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}\Delta ' \le 0\\\left\{ \begin{array}{l}\Delta ' \ge 0\\\left[ \begin{array}{l}\left\{ \begin{array}{l}{x_1} + {x_2} < 2\\{x_1}{x_2} - \left( {{x_1} + {x_2}} \right) + 1 \ge 0\end{array} \right.\\\left\{ \begin{array}{l}{x_1} + {x_2} > 4\\{x_1}{x_2} - 2\left( {{x_1} + {x_2}} \right) + 4 \ge 0\end{array} \right.\end{array} \right.\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}1 - 3m \le 0\\\left\{ \begin{array}{l}1 - 3m \ge 0\\\left[ \begin{array}{l}\frac{2}{3} < 2\\\frac{m}{3} - \frac{2}{3} + 1 \ge 0\end{array} \right.\\\left[ \begin{array}{l}\frac{x}{3} > 4\,\,\,\left( {ktm} \right)\\\frac{m}{3} - \frac{4}{3} + 4 \ge 0\end{array} \right.\end{array} \right.\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}m \ge \frac{1}{3}\\\left\{ \begin{array}{l}m \le \frac{1}{3}\\\frac{m}{3} \ge - \frac{1}{3}\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}m \ge \frac{1}{3}\\\left\{ \begin{array}{l}m \le \frac{1}{3}\\m \ge - 1\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}m \ge \frac{1}{3}\\ - 1 \le m \le \frac{1}{3}\end{array} \right. \Leftrightarrow m \ge - 1.\end{array}\)
Cho hàm số \(f\left( x \right) = \dfrac{1}{{3 + {2^x}}} + \dfrac{1}{{3 + {2^{ - x}}}}\). Trong các khẳng định, có bao nhiêu khẳng định đúng?
1) \(f'\left( x \right) \ne 0,\forall x \in R\)
2) \(f\left( 1 \right) + f\left( 2 \right) + ... + f\left( {2017} \right) = 2017\)
3) \(f\left( {{x^2}} \right) = \dfrac{1}{{3 + {4^x}}} + \dfrac{1}{{3 + {4^{ - x}}}}\)
Ta có:
\(f'\left( x \right) = \dfrac{{ - {2^x}\ln 2}}{{{{\left( {3 + {2^x}} \right)}^2}}} + \dfrac{{{2^{ - x}}\ln 2}}{{{{\left( {3 + {2^{ - x}}} \right)}^2}}} \Rightarrow f'\left( 0 \right) = 0\) nên khẳng định (1) sai.
\(f\left( x \right) = \dfrac{{{2^x} + {2^{ - x}} + 6}}{{\left( {3 + {2^x}} \right)\left( {3 + {2^{ - x}}} \right)}} = \dfrac{{{2^x} + {2^{ - x}} + 6}}{{3\left( {{2^x} + {2^{ - x}}} \right) + 10}}\)
Đặt \(t = {2^x} + {2^{ - x}} \ge 2\sqrt {{2^x}{{.2}^{ - x}}} = 2\) thì \(\dfrac{{{2^x} + {2^{ - x}} + 6}}{{3\left( {{3^x} + {2^{ - x}}} \right) + 10}} = \dfrac{{t + 6}}{{3t + 10}}\)
Xét \(g\left( t \right) = \dfrac{{t + 6}}{{3t + 10}},g'\left( t \right) = - \dfrac{8}{{{{\left( {3t + 10} \right)}^2}}} < 0\) nên hàm số nghịch biến trên \(\left[ {2; + \infty } \right)\).
\( \Rightarrow g\left( t \right) \le g\left( 2 \right) = \dfrac{{2 + 6}}{{3.2 + 10}} = \dfrac{1}{2} < 1\) hay \(f\left( x \right) < 1,\forall x\).
Suy ra \(f\left( 1 \right) < 1,f\left( 2 \right) < 1,...,f\left( {2017} \right) < 1\).
\( \Rightarrow f\left( 1 \right) + f\left( 2 \right) + ... + f\left( {2017} \right) < 2017\) nên (2) sai.
\(f\left( {{x^2}} \right) = \dfrac{1}{{3 + {2^{{x^2}}}}} + \dfrac{1}{{3 + {2^{ - {x^2}}}}} \ne \dfrac{1}{{3 + {4^x}}} + \dfrac{1}{{3 + {4^{ - x}}}}\) (chẳng hạn \(x = 1\)) nên (3) sai.
Do đó không có khẳng định nào đúng.
Cho hàm số \(f(x) = {(3 - \sqrt 2 )^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}}\) . Xét các khẳng định sau:
Khẳng định 1: \(f(x) > 0 \Leftrightarrow {x^3} + {x^2} > 0\)
Khẳng định 2: \(f(x) > 0 \Leftrightarrow x > - 1\).
Khẳng định 3: \(f(x) < 3 - \sqrt 2 \Leftrightarrow {(3 - \sqrt 2 )^{{x^3} - 1}} < 1 + {\left( {\dfrac{{3 + \sqrt 2 }}{7}} \right)^{{x^2} + 1}}\)
Khẳng định 4:\(f(x) < 3 + \sqrt 2 \Leftrightarrow {(3 - \sqrt 2 )^{{x^3} + 1}} < {(3 - \sqrt 2 )^{1 - {x^2}}} + 7\)
Trong các khẳng định trên, có bao nhiêu khẳng định đúng?
Cơ số $3 - \sqrt 2 > 1$
Ta có $f\left( x \right) > 0 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} > 0 \Leftrightarrow {x^3} > - {x^2} \Leftrightarrow {x^3} + {x^2} > 0$ suy ra khẳng định 1 đúng.
Ta có $f\left( x \right) > 0 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} > 0 \Leftrightarrow {x^3} > - {x^2} \Leftrightarrow {x^3} + {x^2} > 0 $
$\Leftrightarrow {x^2}\left( {x + 1} \right) > 0 \Leftrightarrow \left\{ \begin{gathered}
x > - 1 \hfill \\
x \ne 0 \hfill \\
\end{gathered} \right.$ suy ra khẳng định 2 sai.
Ta có
$\begin{array}{l}f\left( x \right) < 3 - \sqrt 2 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} < 3 - \sqrt 2 \\ \Leftrightarrow \dfrac{{{{\left( {3 - \sqrt 2 } \right)}^{{x^3}}}}}{{3 - \sqrt 2 }} - \dfrac{{{{\left( {3 - \sqrt 2 } \right)}^{ - {x^2}}}}}{{3 - \sqrt 2 }} < 1 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} - 1}} < 1 + {\left( {3 - \sqrt 2 } \right)^{ - {x^2} - 1}} \\ \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} - 1}} < 1 + {\left( {\dfrac{1}{{3 - \sqrt 2 }}} \right)^{{x^2} + 1}} \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} - 1}} < 1 + {\left( {\dfrac{{3 + \sqrt 2 }}{7}} \right)^{{x^2} + 1}}\end{array}$
suy ra khẳng định 3 đúng.
Ta có
$\begin{array}{l}f\left( x \right) < 3 + \sqrt 2 \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}} - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}} < 3 + \sqrt 2 \\ \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3}}}\left( {3 - \sqrt 2 } \right) - {\left( {3 - \sqrt 2 } \right)^{ - {x^2}}}\left( {3 - \sqrt 2 } \right) < \left( {3 + \sqrt 2 } \right)\left( {3 - \sqrt 2 } \right)\\ \Leftrightarrow {\left( {3 - \sqrt 2 } \right)^{{x^3} + 1}} < {\left( {3 - \sqrt 2 } \right)^{1 - {x^2}}} + 7\end{array}$
Suy ra khẳng định 4 đúng.
Vậy có $3$ khẳng định đúng.
Cho hai số thực dương $x, y$ thỏa mãn \({2^x} + {2^y} = 4\). Tìm giá trị lớn nhất \({P_{\max }}\) của biểu thức\(P = (2{x^2} + y)(2{y^2} + x) + 9xy\).
Ta có:
\(\begin{array}{l}4 = {2^x} + {2^y} \ge 2\sqrt {{2^x}{{.2}^y}} \Rightarrow 2 \ge \sqrt {{2^x}{2^y}} \\ \Rightarrow 4 \ge {2^{x + y}} \Rightarrow 0 < x + y \le 2\\ \Rightarrow {\left( {x + y} \right)^2} \le 4\end{array}\)
Lại có \(x + y \ge 2\sqrt {xy} \Rightarrow xy \le 1\)
\(\begin{array}{l} \Rightarrow P = 4{x^2}{y^2} + 2{x^3} + 2{y^3} + 10xy\\ = 4{\left( {xy} \right)^2} + 10xy + 2\left( {{x^3} + {y^3}} \right)\\ = 4{\left( {xy} \right)^2} + 10xy \\+ 2.\left( {x + y} \right).\left[ {{{\left( {x + y} \right)}^2} - 3xy} \right]\\ \Rightarrow P \le 4{\left( {xy} \right)^2} + 10xy + 2.2.\left( {4 - 3xy} \right)\\ \Rightarrow P \le 4{\left( {xy} \right)^2} - 2xy + 16\end{array}\)
Đặt \(xy = t \Rightarrow 0 < t \le 1\)
Xét hàm số \(f\left( t \right) = 4{t^2} - 2t + 16\) trên \(\left( {0;1} \right]\).
\( \Rightarrow f\left( t \right) \le \max \left\{ {f\left( 1 \right),f\left( 0 \right)} \right\} = 18\).
Dấu “=” xảy ra khi và chỉ khi \(x = y = 1\).
Vậy \({P_{\max }} = 18 \Leftrightarrow x = y = 1\).
Gọi $I(t)$ là số ca bị nhiễm bệnh Covid-19 ở quốc gia $\mathrm{X}$ sau $t$ ngày khảo sát. Khi đó ta có công thức $I(t)=A . e^{r_{0}(t-1)}$ với $A$ là số ca bị nhiễm trong ngày khảo sát đầu tiên, $r_{0}$ là hệ số lây nhiễm. Biết rằng ngày đầu tiên khảo sát có 500 ca bị nhiễm bệnh và ngày thứ 10 khảo sát có 1000 ca bị nhiễm bệnh. Hỏi ngày thứ 20 số ca nhiễm bệnh gần nhất với số nào dưới đây, biết rằng trong suốt quá trình khảo sát hệ số lây nhiễm là không đổi?
2160
2160
2160
Bước 1: Tính $r_0$
Theo giả thiết ta có: I(1)=A=500
Ngày thứ 10 có 1000 ca nhiễm nên
\(\begin{array}{l}I\left( {10} \right) = A.{e^{9{r_0}}} \Leftrightarrow 1000 = 500.{e^{9{r_0}}}\\ \Leftrightarrow {e^{9{r_0}}} = 2 \Leftrightarrow 9{r_0} = \ln 2\\ \Leftrightarrow {r_0} = \dfrac{{\ln 2}}{9}\end{array}\)
Bước 2: Tính I(20)
Áp dụng công thức thì ta được ngày thứ 20 số ca nhiễm bệnh là:
\(I\left( {20} \right) = 500.{e^{\frac{{19\ln 2}}{9}}} \approx 2160\)

