Xét sự biến thiên của hàm số \(y = \sqrt {4x + 5} + \sqrt {x - 1} \) trên tập xác định của nó. Áp dụng tìm số nghiệm của phương trình \(\sqrt {4x + 5} + \sqrt {x - 1} = 3\)
ĐKXĐ: \(\left\{ {\begin{array}{*{20}{c}}{4x + 5 \ge 0}\\{x - 1 \ge 0}\end{array}} \right. \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{x \ge - \dfrac{5}{4}}\\{x \ge 1}\end{array}} \right. \Leftrightarrow x \ge 1\)
Suy ra TXĐ: ${\rm{D}} = \left[ {1; + \infty } \right)$
Với mọi ${x_1},\,{x_2} \in \left[ {1; + \infty } \right),\,\,{x_1} \ne {x_2}$ ta có
\(\begin{array}{l}f\left( {{x_2}} \right) - f\left( {{x_1}} \right) = \sqrt {4{x_2} + 5} + \sqrt {{x_2} - 1} - \sqrt {4{x_1} + 5} - \sqrt {{x_1} - 1} \\ = \dfrac{{4\left( {{x_2} - {x_1}} \right)}}{{\sqrt {4{x_2} + 5} + \sqrt {4{x_1} + 5} }} + \dfrac{{{x_2} - {x_1}}}{{\sqrt {{x_2} - 1} + \sqrt {{x_1} - 1} }}\\ = \left( {{x_2} - {x_1}} \right)\left( {\dfrac{4}{{\sqrt {4{x_2} + 5} + \sqrt {4{x_1} + 5} }} + \dfrac{1}{{\sqrt {{x_2} - 1} + \sqrt {{x_1} - 1} }}} \right)\end{array}\)
Suy ra \(\dfrac{{f\left( {{x_2}} \right) - f\left( {{x_1}} \right)}}{{{x_2} - {x_1}}} = \dfrac{4}{{\sqrt {4{x_2} + 5} + \sqrt {4{x_1} + 5} }} + \dfrac{1}{{\sqrt {{x_2} - 1} + \sqrt {{x_1} - 1} }} > 0\)
Nên hàm số \(y = \sqrt {4x + 5} + \sqrt {x - 1} \) đồng biến trên khoảng $\left[ {1; + \infty } \right)$.
Vì hàm số đã cho đồng biến trên $\left[ {1; + \infty } \right)$ nên
Nếu \(x > 1 \Rightarrow f\left( x \right) > f\left( 1 \right)\) hay \(\sqrt {4x + 5} + \sqrt {x - 1} > 3\)
Suy ra phương trình \(\sqrt {4x + 5} + \sqrt {x - 1} = 3\) vô nghiệm
Nếu $x < 1 \Rightarrow f\left( x \right) < f\left( 1 \right)$ hay \(\sqrt {4x + 5} + \sqrt {x - 1} < 3\)
Suy ra phương trình \(\sqrt {4x + 5} + \sqrt {x - 1} = 3\) vô nghiệm
Với \(x = 1\) dễ thấy nó là nghiệm của phương trình đã cho
Vậy phương trình có nghiệm duy nhất $x = 1$.
Xét sự biến thiên của hàm số \(y = \sqrt {4x + 5} + \sqrt {x - 1} \) trên tập xác định của nó. Áp dụng tìm số nghiệm của phương trình \(\sqrt {4x + 5} + \sqrt {x - 1} = \sqrt {4{x^2} + 9} + x\)
ĐKXĐ: \(\left\{ {\begin{array}{*{20}{c}}{4x + 5 \ge 0}\\{x - 1 \ge 0}\end{array}} \right. \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{x \ge - \dfrac{5}{4}}\\{x \ge 1}\end{array}} \right. \Leftrightarrow x \ge 1\)
Suy ra TXĐ: ${\rm{D}} = \left[ {1; + \infty } \right)$
Với mọi ${x_1},\,{x_2} \in \left[ {1; + \infty } \right),\,\,{x_1} \ne {x_2}$ ta có
\(\begin{array}{l}f\left( {{x_2}} \right) - f\left( {{x_1}} \right) = \sqrt {4{x_2} + 5} + \sqrt {{x_2} - 1} - \sqrt {4{x_1} + 5} - \sqrt {{x_1} - 1} \\ = \dfrac{{4\left( {{x_2} - {x_1}} \right)}}{{\sqrt {4{x_2} + 5} + \sqrt {4{x_1} + 5} }} + \dfrac{{{x_2} - {x_1}}}{{\sqrt {{x_2} - 1} + \sqrt {{x_1} - 1} }}\\ = \left( {{x_2} - {x_1}} \right)\left( {\dfrac{4}{{\sqrt {4{x_2} + 5} + \sqrt {4{x_1} + 5} }} + \dfrac{1}{{\sqrt {{x_2} - 1} + \sqrt {{x_1} - 1} }}} \right)\end{array}\)
Suy ra \(\dfrac{{f\left( {{x_2}} \right) - f\left( {{x_1}} \right)}}{{{x_2} - {x_1}}} = \dfrac{4}{{\sqrt {4{x_2} + 5} + \sqrt {4{x_1} + 5} }} + \dfrac{1}{{\sqrt {{x_2} - 1} + \sqrt {{x_1} - 1} }} > 0\)
Nên hàm số \(y = \sqrt {4x + 5} + \sqrt {x - 1} \) đồng biến trên khoảng $\left[ {1; + \infty } \right)$.
Xét phương trình đã cho:
Đặt ${x^2} + 1 = t,\,\,t \ge 1 \Rightarrow {x^2} = t - 1$ phương trình trở thành
\(\sqrt {4x + 5} + \sqrt {x - 1} = \sqrt {4t + 5} + \sqrt {t - 1} \Leftrightarrow f\left( x \right) = f\left( t \right)\)
Nếu \(x > t \Rightarrow f\left( x \right) > f\left( t \right)\) hay \(\sqrt {4x + 5} + \sqrt {x - 1} > \sqrt {4t + 5} + \sqrt {t - 1} \)
Suy ra phương trình đã cho vô nghiệm
Nếu $x < t \Rightarrow f\left( x \right) < f\left( t \right)$ hay \(\sqrt {4x + 5} + \sqrt {x - 1} < \sqrt {4t + 5} + \sqrt {t - 1} \)
Suy ra phương trình đã cho vô nghiệm
Vậy \(f\left( x \right) = f\left( t \right) \Leftrightarrow x = t\) hay ${x^2} + 1 = x \Leftrightarrow {x^2} - x + 1 = 0$ (vô nghiệm)
Vậy phương trình đã cho vô nghiệm.
Tìm trên đồ thị hàm số \(y = - {x^3} + {x^2} + 3x - 4\) hai điểm đối xứng nhau qua gốc tọa độ.
Gọi \(M,N\) đối xứng nhau qua gốc tọa độ \(O\). \(M\left( {{x_0};{y_0}} \right) \Rightarrow N\left( { - {x_0}; - {y_0}} \right)\)
Vì $M,{\rm{ }}N$ thuộc đồ thị hàm số nên \(\left\{ {\begin{array}{*{20}{c}}{{y_0} = - x_0^3 + x_0^2 + 3{x_0} - 4}\\{ - {y_0} = x_0^3 + x_0^2 - 3{x_0} - 4}\end{array}} \right.\)
\( \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{{y_0} = - x_0^3 + x_0^2 + 3{x_0} - 4}\\{2x_0^2 - 8 = 0}\end{array}} \right. \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{{y_0} = - x_0^3 + x_0^2 + 3{x_0} - 4}\\{{x_0} = \pm 2}\end{array}} \right.\)
\( \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{{x_0} = 2}\\{{y_0} = - 2}\end{array}} \right.\) hoặc \(\left\{ {\begin{array}{*{20}{c}}{{x_0} = - 2}\\{{y_0} = 2}\end{array}} \right.\)
Vậy hai điểm cần tìm có tọa độ là \(\left( {2; - 2} \right)\) và \(\left( { - 2;2} \right)\).
Xác định parabol \(\left( P \right)\): \(y = a{x^2} + bx + c\), \(a \ne 0\) đỉnh \(I\) biết \(\left( P \right)\) đi qua \(M(4;3)\) cắt \(Ox\) tại \(N(3;0)\) và \(P\) sao cho \(\Delta INP\) có diện tích bằng $1$, biết hoành độ điểm \(P\) nhỏ hơn \(3\).
Vì \(\left( P \right)\) đi qua \(M(4;3)\) nên \(3 = 16a + 4b + c\) (1)
Mặt khác \(\left( P \right)\) cắt \(Ox\) tại \(N(3;0)\) suy ra \(0 = 9a + 3b + c\) (2), \(\left( P \right)\) cắt \(Ox\) tại \(P\) nên \(P\left( {t;0} \right),\,\,t < 3\)
Theo định lý Viét ta có \(\left\{ {\begin{array}{*{20}{c}}{t + 3 = - \dfrac{b}{a}}\\{3t = \dfrac{c}{a}}\end{array}} \right.\)
Ta có \({S_{\Delta IPN}} = \dfrac{1}{2}IH.NP\) với \(H\) là hình chiếu của \(I\left( { - \dfrac{b}{{2a}}; - \dfrac{\Delta }{{4a}}} \right)\) lên $PN$ hay trục hoành
Do \(IH = \left| { - \dfrac{\Delta }{{4a}}} \right|\), \(NP = 3 - t\) nên \({S_{\Delta INP}} = 1 \Leftrightarrow \dfrac{1}{2}\left| { - \dfrac{\Delta }{{4a}}} \right|.\left( {3 - t} \right) = 1\)
\( \Leftrightarrow \left( {3 - t} \right)\left| {{{\left( {\dfrac{b}{{2a}}} \right)}^2} - \dfrac{c}{a}} \right| = \left| {\dfrac{2}{a}} \right| \Leftrightarrow \left( {3 - t} \right)\left| {{{\dfrac{{\left( {t + 3} \right)}}{4}}^2} - 3t} \right| = \left| {\dfrac{2}{a}} \right| \Leftrightarrow {\left( {3 - t} \right)^3} = \dfrac{8}{{\left| a \right|}}\) (3)
Từ (1) và (2) ta có \(7a + b = 3 \Leftrightarrow b = 3 - 7a\) suy ra \(t + 3 = - \dfrac{{3 - 7a}}{a} \Leftrightarrow \dfrac{1}{a} = \dfrac{{4 - t}}{3}>0\) do $t<3$
Thay vào (3) ta có \({\left( {3 - t} \right)^3} = \dfrac{{8\left( {4 - t} \right)}}{3} \Leftrightarrow 3{t^3} - 27{t^2} + 73t - 49 = 0 \Leftrightarrow t = 1\)
Suy ra $a = 1 \Rightarrow b = - 4 \Rightarrow c = 3$.
Vậy \(\left( P \right)\) cần tìm là $y = {x^2} - 4x + 3$.
Tìm giá trị nhỏ nhất của hàm số: \(y = \sqrt[3]{{{x^4} + 2{x^2} + 1}} - 3\sqrt[3]{{{x^2} + 1}} + 1\)
Đặt \(t = \sqrt[3]{{{x^2} + 1}},\,\,t \ge 1 \Rightarrow {t^2} = \sqrt[3]{{{x^4} + 2{x^2} + 1}}\)
Khi đó hàm số trở thành \(y = {t^2} - 3t + 1\) với \(t \ge 1\).
Bảng biến thiên
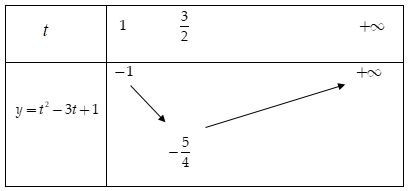
Suy ra giá trị nhỏ nhất của hàm số \(y = \sqrt[3]{{{x^4} + 2{x^2} + 1}} - 3\sqrt[3]{{{x^2} + 1}} + 1\) là \( - \dfrac{5}{4}\) khi và chỉ khi $t = \dfrac{3}{2}$ hay \(\sqrt[3]{{{x^2} + 1}} = \dfrac{3}{2} \Leftrightarrow x = \pm \sqrt {\dfrac{{19}}{8}} \)
