Hỏi có bao nhiêu giá trị nguyên $x$ thỏa mãn bất phương trình $\left| {\dfrac{{2 - x}}{{x + 1}}} \right| \ge 2$ ?

Điều kiện: $x + 1 \ne 0 \Leftrightarrow x \ne  - \,1.$

Bất phương trình $\left| {\dfrac{{2 - x}}{{x + 1}}} \right| \ge 2 \Leftrightarrow \left[ \begin{array}{l}\dfrac{{2 - x}}{{x + 1}} \ge 2\\\dfrac{{2 - x}}{{x + 1}} \le  - 2\end{array} \right.$$ \Leftrightarrow \left[ \begin{array}{l}\dfrac{{2 - x}}{{x + 1}} - 2 \ge 0\\\dfrac{{2 - x}}{{x + 1}} + 2 \le 0\end{array} \right.$

\( \Leftrightarrow \left[ \begin{array}{l}
\dfrac{{2 - x - 2x - 2}}{{x + 1}} \ge 0\\
\dfrac{{2 - x + 2x + 2}}{{x + 1}} \le 0
\end{array} \right. \) \(\Leftrightarrow \left[ \begin{array}{l}
\dfrac{{ - 3x}}{{x + 1}} \ge 0\\
\dfrac{{x + 4}}{{x + 1}} \le 0
\end{array} \right. \) \(\Leftrightarrow \left[ \begin{array}{l}
\dfrac{x}{{x + 1}} \le 0\,\,\left( 1 \right)\\
\dfrac{{x + 4}}{{x + 1}} \le 0\,\,\left( 2 \right)
\end{array} \right.\)

Giải $\left( 1 \right),$ ta có bảng xét dấu:

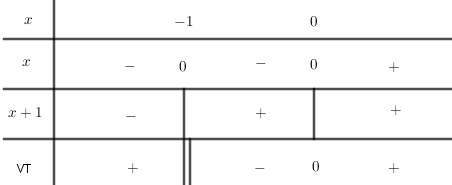
Ta có bất phương trình $\left( 1 \right) \Leftrightarrow \dfrac{x}{{x + 1}} \le 0 \Leftrightarrow  - \,1 < x \le 0.$

Giải $\left( 2 \right),$ ta có bảng xét dấu:

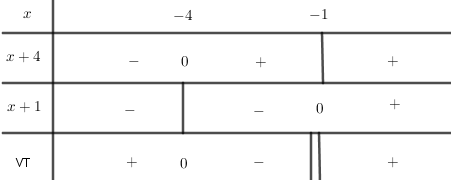
Ta có bất phương trình $\left( 2 \right) \Leftrightarrow  - \,4 \le x <  - \,1.$

Do đó, tập nghiệm của bất phương trình là $S = \left[ { - \,4; - \,1} \right) \cup \left( { - \,1;0} \right].$

Vậy có tất cả $4$ giá trị nguyên $x$ cần tìm là $x = \left\{ { - \,4; - \,3; - \,2;0} \right\}.$