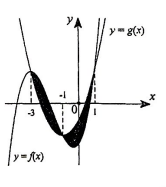
Cho hai hàm số \(f\left( x \right) = m{x^3} + n{x^2} + px - \dfrac{5}{2}\)\(\left( {m,n,p \in \mathbb{R}} \right)\) và \(g\left( x \right) = {x^2} + 2x - 1\) có đồ thị cắt nhau tại ba điểm có hoành độ lần lượt là \( - 3;\,\, - 1;\,\,1\)( tham khảo hình vẽ bên). Diện tích hình phẳng giới hạn bởi hai đồ thị hàm số \(f\left( x \right)\)và \(g\left( x \right)\) bằng

Đồ thị hàm số \(f\left( x \right) = m{x^3} + n{x^2} + px - \dfrac{5}{2}\) đi qua các điểm có tọa độ \(\left( {1;2} \right);\) \(\left( { - 1; - 2} \right);\)\(\left( { - 3;2} \right)\) nên ta có hệ phương trình: \(\left\{ \begin{array}{l}m + n + p - \dfrac{5}{2} = 2\\ - m + n - p - \dfrac{5}{2} =  - 2\\ - 27m + 9n - 3p - \dfrac{5}{2} = 2\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}m = \dfrac{1}{2}\\n = \dfrac{5}{2}\\p = \dfrac{3}{2}\end{array} \right.\).

\( \Rightarrow f\left( x \right) = \dfrac{1}{2}{x^3} + \dfrac{5}{2}{x^2} + \dfrac{3}{2}x - \dfrac{5}{2}.\)

Xét phương trình hoành độ giao điểm \(f\left( x \right) = g\left( x \right) \Leftrightarrow f\left( x \right) - g\left( x \right) = 0\).

Dựa vào đồ thị ta thấy phương trình \(f\left( x \right) - g\left( x \right) = 0\) có 3 nghiệm là \(\left[ \begin{array}{l}{x_1} =  - 3\\{x_2} =  - 1\\{x_3} = 1\end{array} \right.\)

Do đó diện tích hình phẳng giới hạn bởi hai đồ thị hàm số \(f\left( x \right);g\left( x \right)\) bằng

\(\begin{array}{l}S = \int\limits_{ - 3}^{ - 1} {\left[ {f\left( x \right) - g\left( x \right)} \right]dx}  + \int\limits_{ - 1}^1 {\left[ {g\left( x \right) - f\left( x \right)} \right]dx} \\\,\,\,\, = \int\limits_{ - 3}^{ - 1} {\left( {\dfrac{1}{2}{x^3} + \dfrac{5}{2}{x^2} + \dfrac{3}{2}x - \dfrac{5}{2} - {x^2} - 2x + 1} \right)dx}  + \int\limits_{ - 1}^1 {\left( {{x^2} + 2x - 1 - \dfrac{1}{2}{x^3} - \dfrac{5}{2}{x^2} - \dfrac{3}{2}x + \dfrac{5}{2}} \right)dx} \\\,\,\,\, = \int\limits_{ - 3}^{ - 1} {\left( {\dfrac{1}{2}{x^3} + \dfrac{3}{2}{x^2} - \dfrac{1}{2}x - \dfrac{3}{2}} \right)dx}  + \int\limits_{ - 1}^1 {\left( { - \dfrac{1}{2}{x^3} - \dfrac{3}{2}{x^2} + \dfrac{1}{2}x + \dfrac{3}{2}} \right)dx} \\\,\,\,\,\, = 2 + 2 = 4.\end{array}\)