Cho hình chóp S.ABC có đáy ABC là tam giác đều cạnh bằng a. Biết tam giác SBA vuông tại B, tam giác SCA vuông tại C và khoảng cách giữa hai đường thẳng AC và SB bằng 3a√13. Tính thể tích khối chóp S.ABC.
Trả lời bởi giáo viên
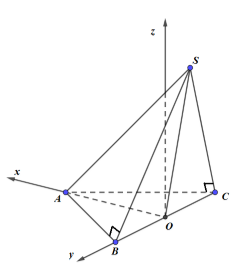
Gọi O là trung điểm của BC.
Ta gắn hệ trục tọa độ Oxyz như hình vẽ. Trong đó:
A(a√32;0;0),B(0;a2;0),C(0;−a2;0)
Gọi (P) là mặt phẳng vuông góc với AB tại B, (Q) là mặt phẳng vuông góc với AC tại C. Gọi giao tuyến của (P) và (Q) là đường thẳng d.
Do SB⊥AB,SC⊥AC nên S∈d.
→AB=(−a√32;a2;0),→AC=(−a√32;−a2;0)
Mặt phẳng (P) đi qua B(0;a2;0), nhận →n1=(√3;−1;0) là 1 VTPT, có phương trình là: √3x−y+a2=0.
Mặt phẳng (Q) đi qua C(0;−a2;0), nhận →n2=(√3;1;0) là 1 VTPT, có phương trình là: √3x+y+a2=0.
d là giao của (P) và (Q)⇒d:{√3x−y+a2=0√3x+y+a2=0, [→n1;→n2]=(0;0;2√3)
⇒d đi qua I(−a2√3;0;0)có 1 VTCP →u=(0;0;1), có phương trình tham số là: {x=−a2√3y=0z=t
Giả sử S(−a2√3;0;t). Ta có: →SB=(a2√3;a2;−t);→CA=(a√32;a2;0)⇒[→SB;→CA]=(at2;a√3t2;−a2√36)⇒|[→SB;→CA]|=√a2t24+3a2t24+a212=√a2t2+a212
Ta có: →CB=(0;a;0)⇒[→SB;→CA].→CB=0+a√3t2.a+0=a2√3t2
d(SB;AC)=|[→SB;→CA].→CB||[→SB;→CA]|=|a2√3t2|√a2t2+a212
⇒|a2√3t2|√a2t2+a212=3a√13⇔3a4t24a2t2+13a2=9a213⇔39a2t2=36a2t2+3a2⇔t2=a2⇔t=a
⇒S(−a2√3;0;a)⇒h=d(S;(Oxy))=a
Diện tích tam giác đều ABC là: S=a2√34⇒VS.ABC=13.h.S=13.a.a2√34=a3√312.
Hướng dẫn giải:
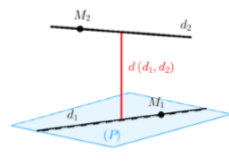
Gắn hệ trục tọa độ.
Đường thẳng d1 có 1 VTCP →u1, đi qua điểm M1.
Đường thẳng d2 có 1 VTCP →u2, đi qua điểm M2.
Khoảng cách giữa d1 và d2 được tính theo công thức:
d(d1;d2)=|→M1M2.[→u1;→u2]||[→u1;→u2]|